Match the following figures Prisms Nets with areas of faces (a) (b) (c) (d)
To solve the problem of matching the figures of prisms with their corresponding nets based on the areas of their faces, we will follow these steps:
1. Identify the Areas of Faces:
- We need to look at the areas of the adjacent faces of each prism and calculate their products.
2. Calculate for Prism A:
- The adjacent faces are given as 4 and 4.
- Calculate the area: 4×4=16.
- Now, we look for a net with an area of 16.
3. Match Prism A:
- From the options, we find that the net with an area of 16 is D.
- Therefore, A matches with D.
4. Calculate for Prism B:
- The adjacent faces are given as 3 and 3.
- Calculate the area: 3×3=9.
- Now, we look for a net with an area of 9.
5. Match Prism B:
- From the options, we find that the net with an area of 9 is A.
- Therefore, B matches with A.
6. Calculate for Prism C:
- The adjacent faces are given as 4 and 3.
- Calculate the area: 4×3=12.
- Now, we look for a net with an area of 12.
7. Match Prism C:
- From the options, we find that the net with an area of 12 is B.
- Therefore, C matches with B.
8. Calculate for Prism D:
- The adjacent faces are given as 3 and 3.
- Calculate the area: 3×3=9.
- Now, we look for a net with an area of 9.
9. Match Prism D:
- From the options, we find that the net with an area of 9 is C.
- Therefore, D matches with C.
Final Matches:
- A → D
- B → A
- C → B
- D → C
- We need to look at the areas of the adjacent faces of each prism and calculate their products.
- The adjacent faces are given as 4 and 4.
- Calculate the area: 4×4=16.
- Now, we look for a net with an area of 16.
- From the options, we find that the net with an area of 16 is D.
- Therefore, A matches with D.
- The adjacent faces are given as 3 and 3.
- Calculate the area: 3×3=9.
- Now, we look for a net with an area of 9.
- From the options, we find that the net with an area of 9 is A.
- Therefore, B matches with A.
- The adjacent faces are given as 4 and 3.
- Calculate the area: 4×3=12.
- Now, we look for a net with an area of 12.
- From the options, we find that the net with an area of 12 is B.
- Therefore, C matches with B.
- The adjacent faces are given as 3 and 3.
- Calculate the area: 3×3=9.
- Now, we look for a net with an area of 9.
- From the options, we find that the net with an area of 9 is C.
- Therefore, D matches with C.
- A → D
- B → A
- C → B
- D → C
Topper's Solved these Questions
Similar Questions
Match the following nets with appropriate solids:
View SolutionMatch the following

(a)
(b)
(c )
(d)
View SolutionIdentify the following figure with A,B,C and D
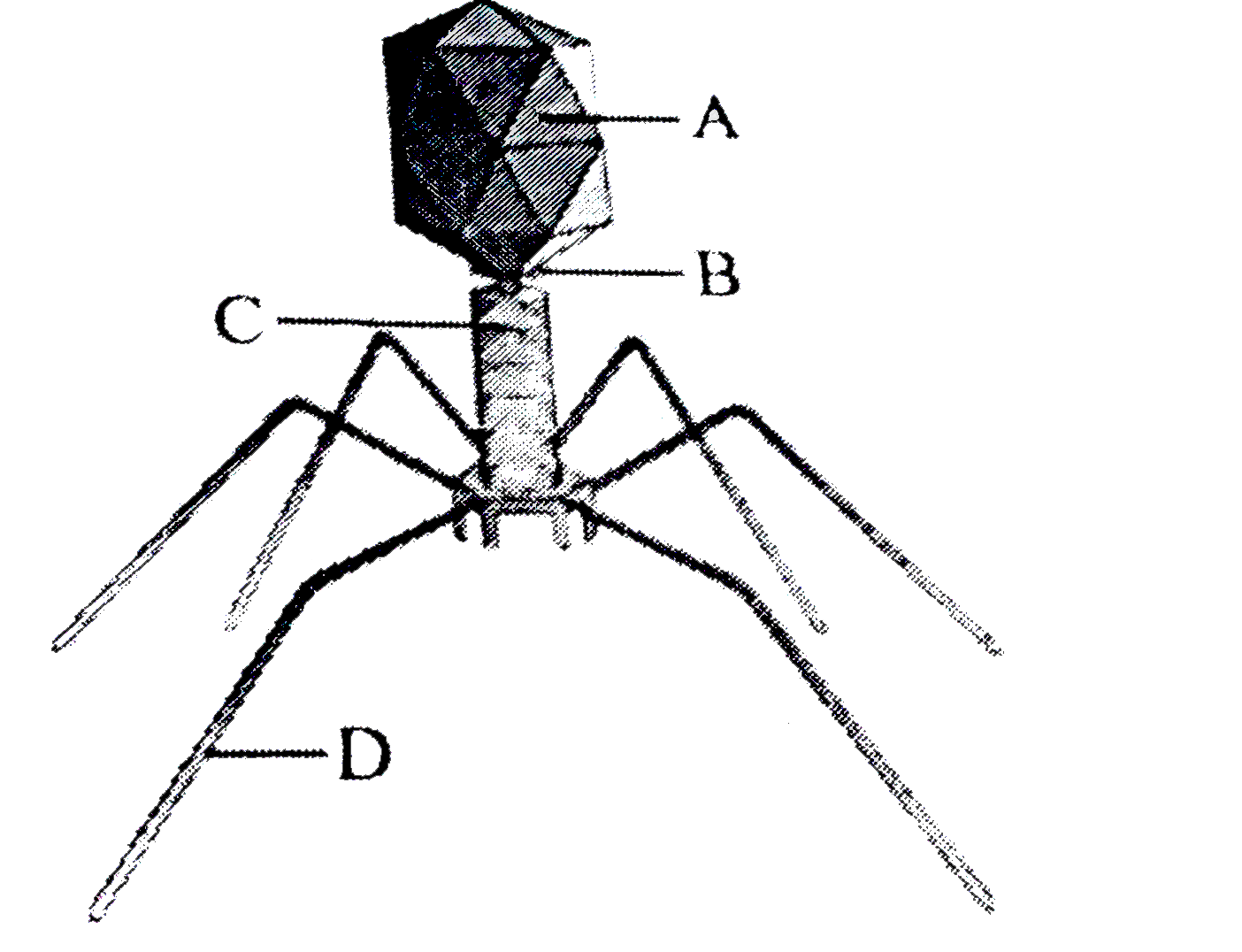
View SolutionIndentify the following figures (A,B,C and D)
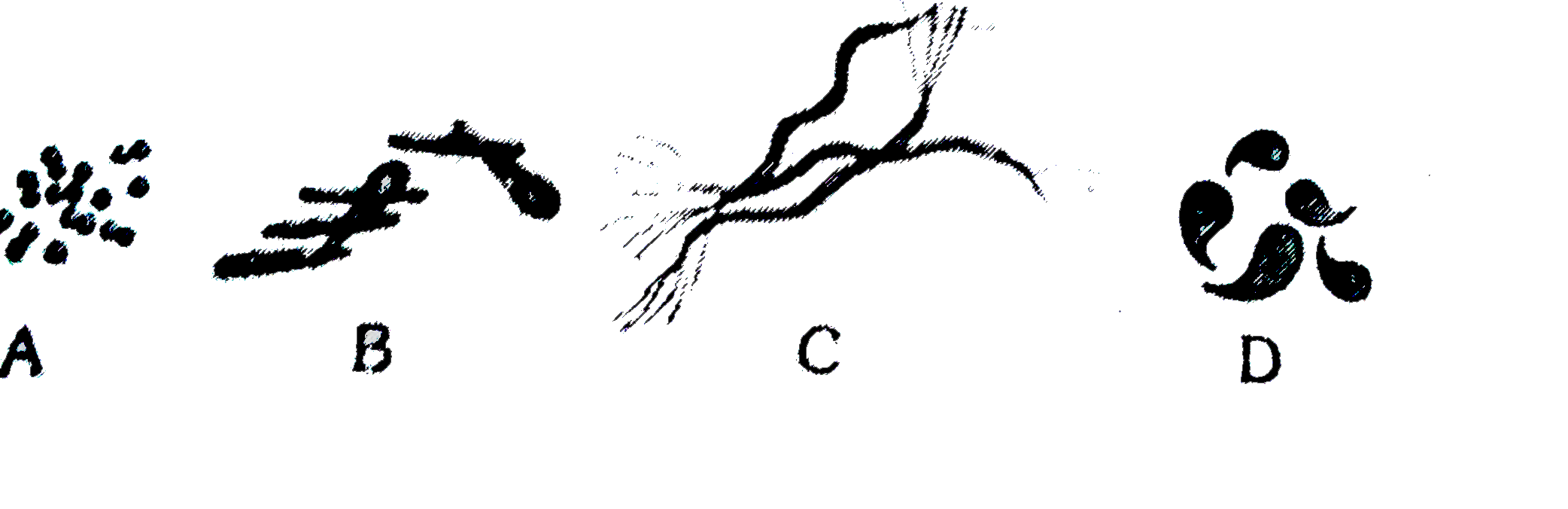 .
.
View SolutionIn a sphere the number of faces is 1 (b) 2 (c) 3 (d) 4
View Solution
Following figure showing pistil identify-A, B, C & D.
View SolutionIn which of the following entropy increases?
View SolutionIn Figure, compute the area of quadrilateral ABCD.
View SolutionMatch the following and choose the correct combination from the options given :

(a) ABCD3154
(b) ABCD1234
(c) ABCD2134
(d) ABCD3152
View SolutionIdentify the following A,B,C and D in given figure:
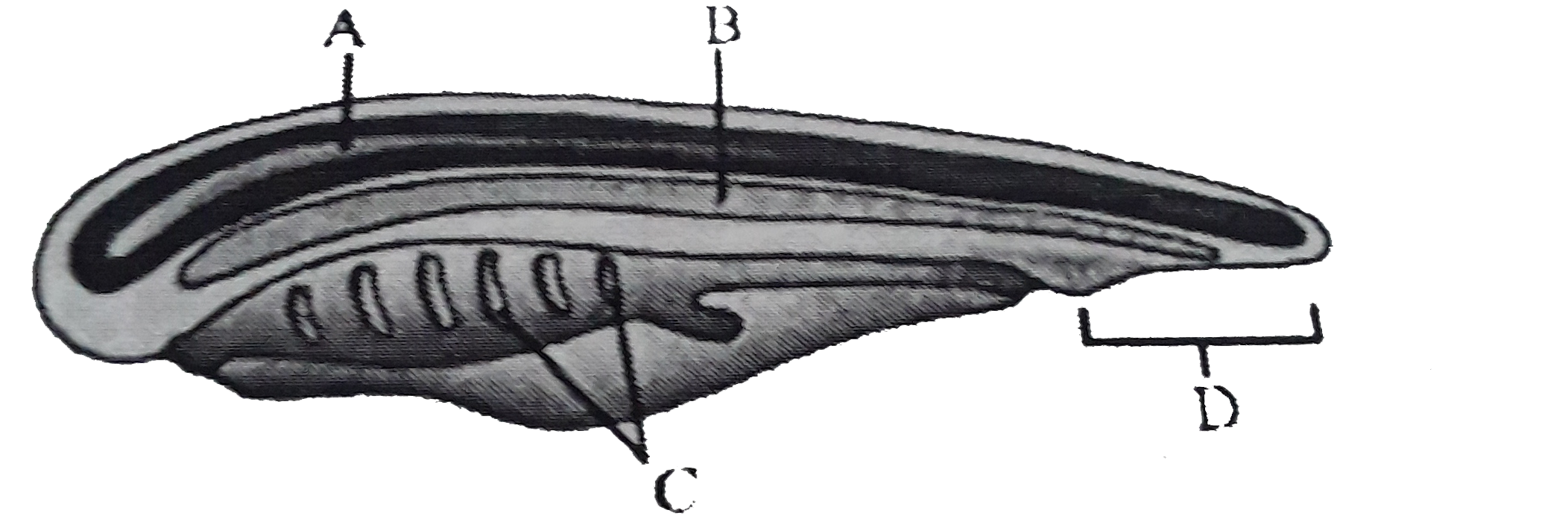
View Solution