0.040g of He is kept in a closed container initially at 100.0∘C.The container is now heated. Neglecting the expansion of the container, Calculate the temperature at which the inernal energy is increased by 12J.
To solve the problem step by step, we will follow the outlined process in the video transcript and provide a detailed explanation for each step.
Step 1: Calculate the number of moles of Helium
Given:
- Mass of Helium (m) = 0.040 g
- Molar mass of Helium (M) = 4 g/mol
Using the formula for the number of moles:
n=mM
Substituting the values:
n=0.040g4g/mol=0.01mol
Step 2: Determine the specific heat at constant volume (Cv)
For a monoatomic gas like Helium, the specific heat at constant volume is given by:
Cv=32R
Where R=8.314J/(mol K). Thus,
Cv=32×8.314=12.471J/(mol K)≈12.45J/(mol K)
Step 3: Use the change in internal energy formula
The change in internal energy (ΔU) at constant volume is given by:
ΔU=nCvΔT
Where:
- ΔU=12J
- n=0.01mol
- Cv=12.45J/(mol K)
Rearranging the formula to find ΔT:
ΔT=ΔUnCv
Substituting the values:
ΔT=12J0.01mol×12.45J/(mol K)=120.1245≈96.39K
Step 4: Calculate the final temperature (T2)
The initial temperature (T1) is given as 100.0∘C, which we need to convert to Kelvin:
T1=100+273=373K
Now, we can find the final temperature (T2):
T2=T1+ΔT=373K+96.39K≈469.39K
Step 5: Convert the final temperature back to Celsius
To convert T2 back to Celsius:
T2=469.39−273≈196.39∘C
Final Answer
The temperature at which the internal energy is increased by 12 J is approximately 196.39∘C.
---
Given:
- Mass of Helium (m) = 0.040 g
- Molar mass of Helium (M) = 4 g/mol
n=mM
Substituting the values:
n=0.040g4g/mol=0.01mol
For a monoatomic gas like Helium, the specific heat at constant volume is given by:
Cv=32R
Where R=8.314J/(mol K). Thus,
Cv=32×8.314=12.471J/(mol K)≈12.45J/(mol K)
The change in internal energy (ΔU) at constant volume is given by:
ΔU=nCvΔT
Where:
- ΔU=12J
- n=0.01mol
- Cv=12.45J/(mol K)
ΔT=ΔUnCv
Substituting the values:
ΔT=12J0.01mol×12.45J/(mol K)=120.1245≈96.39K
The initial temperature (T1) is given as 100.0∘C, which we need to convert to Kelvin:
T1=100+273=373K
Now, we can find the final temperature (T2):
T2=T1+ΔT=373K+96.39K≈469.39K
To convert T2 back to Celsius:
T2=469.39−273≈196.39∘C
The temperature at which the internal energy is increased by 12 J is approximately 196.39∘C.
Topper's Solved these Questions
Similar Questions
An open flask at 25∘C contains air. Calculate the temperature at which one fifth of air measured at 25∘C escapes.
View Solution200 g of hot water at 80∘C is added to 300 g of cold water at 10∘C. Neglecting the heat taken by the container, calculate the final temperature of the mixture of water. Specific heat capacity of water = 4200 J kg−1K−1
View SolutionA bucket contains 8 kg of water at 25∘C. 2 kg of water at 80∘C is poured into it. Neglecting the heat energy absorbed by the bucket, calculate the final temperature of water.
View SolutionA gas is contained in a vessel fitted with a movable piston. The container is placed on a stove. A total of 100 cal of heat is given to the gas and the gas does 40 J of work in the expansion resulting from heating. Calculate the increase in internal energy in the process.
View SolutionAn ice cube of mass 0.1 kg at 0∘C is placed in an isolated container which is at 227∘C. The specific heat s of the container varies with temperature T according to the empirical relation s=A+BT, where A=100cal/kgKandB=2×(10−2)cal/kg. If the final temperature of the container is 27∘C, determine the mass of the container.
(Latent heat of fusion for water = 8×(104)cal/kg, specific heat of water =103cal/kg−K).
View SolutionIn a closed insulated container, a liquid is stirred with a paddle to increase the temperature. Which of the following is true?
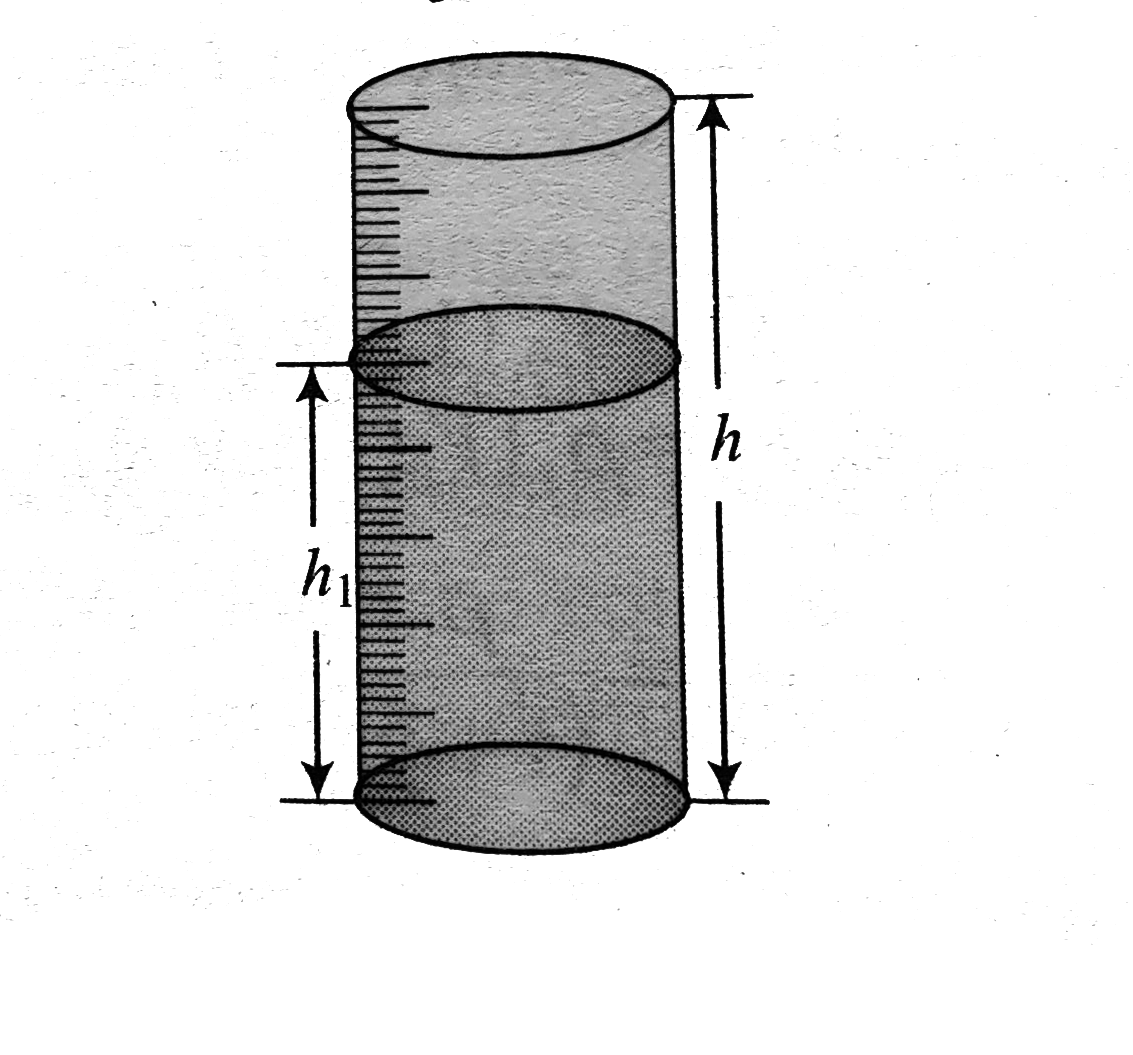
View SolutionConsider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
View SolutionA closed box contains an ideal gas if temperature of gas increased which
View SolutionTwo idential container joined by a small pipe initially contain the same gas at pressure p0 and absolute temperature T0. One container is now maintained at the same temperature while the other is heated to 2T0. The common pressure of the gas
View SolutionTwo identical containers joned by a small pipe initially contain the same gas at pressue po and abosolute temperature To. One container is now mantained at the same temperature while the other is heated to 2T0. The commmon pressure of the gases will be
View Solution
