A block A of mass m is tied to a fixed point C on a horizontal table through a string passing round a massless smooth pulley B. A force F is applied by the experimenter to the pulley. Show that if the pulley is displaced by a distance x the block will be displaced by 2x. Find the acceleration of the block and the pulley.
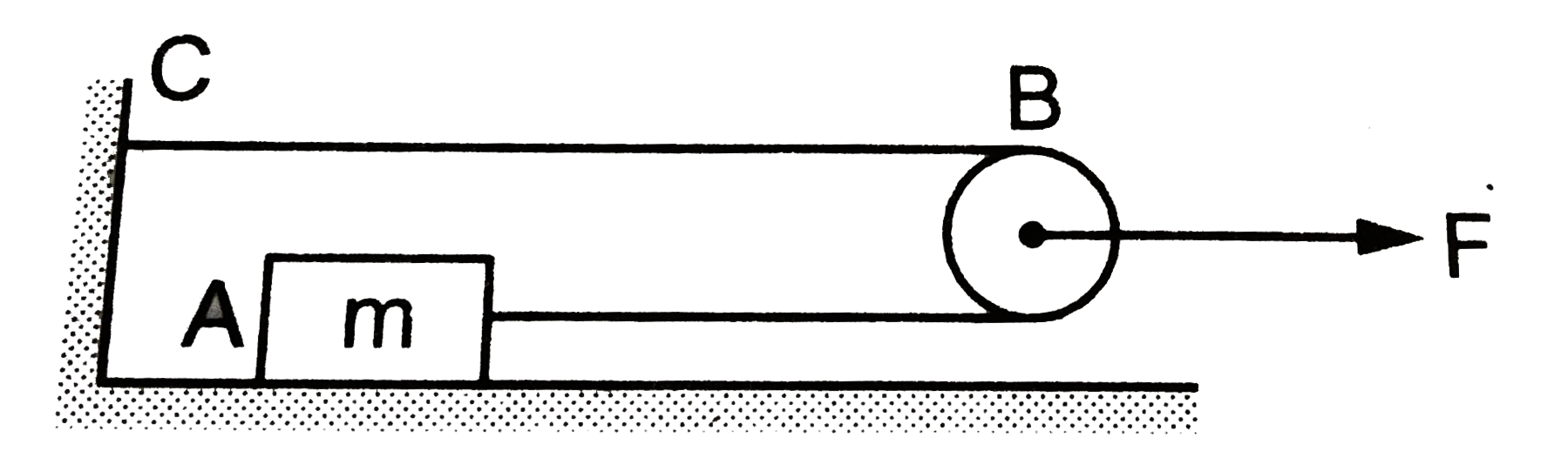
Suppose the pulley is displaced to B and the block to A. The length of the string is and is also equal to . Hence, or, A'A=2BBF - 2T = 0T =F / 2a=T/m=F/(2m)a/2=F/(4m)
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective -114videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective -29videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer17videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - All Questions64videos
LAWS OF THERMODYNAMICS
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer14videos
PHYSICS AND MATHEMATICS
Similar Questions
A block of mass 20 kg is set into motion on a frictionless horizontal surface with the help of massless smooth pulley and rope as shown in the figure. Find the acceleration of the pulley
_PHY_E05_192_Q05.png) View Solution
View SolutionA block of mass of 20kg is set into motion on a frictionless horizontal surface with the help of massless smooth pulley and rope as shown in figure.Find the acceleration of the pulley
_PHY_E05_034_Q01.png) .jpgView Solution
.jpgView SolutionFind the acceleration of blocks in fig. The pulley and the string are massless.
 View Solution
View SolutionTwo block of masses m=5kgandM=10kg are connected by a string passing over a pulley B as shown. Another string connect the center of pulley B to the floor and passes over another pulley A as shown. An upwards force F is applied at the center of pulley A. Both the pulleys are massless.
Find the acceleration of block m and M, if F is
(a) 100N
(b)300N
(c)500N(Takeg=10m/s2)
 View Solution
View SolutionConsider the situation shown in the figure. The surface is smooth and the string and the pulley are light. Find the acceleration of each block and tension in the string.
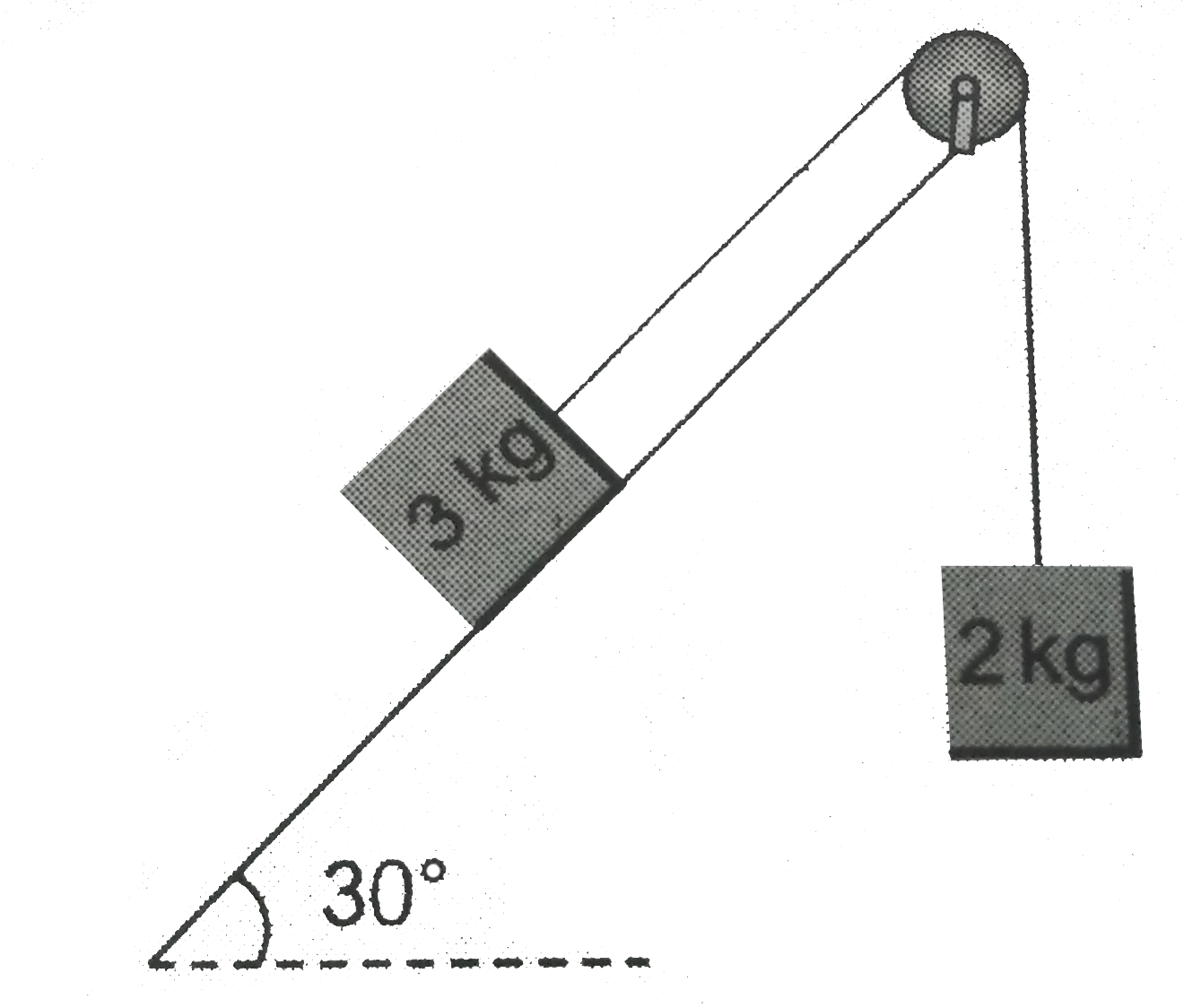 View Solution
View Solution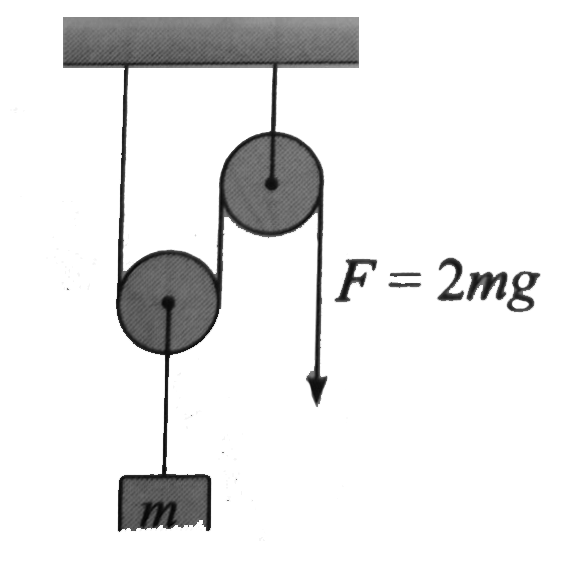
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will beView Solution
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will beView Solution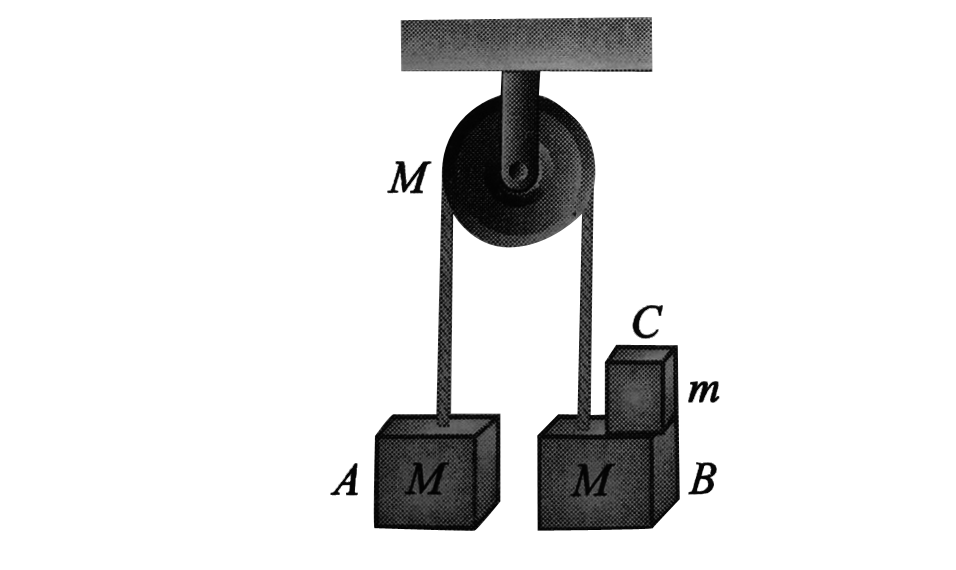
For the following system shown assume that pulley is frictionless, string is massless (m remains on M),
Q. The acceleration of the block A isView Solution
For the following system shown assume that pulley is frictionless, string is massless (m remains on M),
Q. The acceleration of the block A isView SolutionThe strings are inextensible and light: the pulleys are smooth and light. Find the acceleration of each block and tensions in the strings.
 View Solution
View Solution

