A particle is observed from two frames S1andS2. Lthe frame S2 moves with respect toS1 with an acceleration a. Let F1andF2 be the pseudo forces on the particle when seen from S1andS2 respectively. Which of the following are not possible/
F1=0,F2≠0
F1≠0,F2=0
F1≠0,F2≠0
F1=0,F2=0
The correct Answer is:D
To solve the problem, we need to analyze the pseudo forces acting on a particle observed from two different frames of reference, S1 and S2. The frame S2 is moving with respect to S1 with an acceleration a. We will evaluate the four scenarios presented in the question regarding the pseudo forces F1 and F2.
1. Understanding Pseudo Forces:
- A pseudo force is an apparent force that acts on an object when viewed from a non-inertial frame of reference.
- If a frame is accelerating, an observer in that frame will perceive a pseudo force acting on objects within that frame.
2. Analyzing the First Condition: F1=0 and F2≠0
- If F1=0, it implies that frame S1 is either at rest or moving with a constant velocity (inertial frame).
- Since S2 is accelerating with respect to S1, F2 must be non-zero.
- Conclusion: This condition is possible.
3. Analyzing the Second Condition: F1≠0 and F2=0
- If F1≠0, it means that frame S1 is accelerating.
- If F2=0, it means that frame S2 is inertial (not accelerating).
- However, since S2 is defined to be accelerating with respect to S1, this condition cannot hold.
- Conclusion: This condition is not possible.
4. Analyzing the Third Condition: F1≠0 and F2≠0
- If both F1 and F2 are non-zero, it indicates that both frames S1 and S2 are accelerating.
- This is a valid scenario as both frames can have their own accelerations.
- Conclusion: This condition is possible.
5. Analyzing the Fourth Condition: F1=0 and F2=0
- If both pseudo forces are zero, it means both frames are inertial frames (not accelerating).
- However, since S2 is defined to be accelerating with respect to S1, this condition cannot hold.
- Conclusion: This condition is not possible.
Final Answer:
The conditions that are not possible are:
- F1≠0 and F2=0
- F1=0 and F2=0
- A pseudo force is an apparent force that acts on an object when viewed from a non-inertial frame of reference.
- If a frame is accelerating, an observer in that frame will perceive a pseudo force acting on objects within that frame.
- If F1=0, it implies that frame S1 is either at rest or moving with a constant velocity (inertial frame).
- Since S2 is accelerating with respect to S1, F2 must be non-zero.
- Conclusion: This condition is possible.
- If F1≠0, it means that frame S1 is accelerating.
- If F2=0, it means that frame S2 is inertial (not accelerating).
- However, since S2 is defined to be accelerating with respect to S1, this condition cannot hold.
- Conclusion: This condition is not possible.
- If both F1 and F2 are non-zero, it indicates that both frames S1 and S2 are accelerating.
- This is a valid scenario as both frames can have their own accelerations.
- Conclusion: This condition is possible.
- If both pseudo forces are zero, it means both frames are inertial frames (not accelerating).
- However, since S2 is defined to be accelerating with respect to S1, this condition cannot hold.
- Conclusion: This condition is not possible.
The conditions that are not possible are:
- F1≠0 and F2=0
- F1=0 and F2=0
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Exercises
42 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer
17 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective -1
14 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMA ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - All Questions
64 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
Similar Questions
Which of the following orbitals are possible ?
1s,2s,3f,3d
View SolutionA particle is executing S.H.M. Ifu1 and u2 are the velocitiesof the particle at distancesx1 andx2 from the mean position respectively, then
View SolutionA particle is found to be at rest when seen from a frame S1 and moving with a constant velocity when seen from another frame S2. Select the possible options :
View SolutionA constatnt force of 10 N act on a block as shown in figure intal velocity of the particle is zero. It is observed from three frames of reference S1,S2 and ground. The frame S1 start from rest and move with constnat acceleration 10m/s. The frames S1andS2 and particle start moving simultaneously at t=0.
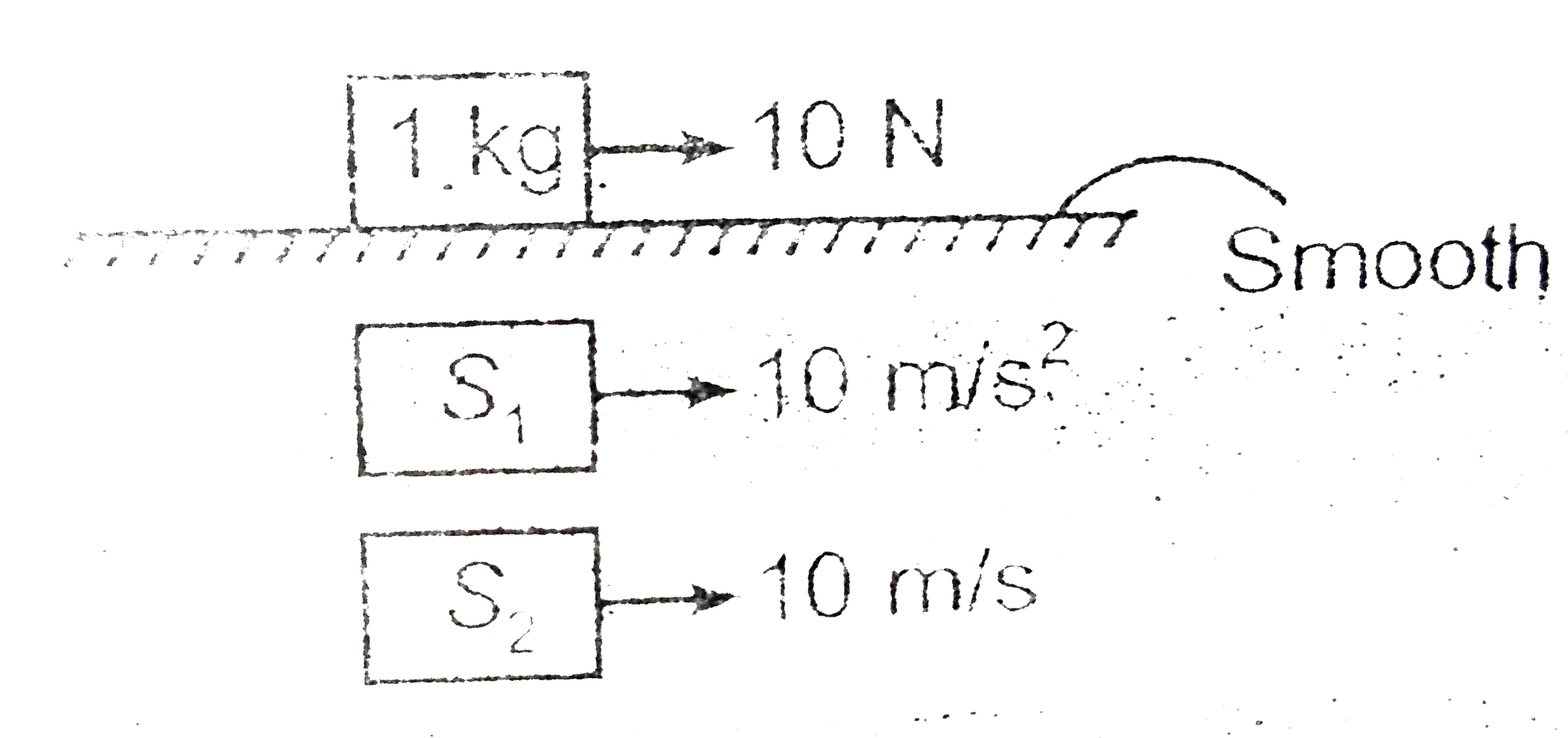
The rate at which work is done by constant force on the particle is always non-negative when observed fom
View SolutionA constant force of 10 N act on a block as shown in figure initial velocity of the block is zero. It is observed from three frames of reference S1,S2 and ground. The frame S1 start from rest and move with constnat acceleration 10m/s2. The frames S1andS2 and particle start moving simultaneously at t=0.
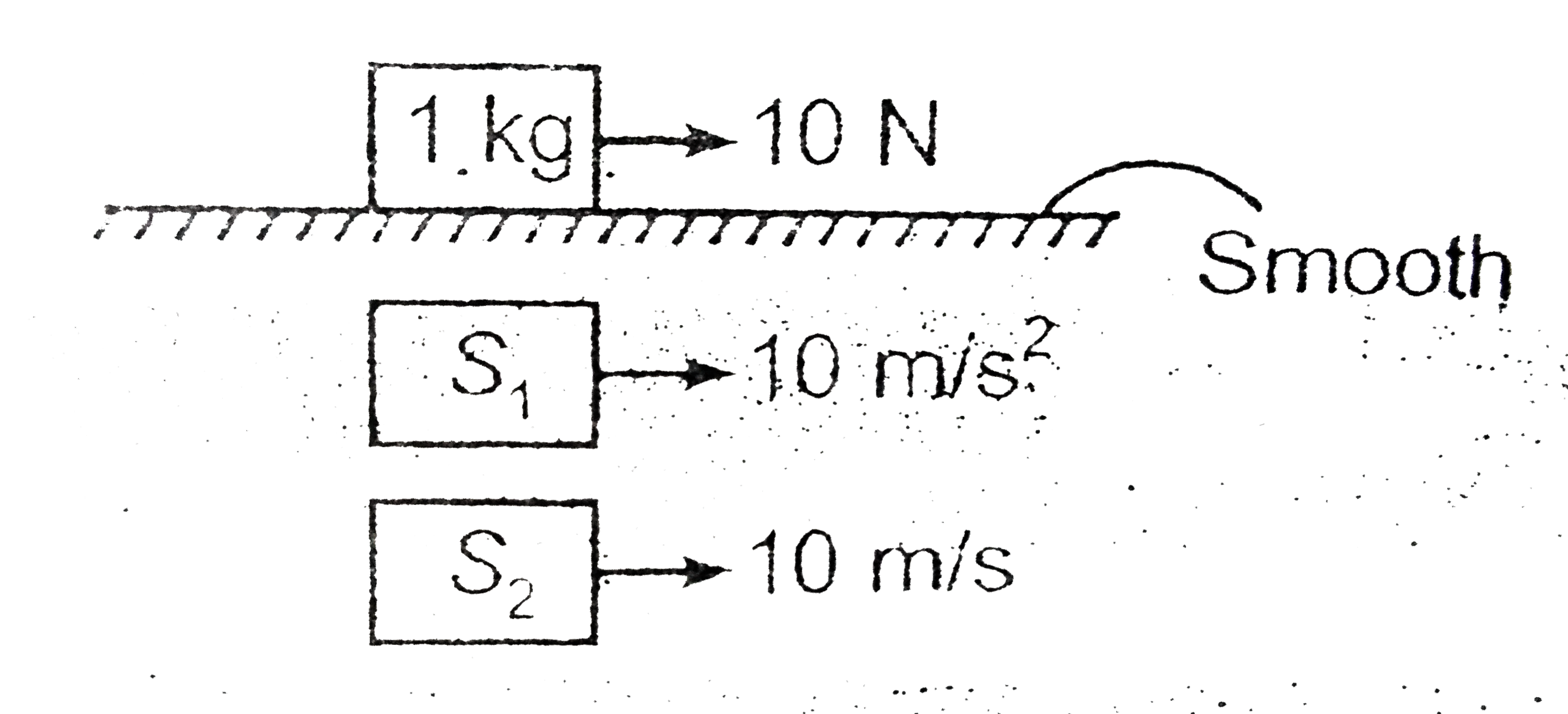
Work done on block in 1 s as observed from frame S1 is
View SolutionA constant force of 10 N act on a block as shown in figure initial velocity of the block is zero. It is observed from three frames of reference S1,S2 and ground. The frame S1 start from rest and move with constnat acceleration 10m/s2. The frames S1andS2 and block start moving simultaneously at t=0.
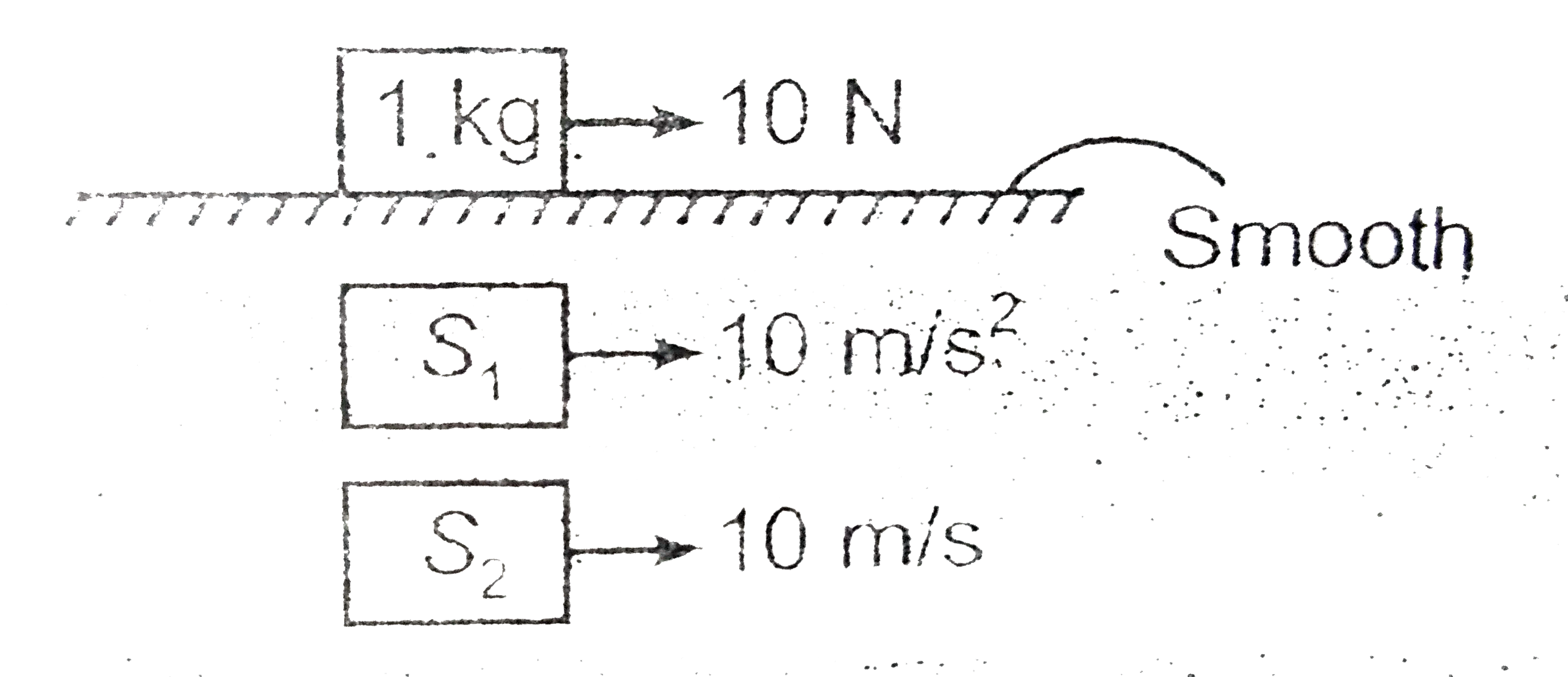
Net work done on block in 1 s as observed from frame S2 is
View SolutionStarting from rest, the acceleration of a particle is a=2(t-1). The velocity of the particle at t=5s is :-
View SolutionA particle starts to move in a straight line from a point with velocity 10 m s−1 and acceleration - 2.0 ms−2. Find the position and velocity of the particle at (i) t = 5 s, (ii) t' = 10 s.
View SolutionTwo frame S1 and S2 are non-inertial. Then frame S2 when observed from S1 is inertial.
A frame in motion is not necessarily a non-inertial frame
View SolutionTwo frame S1 and S2 are non-inertial. Then frame S2 when observed from S1 is inertial.
A frame in motion is not necessarily a non-inertial frame
View Solution
