Let F,FNandf denote the magnitudes of the contact force, normal force and the friction exerted by one surface on the other kept in contact. If none of these is zero,
F>FN
F>1
F_N > f
FN−f<F<FN+f.
The correct Answer is:A, B, D
To solve the problem, we need to analyze the relationships between the contact force (F), normal force (Fn), and frictional force (f) when none of these forces is zero.
1. Understanding the Forces:
- The contact force (F) is the resultant of the normal force (Fn) and the frictional force (f).
- The normal force acts perpendicular to the surfaces in contact, while the frictional force acts parallel to the surfaces.
2. Using Vector Addition:
- Since Fn and f are perpendicular to each other, we can use the Pythagorean theorem to find the magnitude of the contact force:
F=√F2n+f2
3. Analyzing the Magnitudes:
- From the equation F=√F2n+f2, we can deduce that:
- F is always greater than both Fn and f because both Fn and f are squared and added together.
4. Frictional Force Relation:
- The frictional force can be expressed as:
f=μFn
- Here, μ is the coefficient of friction, which can vary depending on the surfaces in contact.
5. Comparing Normal Force and Frictional Force:
- The relationship between Fn and f depends on the value of μ:
- If μ>1, then f can be greater than Fn.
- If μ<1, then Fn can be greater than f.
- Therefore, it is not always true that Fn>f.
6. Verifying the Options:
- Option 1: F>Fn (True)
- Option 2: F>f (True)
- Option 3: Fn>f (Not always true)
- Option 4: Fn−f<F<Fn+f (True)
- This can be shown by manipulating the inequalities derived from the Pythagorean theorem.
Conclusion:
The correct options are 1, 2, and 4.
- The contact force (F) is the resultant of the normal force (Fn) and the frictional force (f).
- The normal force acts perpendicular to the surfaces in contact, while the frictional force acts parallel to the surfaces.
- Since Fn and f are perpendicular to each other, we can use the Pythagorean theorem to find the magnitude of the contact force:
F=√F2n+f2
- From the equation F=√F2n+f2, we can deduce that:
- F is always greater than both Fn and f because both Fn and f are squared and added together.
- The frictional force can be expressed as:
f=μFn
- Here, μ is the coefficient of friction, which can vary depending on the surfaces in contact.
- The relationship between Fn and f depends on the value of μ:
- If μ>1, then f can be greater than Fn.
- If μ<1, then Fn can be greater than f.
- Therefore, it is not always true that Fn>f.
- Option 1: F>Fn (True)
- Option 2: F>f (True)
- Option 3: Fn>f (Not always true)
- Option 4: Fn−f<F<Fn+f (True)
- This can be shown by manipulating the inequalities derived from the Pythagorean theorem.
The correct options are 1, 2, and 4.
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Exercises
31 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -1
10 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
Similar Questions
Let Fpp,FpnandF∩ denote the magnitudes of the nuclear force by a proton on a proton, by a proton on a neutron and by a neutron on a neutron respectively. When the separation is 1fm,
View SolutionLet F1 be the magnitude of the gravitational force exerted on the Sun by Earth and F2 be the magnitude of the force exerted on Earth by the Sun. Then:
View SolutionThe angle between the resultant contact force and the normal force exerted by a body on the other is called the angle of friction. Show that it λ be the angle of friction and μ the coefficient of static friction. λ≤tan−1μ.
View SolutionChoose the correct statement(s) about the frictional force between two solid surfaces in contact
View SolutionAssertion: Magnitude of the contact force is always greater than the magnitude of frictional force.
Reason: Contact force is the resultant of the friction force and normal reaction.
View SolutionTwo forces of magnitude F are acting on a uniform disc kept on a horizontal rough surface as shown in the figure. Friction force by the horizontal surface on the disc is nF. Find the value of n.
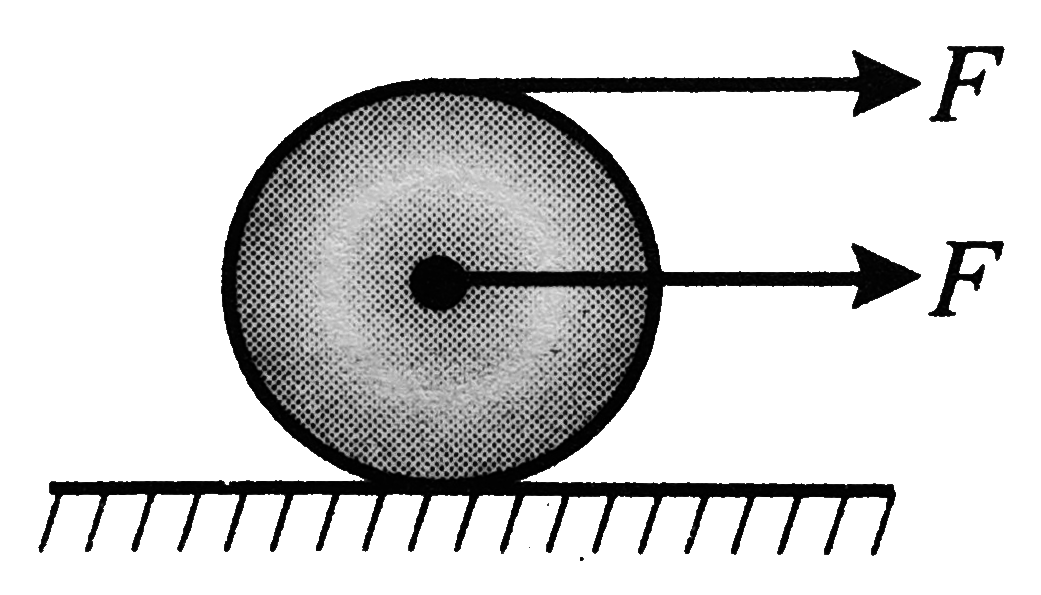
View SolutionAssertion: Frictional force is the component of contact force parallel to the surface.
Reason: Friction force always opposes the motion of a body.
View SolutionDo the forces of friction and other contact forces arises due to gravitational attraction? If not, what is the origin of these forces?
View SolutionA block is kept on a smooth inclined plane of angle of inclination 30∘ that moves with a constant acceleration so that the block does not slide relative to the inclined plane. Let F1 be the the contact force between the block and the plane . Now the inclined plane stops and let F2 be the contact force between the two in this case. Then, F1/F2 is
View SolutionA block of mass 70 kg is kept on a rough horizontal surface and coefficient of static friction between block and surface is 0.4 . A man is trying to pull the block by applying a horizontal force .The net contact force exerted by the surface on the block is F , then:
View Solution
