Can two particles be in equilibrium under the action of their mutual gravitational force? Can three particle be? Can one of the three particles be?
To determine whether two, three, or one of three particles can be in equilibrium under the action of their mutual gravitational forces, let's analyze each case step by step.
Step 1: Two Particles
1. Understanding Gravitational Force: The gravitational force between two particles is always attractive. If we have two particles, say A and B, they will exert a gravitational force on each other that pulls them together.
2. Equilibrium Condition: For a particle to be in equilibrium, the net force acting on it must be zero. However, in the case of two particles, the force exerted by A on B and the force exerted by B on A are equal in magnitude but opposite in direction.
3. Conclusion: Since the gravitational force always pulls the two particles towards each other, they cannot be in equilibrium. Therefore, two particles cannot be in equilibrium under their mutual gravitational force.
Step 2: Three Particles
1. Analyzing Three Particles: Let’s consider three particles A, B, and C. Similar to the case of two particles, each pair of particles will exert a gravitational force on each other.
2. Resultant Forces: The gravitational forces between each pair will create a resultant force on each particle. Since these forces are always attractive, there will always be a net force acting on at least one of the particles.
3. Conclusion: Therefore, three particles cannot be in equilibrium under their mutual gravitational forces.
Step 3: One of the Three Particles
1. Considering One Particle in Motion: Now, let’s consider the scenario where we have three particles, and we want to see if one of them can be in equilibrium while the others are in motion.
2. Centrifugal Force and Gravitational Force: If two particles (say A and B) are moving in a circular path due to their mutual gravitational attraction, they will experience a centripetal force directed towards the center of the circular path. The gravitational force acts as this centripetal force.
3. Equilibrium of the Third Particle: The third particle (C) can be positioned such that the gravitational forces from A and B on C balance each other out. If A and B are moving in a way that their gravitational pulls on C are equal and opposite, C can be in equilibrium.
4. Conclusion: Therefore, one of the three particles can be in equilibrium under specific conditions while the other two are in motion.
Summary of Conclusions:
- Two particles cannot be in equilibrium under mutual gravitational force.
- Three particles cannot be in equilibrium under mutual gravitational forces.
- One of the three particles can be in equilibrium under specific conditions while the other two are in motion.
1. Understanding Gravitational Force: The gravitational force between two particles is always attractive. If we have two particles, say A and B, they will exert a gravitational force on each other that pulls them together.
2. Equilibrium Condition: For a particle to be in equilibrium, the net force acting on it must be zero. However, in the case of two particles, the force exerted by A on B and the force exerted by B on A are equal in magnitude but opposite in direction.
3. Conclusion: Since the gravitational force always pulls the two particles towards each other, they cannot be in equilibrium. Therefore, two particles cannot be in equilibrium under their mutual gravitational force.
1. Analyzing Three Particles: Let’s consider three particles A, B, and C. Similar to the case of two particles, each pair of particles will exert a gravitational force on each other.
2. Resultant Forces: The gravitational forces between each pair will create a resultant force on each particle. Since these forces are always attractive, there will always be a net force acting on at least one of the particles.
3. Conclusion: Therefore, three particles cannot be in equilibrium under their mutual gravitational forces.
1. Considering One Particle in Motion: Now, let’s consider the scenario where we have three particles, and we want to see if one of them can be in equilibrium while the others are in motion.
2. Centrifugal Force and Gravitational Force: If two particles (say A and B) are moving in a circular path due to their mutual gravitational attraction, they will experience a centripetal force directed towards the center of the circular path. The gravitational force acts as this centripetal force.
3. Equilibrium of the Third Particle: The third particle (C) can be positioned such that the gravitational forces from A and B on C balance each other out. If A and B are moving in a way that their gravitational pulls on C are equal and opposite, C can be in equilibrium.
4. Conclusion: Therefore, one of the three particles can be in equilibrium under specific conditions while the other two are in motion.
- Two particles cannot be in equilibrium under mutual gravitational force.
- Three particles cannot be in equilibrium under mutual gravitational forces.
- One of the three particles can be in equilibrium under specific conditions while the other two are in motion.
Topper's Solved these Questions
Similar Questions
Two particles of equal mass go round a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View Solution_S01_003_Q01.png)
Two particles of equal mass (m) each move in a circle of radius (r) under the action of their mutual gravitational attraction find the speed of each particle.
View Solution
Knowledge Check
Two particles of equal mass go around a circle of radius R under the action of their mutual gravitational attraction. The speed v of each particle is
A12R√(1Gm)B√(Gm2R)C12√(GmR)D√(4GmR)
Two particles of equal masses m go round a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is v=√GmnR. Find the value of n.
View SolutionFour particles of equal masses M move along a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionThree charged particle sare in equilibrium under their electrostatic forces only. Then
View SolutionA body is in equilibrium under the action of three coplanar forces P,QandR as shown in figure. Select the correct statement.
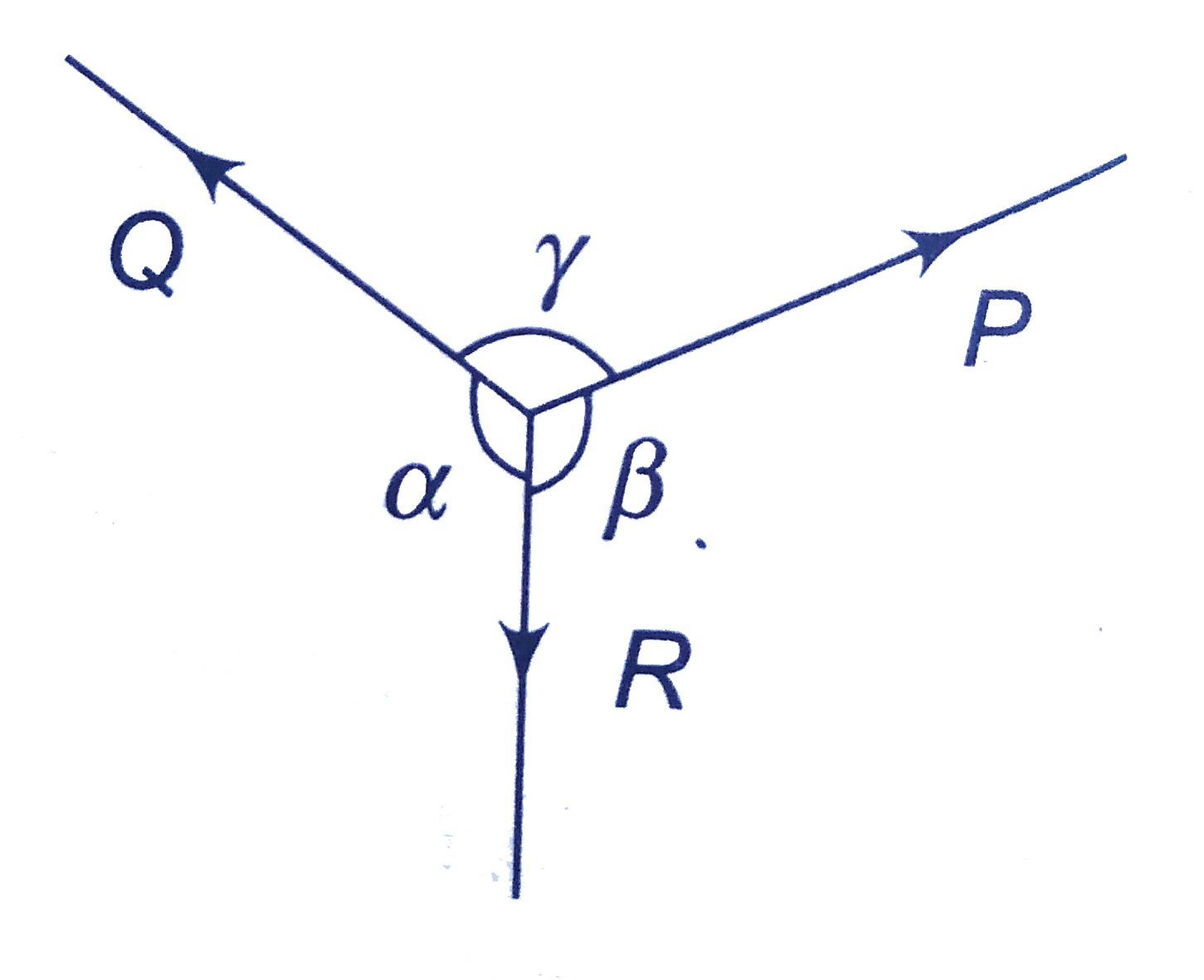
View Solution
