A car runs east to west and another car B of the same mass runs from west to east at the same path along the equator. A presses the track with a force and B presses the track with a force . Then
the information is insufficient to find the relation between .
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on both cars A and B as they move along the equator of the Earth. Both cars have the same mass and are moving in opposite directions.
1. Understanding the Motion:
- Car A moves from east to west, while car B moves from west to east. Both are moving along the equator of the Earth.
2. Identifying Forces:
- Each car experiences gravitational force downward, which is mg, where m is the mass of the car and g is the acceleration due to gravity.
- Each car also experiences a centrifugal force due to the Earth's rotation, which acts outward (upward) and is given by mv2R, where v is the velocity of the car relative to the Earth's rotation and R is the radius of the Earth.
3. Calculating the Velocities:
- For car A (moving against the rotation of Earth):
vA=ωR−v
- For car B (moving with the rotation of Earth):
vB=ωR+v
- Here, ω is the angular velocity of the Earth.
4. Comparing Velocities:
- Since vB includes an additional v compared to vA, we conclude:
vB>vA
5. Setting Up the Equations for Normal Force:
- For car A, the normal force N1 is given by:
N1+mv2AR=mg
Rearranging gives:
N1=mg−mv2AR
- For car B, the normal force N2 is given by:
N2+mv2BR=mg
Rearranging gives:
N2=mg−mv2BR
6. Comparing Normal Forces:
- Since vB>vA, it follows that:
mv2BR>mv2AR
- Therefore, substituting back into the equations for N1 and N2:
N2<N1
- Thus, we conclude that:
N1>N2
7. Final Conclusion:
- The correct answer is that the normal force exerted by car A on the track is greater than that exerted by car B:
N1>N2
- Car A moves from east to west, while car B moves from west to east. Both are moving along the equator of the Earth.
- Each car experiences gravitational force downward, which is mg, where m is the mass of the car and g is the acceleration due to gravity.
- Each car also experiences a centrifugal force due to the Earth's rotation, which acts outward (upward) and is given by mv2R, where v is the velocity of the car relative to the Earth's rotation and R is the radius of the Earth.
- For car A (moving against the rotation of Earth):
vA=ωR−v
- For car B (moving with the rotation of Earth):
vB=ωR+v
- Here, ω is the angular velocity of the Earth.
- Since vB includes an additional v compared to vA, we conclude:
vB>vA
- For car A, the normal force N1 is given by:
N1+mv2AR=mg
Rearranging gives:
N1=mg−mv2AR
- For car B, the normal force N2 is given by:
N2+mv2BR=mg
Rearranging gives:
N2=mg−mv2BR
- Since vB>vA, it follows that:
mv2BR>mv2AR
- Therefore, substituting back into the equations for N1 and N2:
N2<N1
- Thus, we conclude that:
N1>N2
- The correct answer is that the normal force exerted by car A on the track is greater than that exerted by car B:
N1>N2
Topper's Solved these Questions
- CIRCULAR MOTIONBook:HC VERMA ENGLISHChapter:CIRCULAR MOTIONExercise:Objective -2
Explore 6 Videos
- CIRCULAR MOTIONBook:HC VERMA ENGLISHChapter:CIRCULAR MOTIONExercise:Exercises
Explore 30 Videos
- CIRCULAR MOTIONBook:HC VERMA ENGLISHChapter:CIRCULAR MOTIONExercise:worked out Examples
Explore 13 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBook:HC VERMA ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONExercise:Objective -2
Explore 11 Videos
- FLUID MECHANICSBook:HC VERMA ENGLISHChapter:FLUID MECHANICSExercise:All Questions
Explore 90 Videos
Similar Questions
Two motor boats A and B move from same point along a circle of radius 10 m in still water. The boats are so designed that they can move only with constant speeds. The boats A and B take 16 and 8 sec respectively to complete one circle in stationary water. Now water starts flowing at t=0 with a speed 4ms in a fixed direction. Find the distance between the boats after t=8 sec.
View Solution
Two trains A and B each of mass m are moving on a longitude of a spherical planet of mass M and radius R with velocity v relative to to planet. Train A & B presses thhe rail track with forces NA & NB respectively. [ω is angular velocity of planet about its vertical axis] Then
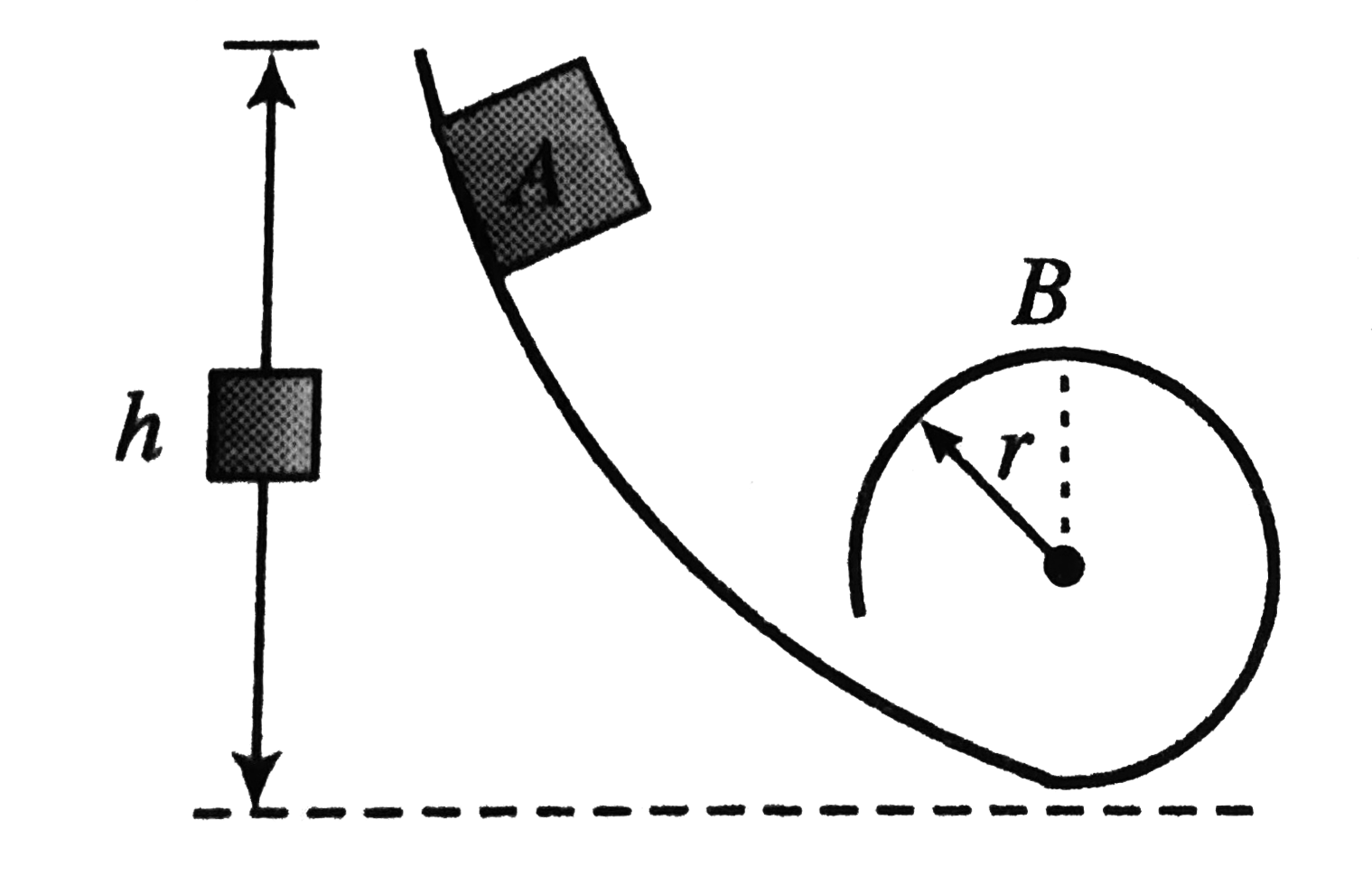
View SolutionA mass m starting from A reaches B of a frictionless track. On reaching B, it pushes the track with a force equal to x times its weight, then the applicable relation is
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionAn automobile engine propels a 1000 kg car (A) along a levelled road at a speed of 36 km//h. Find the power if the opposing frictional force is 100 N Now, suppose after travelling a distance of 200 m, this car collides with another stationary car (B) of same mass and comes to rest. Let its engine also stop at the same time. Now car (B) starts moving on the same level road without getting its engine started. Find the speed of the car (B) just after the collision.
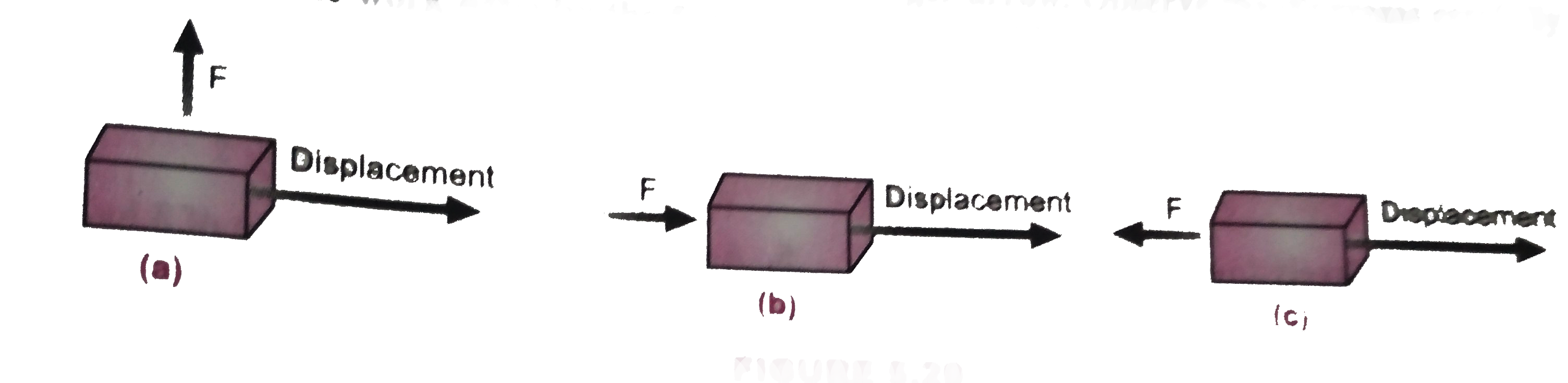
View SolutionIn each of the following, a force, F is acting on an object of mass, m. The direction of displacement is from west to east shown by the longer arrow. Observe the diagrams carefully and state whether the work done by the force is negative, positive or zero.
View SolutionFind the pressure exerted at the tip of a board pin, of area 0.1 mm2, if it is pressed against the board with a force 15 N.
View SolutionPoints A and B are 70 km. apart on a highway. A car starts from A and another car starts from B simultaneously. If they travel in the same direction, they meet in 7 hours, but if they travel towards each other, they meet in one hour. Find the speed of the two cars.
View SolutionWind is blowing west to east along twoparallelracs. Two trais moving with the same speed in opposite directions on these tracks have the steam tracks. If one stream track isdouble than the other, what is the speed of each train ?
View Solution