A block is mass m moves on as horizontal circle against the wall of a cylindrical room of radius R. The floor of the room on which the block moves is smooth but the friction coefficient between the wall and the block is μ. The block is given an initial speed v0. As a function of the speed v write a. the normal force by the wall on the block. b. the frictional force by the wall and c. the tangential acceleration of the block. d. Integrate the tangential acceleration (dvdt=vdvds) to obtain the speed of the block after one revoluton.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the forces acting on the block and derive the required expressions.
Step 1: Determine the Normal Force by the Wall on the Block
The block is moving in a circular path, and the normal force (N) exerted by the wall on the block provides the necessary centripetal force for circular motion. The centripetal force required for an object of mass m moving with speed v in a circle of radius R is given by:
Fc=mv2R
Thus, the normal force N can be expressed as:
N=mv2R
Step 2: Determine the Frictional Force by the Wall
The frictional force f acting on the block is given by the product of the normal force and the coefficient of friction μ:
f=μN=μ(mv2R)=μmv2R
Step 3: Determine the Tangential Acceleration of the Block
The tangential acceleration at can be calculated using the frictional force. According to Newton's second law, the tangential acceleration is given by:
at=fm=μmv2Rm=μv2R
Step 4: Integrate the Tangential Acceleration to Obtain Speed After One Revolution
We start with the relationship between tangential acceleration and speed:
dvdt=at=μv2R
Using the chain rule, we can write:
dvdt=vdvds
Thus, we have:
vdvds=μv2R
Dividing both sides by v (assuming v≠0):
dvds=μvR
Now, we can separate variables and integrate:
dvv=μRds
Integrating both sides:
∫dvv=∫μRds
This gives us:
lnv=μRs+C
To find the constant C, we use the initial condition. When s=0, v=v0:
lnv0=C
Substituting back into the equation:
lnv=μRs+lnv0
Exponentiating both sides:
v=v0eμRs
Speed After One Revolution
After one complete revolution, the displacement s is equal to the circumference of the circle, which is 2πR:
v=v0eμR(2πR)=v0e2πμ
Summary of Results
a. The normal force by the wall on the block is:
N=mv2R
b. The frictional force by the wall is:
f=μmv2R
c. The tangential acceleration of the block is:
at=μv2R
d. The speed of the block after one revolution is:
v=v0e2πμ
The block is moving in a circular path, and the normal force (N) exerted by the wall on the block provides the necessary centripetal force for circular motion. The centripetal force required for an object of mass m moving with speed v in a circle of radius R is given by:
The frictional force f acting on the block is given by the product of the normal force and the coefficient of friction μ:
The tangential acceleration at can be calculated using the frictional force. According to Newton's second law, the tangential acceleration is given by:
We start with the relationship between tangential acceleration and speed:
After one complete revolution, the displacement s is equal to the circumference of the circle, which is 2πR:
a. The normal force by the wall on the block is:
N=mv2R
f=μmv2R
at=μv2R
v=v0e2πμ
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -2
6 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
Similar Questions
Block A is moving away from the wall at a speed v and acceleration a.
View SolutionA block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass 1 kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5, magnitude of the frictional force acting on the block is
View SolutionFor the arrangement shown in the figure the coefficient of friction between the two blocks is μ. If both the blocks are identical and moving ,then the acceleration of each block is
View SolutionA 250 g block slides on aeroug horizontal table. Find the work donen by the frictinal force in bringing the block to rest if it is initially moving at a speed of 40 cm/s. If the friction coefficient between te table and the block is 0.1 how far does the block move before coming to rest?
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA block of mass 0.1 kg is held against a wall applying a horizontal force of 5 N on the block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is :
View SolutionA block of mass 0.1 is held against a wall applying a horizontal force of 5N on block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is:
View SolutionA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
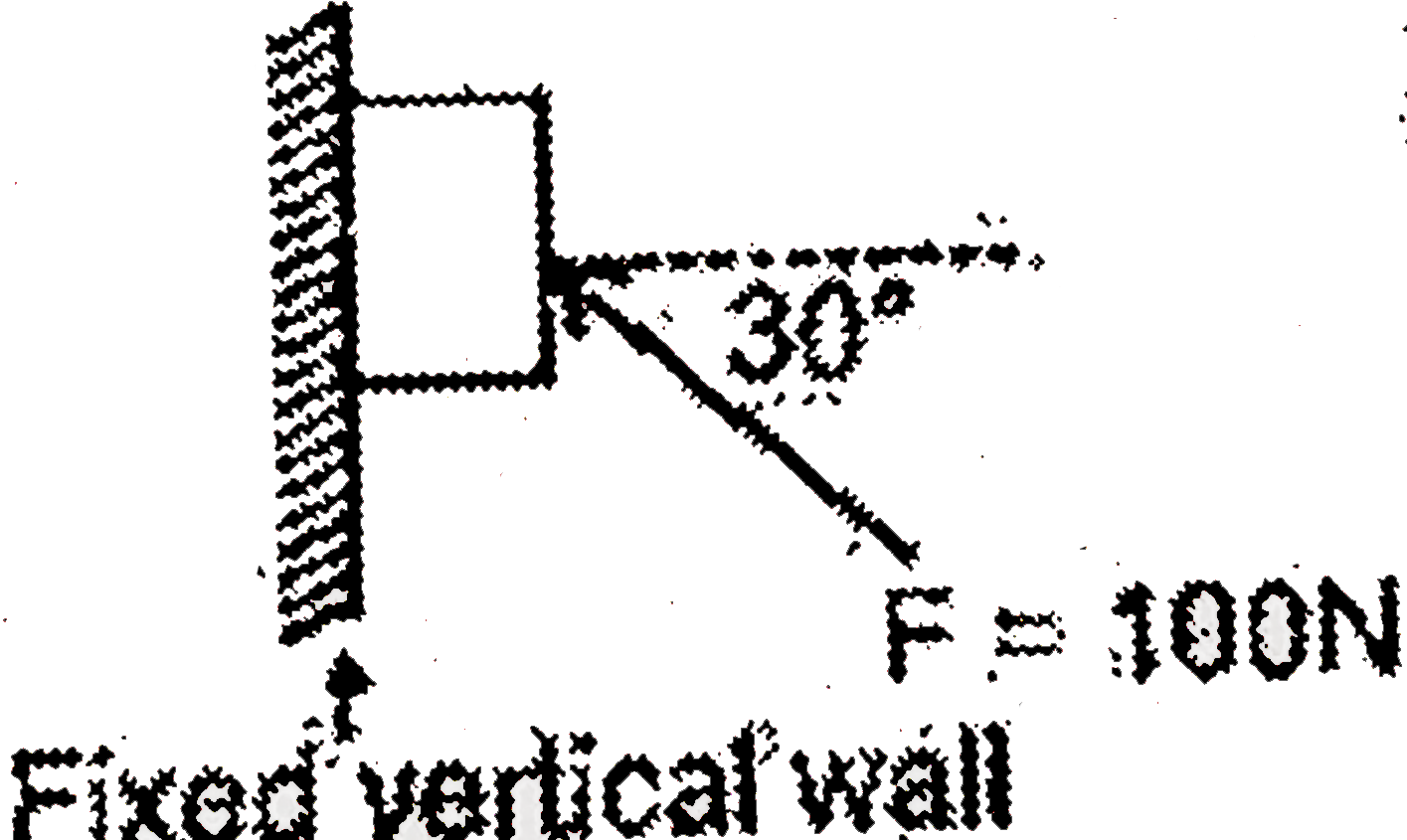
View SolutionA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
View Solution
