Can you think of two particles which do not exert gravitational force on each other?
To answer the question, "Can you think of two particles which do not exert gravitational force on each other?", we need to analyze the nature of gravitational force as described by Newton's law of gravitation.
1. Understanding Gravitational Force: According to Newton's law of universal gravitation, every particle with mass attracts every other particle with mass. The force of attraction (gravitational force) between two masses m1 and m2 is given by the formula:
F=G⋅m1⋅m2r2
where G is the gravitational constant, and r is the distance between the centers of the two masses.
2. Identifying Masses: Since gravitational force depends on mass, we need to consider two particles that have mass. Even the smallest particles, such as electrons or protons, have mass and therefore exert gravitational forces on each other.
3. Considering Massless Particles: The only scenario where two particles do not exert gravitational force on each other is when at least one of the particles is massless. For example, photons (particles of light) are massless. If we consider a photon and an electron, the photon does not exert a gravitational force on the electron because it has no mass.
4. Conclusion: Therefore, the answer to the question is that two particles can be a photon (massless) and an electron (massive). The photon does not exert a gravitational force on the electron due to its lack of mass.
Final Answer:
Two particles that do not exert gravitational force on each other can be a photon (massless) and an electron (massive).
F=G⋅m1⋅m2r2
where G is the gravitational constant, and r is the distance between the centers of the two masses.
Two particles that do not exert gravitational force on each other can be a photon (massless) and an electron (massive).
Topper's Solved these Questions
Similar Questions
Which of the following pairs particles cannot exert nuclear force on each other ?
View SolutionThree uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View Solution
Knowledge Check
If the distance between two point particles is doubled, then the gravitational force between them
Adecreases by a factor of 4Bdecreases by a factor of 2Cincreases by a factor of 2Dincreases by a factor of 4Assertion: The gravitational force on a particle inside a spherical shell is zero.
Reason: The shell shields other bodies outside it form exerting gravitational forces on a particle inside.AIf both assertion and reason are true and reason is the correct explanation of assertionBIf both assertion and reason are truebut reason is not the correct explanation of assertionCIf assertion is true but reason is falseDIf both assertion and reason are false.
Three uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionThree uniform spheres each having a mass M and radius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
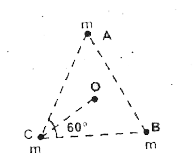
Force acting on particle C, due to particle A and B
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
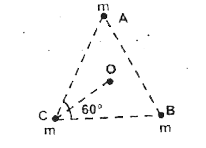
The gravitational potential at O is
View SolutionCan two equipotential surfaces cut each other?
View SolutionThe gravitational force exerted by the Sun on the Moon is about twice as great as the gravitational force exerted by the earth on the Moon, but still Moon is not escaping from the gravitational influence of the earth. Mark the option which correctly explains the above system.
View Solution
