A particle is fastened at the end of a string and whirled in a vertical circle with the other end of the string being fixed. The motion of the particle is
periodic
oscillatory
simple harmonic
with simple harmonic
The correct Answer is:A
To solve the question regarding the motion of a particle fastened at the end of a string and whirled in a vertical circle, we can analyze the nature of the motion step by step.
1. Understanding the Setup:
- A particle (let's say a stone) is attached to the end of a string. The other end of the string is fixed, and the particle is being whirled in a vertical circle.
2. Identifying the Type of Motion:
- The motion of the particle can be described as circular motion since it is moving along a circular path due to the tension in the string and the gravitational force acting on it.
3. Periodic Motion:
- Circular motion is a type of periodic motion. Periodic motion is defined as motion that repeats itself after a fixed interval of time. In this case, the particle completes one full revolution around the circle in a certain time period, thus exhibiting periodic behavior.
4. Oscillatory Motion:
- Oscillatory motion refers to motion that moves back and forth around a central point (like a pendulum). The motion of the particle in a vertical circle does not fit this definition as it does not oscillate about a fixed point but rather moves in a circular path.
5. Simple Harmonic Motion (SHM):
- Simple Harmonic Motion is a specific type of oscillatory motion where the restoring force is directly proportional to the displacement from the equilibrium position and acts in the opposite direction. The motion of the particle in a vertical circle does not exhibit this characteristic, as it is not oscillating about a fixed point.
6. Conclusion:
- Since the particle is undergoing circular motion, which is periodic but not oscillatory or simple harmonic, the correct classification of the motion is periodic.
Final Answer:
The motion of the particle is Periodic.
- A particle (let's say a stone) is attached to the end of a string. The other end of the string is fixed, and the particle is being whirled in a vertical circle.
- The motion of the particle can be described as circular motion since it is moving along a circular path due to the tension in the string and the gravitational force acting on it.
- Circular motion is a type of periodic motion. Periodic motion is defined as motion that repeats itself after a fixed interval of time. In this case, the particle completes one full revolution around the circle in a certain time period, thus exhibiting periodic behavior.
- Oscillatory motion refers to motion that moves back and forth around a central point (like a pendulum). The motion of the particle in a vertical circle does not fit this definition as it does not oscillate about a fixed point but rather moves in a circular path.
- Simple Harmonic Motion is a specific type of oscillatory motion where the restoring force is directly proportional to the displacement from the equilibrium position and acts in the opposite direction. The motion of the particle in a vertical circle does not exhibit this characteristic, as it is not oscillating about a fixed point.
- Since the particle is undergoing circular motion, which is periodic but not oscillatory or simple harmonic, the correct classification of the motion is periodic.
The motion of the particle is Periodic.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective -1
21 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
Similar Questions
A particle tide to the end of a string oscillates along a circular arc in a vertical plane. The other end of the string is fixed at the center of the circle. If the string has a breaking strength of twice the weight of the particles,
a. find the maximum distance that the particle can cover in one cycle of oscillation. The length of the string is 50cm.
b. Find the tension in the extreme position.
c. Find the acceleration of the particle at bottom and extreme position.
View SolutionA ball of mass m is fastened to a string. The ball swings in a vertical circle of radius R with the other end of the string held fixed. Neglecting the air resistance, the difference between the string's tension at the bottom of the circle and at the quarter of the cirlce is
View Solution
Knowledge Check
A 1 kg stone at the end of 1 m long string is whirled in a vertical circle at constant speed of 4 m/sec. The tension in the string is 6 N when the stone is at (g=10m/sec2)
Atop of the cirlceBbottom of the circleChalf way downDnone of the above
A stone tied to a string of length L is whirled in a vertical circle with the other end of the string at the centre. At a certain instant of time the stone is at lowest position and has a speed u . Find the magnitude of the change in its velocity as it reaches a position, where the string is horizontal.
View SolutionA particle of mass m1 is fastened to one end of a string and one of m2 to the middle point, the other end of the string being fastened to a fixed point on a smooth horizontal table The particles are then projected, so that the two portions of the string are always in the same straight line and describes horizontal circles find the ratio of tensions in the two parts of the string

View SolutionA stone of mass of 1 kg is tied to the end of a string 1 m long. It is whirled in a vertical circle. The velocity of the stone at the bottom of the circle is just sufficient to take it to the top of circle without slackening of the string. What is the tension in the string at the top of the circle? (Take, g =10 ms−2)
View SolutionA stone is fastened to one end of a string and is whirled in a verticla circle of radius R. Find the minimum speed the stone can have at the highest point of the circle.
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then
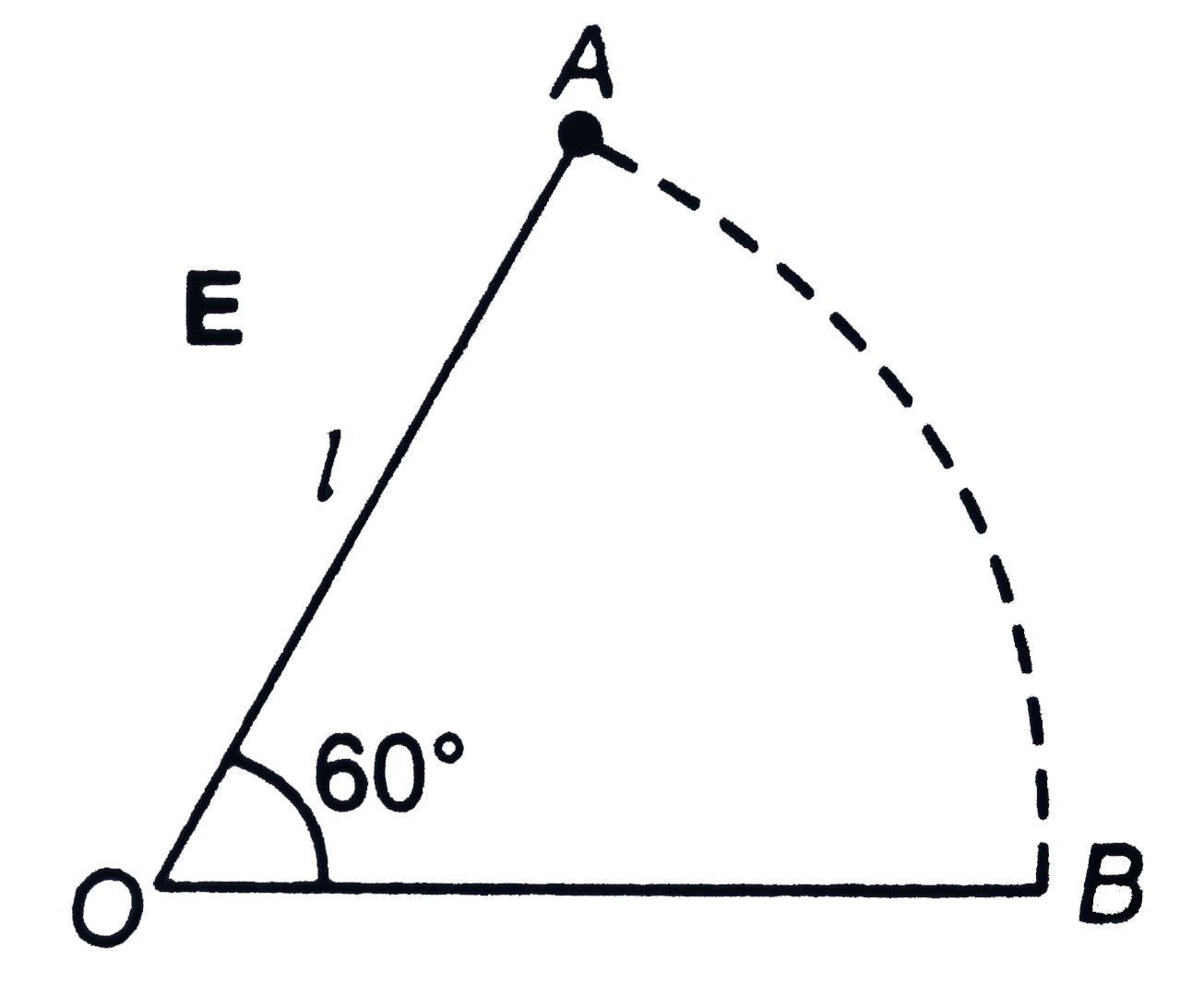
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then

View SolutionAt stone is tied to the end of a string and is whirled in a horizontal aide with a constant speed. Suppose the string breaks suddenly. What happens to the stone ?
View Solution
