A wave pulse passing on a string with speed of 40cms−1 in the negative x direction has its maximum at x=0 at t=0. Where will this maximum be located at t=5s?
The correct Answer is:A, B
To solve the problem step by step, we will analyze the motion of the wave pulse and calculate the position of its maximum at a given time.
Step 1: Understand the given information
- The speed of the wave pulse is v=40cm/s.
- The wave pulse is moving in the negative x-direction.
- At time t=0, the maximum of the wave pulse is located at x=0.
Step 2: Use the formula for distance
The distance traveled by the wave pulse can be calculated using the formula:
Distance=Speed×Time
Here, the speed is 40cm/s and the time is 5s.
Step 3: Calculate the distance traveled
Substituting the values into the formula:
Distance=40cm/s×5s=200cm
Step 4: Determine the direction of movement
Since the wave pulse is moving in the negative x-direction, we need to account for this direction in our final position calculation. Therefore, the position of the maximum at t=5s will be:
x=−200cm
Step 5: Final answer
Thus, at t=5s, the maximum of the wave pulse will be located at:
x=−200cm
---
- The speed of the wave pulse is v=40cm/s.
- The wave pulse is moving in the negative x-direction.
- At time t=0, the maximum of the wave pulse is located at x=0.
The distance traveled by the wave pulse can be calculated using the formula:
Distance=Speed×Time
Here, the speed is 40cm/s and the time is 5s.
Substituting the values into the formula:
Distance=40cm/s×5s=200cm
Since the wave pulse is moving in the negative x-direction, we need to account for this direction in our final position calculation. Therefore, the position of the maximum at t=5s will be:
x=−200cm
Thus, at t=5s, the maximum of the wave pulse will be located at:
x=−200cm
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -2
9 Videos
- THE FORCESBOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer
9 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
Similar Questions
A sinusoidal wave is traveling on a string with speed 40 cm/s. The displacement of the particle of the string at x = 10 cm varies with time according to
y = (5.0cm) sin(1.0−4.0s−1)t)The linear density of the string is 4.0 g/cm
View SolutionThe equation of a wave travelling on a string stretched along the X-axis is given by
y=Ae(−xa+tT)2
(a) Write the dimensions of A, a and T.
(b) Find the wave speed.
(c) In which direction is the wave travelling?
(d) Where is the maximum of the pulse located at t=T and at t=2T?
View SolutionA wave pulse is travelling on a string with a speed v towards the positive X-axis. The shape of the string at t = 0 is given by g(x)=Asin(xa), where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time 1, if the wave speed is v.
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
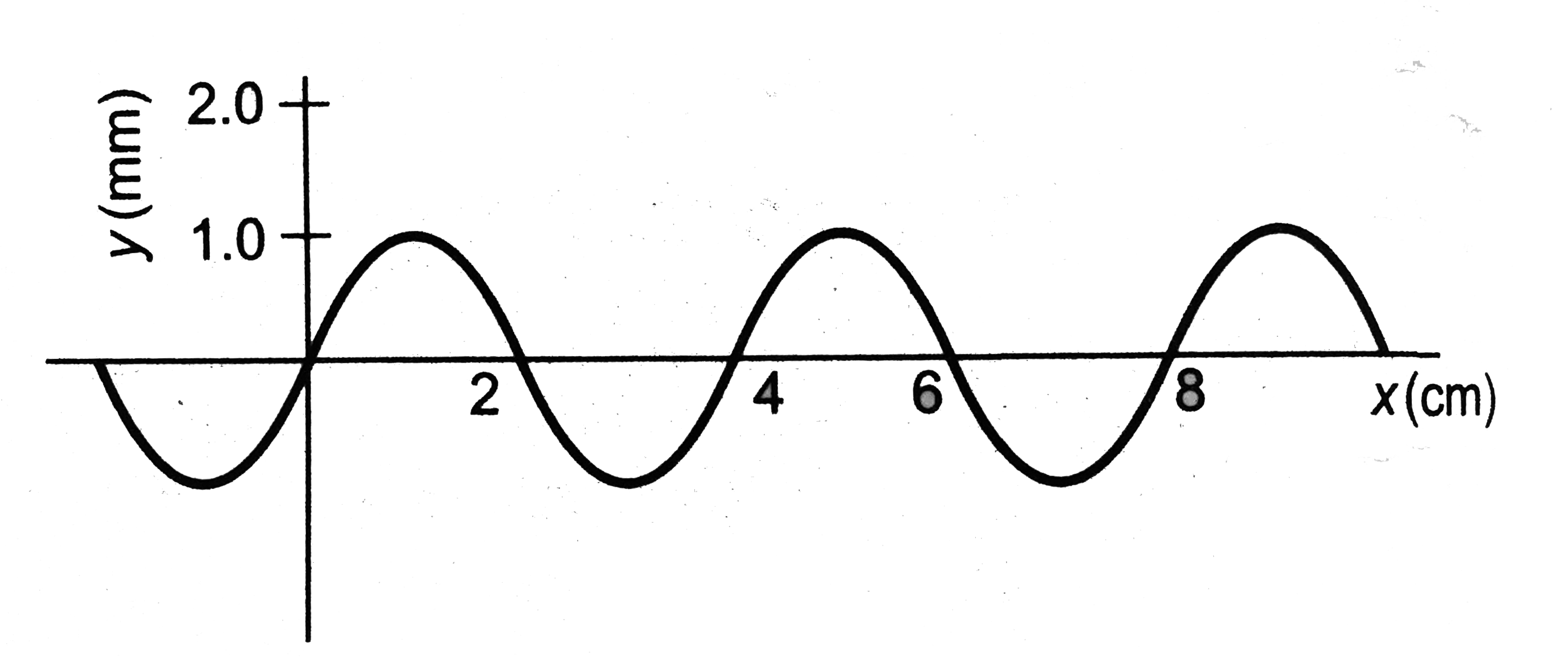
View SolutionA string of linear mass density 0.5gcm−1 and a total length 30 cm is tied to a fixed wall at one end and to a frictionless ring at the other end. The ring can move on a vertical rod. A wave pulse is produced on the string which moves towards the ring at a speed of 20cms−1. The pulse is symmetric about its maximum which is located at a distance of 20 cm from the end joined to the ring. (a) Assuming that the wave is reflected from the ends without loss of energy, find the time taken by the string to regain its shape. (b) The shape of the string changes periodically with time. Find this time period. (c) What is the tension in the string ?

View SolutionFigure shows a wave pulse at t=0. The pulse moves to the right with a speed of 10cms^-1. Sketch the shape of the string at t=1s, 2s and 3s.

View SolutionA wave pulse on a string on a string has the dimension shown in figure. The wave speed is v=1 cm /s . If point O is a free end. The shape of wave at time t = 3s si

View SolutionA simple harmonic oscillator at the point x=0 genrates a wave on a rope. The oscillator operates at a frequency of 40.0 Hz and with an amplitude of 3.00 cm. the rope has a linear mass density of 50.0g/m and is strectches with a tension of 5.00 N. (a) determine the speed of the wave. (b) find the wavelength. (c ) write the wave function y(x,t) for the wave, Assume that the oscillator has its maximum upward displacement at time t=0. (d) find the maximum transverse acceleration at time t=0. (d) find the maximum transverse of points on the rope. (e) in the discussion of transverse waves in this chapter, the force of gravity was ignored. is that a reasonable assumption for this wave? explain.
View SolutionAt t=0,a transverse wave pulse travelling in the positive x direction with a speed of 2m/s in a wire is described by the function y=6/x2 given that x≠0. Transverse velocity of a particle at x=2 m and t= 2 s is
View SolutionAt t=0,a transverse wave pulse travelling in the positive x direction with a speed of 2m/s in a wire is described by the function y=6/x2 given that x≠0. Transverse velocity of a particle at x=2 m and t= 2 s is
View Solution
