White light is incident normally on a glass plate of thickness 0.50×10−6 and index of refraction 1.50. Which wavelength in the visible region (400 nm - 700 nm) are strongly reflacted by the plate ?
To solve the problem of determining which wavelengths in the visible region (400 nm - 700 nm) are strongly reflected by a glass plate, we can follow these steps:
Step 1: Understand the Condition for Strong Reflection
For constructive interference (strong reflection) to occur, the path difference must satisfy the condition:
Δx=2t=(2n−1)λ2
where:
- t is the thickness of the glass plate,
- n is the order of interference,
- λ is the wavelength of light.
Step 2: Rearranging the Equation
From the above condition, we can rearrange it to find the wavelength:
λ=4t2n−1
Step 3: Substitute the Given Values
Given:
- Thickness t=0.50×10−6 m = 500 nm,
- Refractive index n=1.5.
Now we can substitute t into the equation:
λ=4×5002n−1
Step 4: Calculate Wavelengths for Different Orders
We will calculate the wavelengths for different values of n:
1. For n=1:
λ=20002×1−1=20001=2000 nm(Not in visible range)
2. For n=2:
λ=20002×2−1=20003≈666.67 nm(In visible range)
3. For n=3:
λ=20002×3−1=20005=400 nm(In visible range)
4. For n=4:
λ=20002×4−1=20007≈285.71 nm(Not in visible range)
Step 5: Conclusion
The wavelengths that are strongly reflected by the glass plate in the visible range are:
- 666.67 nm (approximately 667 nm)
- 400 nm
Thus, the answer is that the wavelengths strongly reflected by the plate are 400 nm and approximately 667 nm.
---
For constructive interference (strong reflection) to occur, the path difference must satisfy the condition:
Δx=2t=(2n−1)λ2
where:
- t is the thickness of the glass plate,
- n is the order of interference,
- λ is the wavelength of light.
From the above condition, we can rearrange it to find the wavelength:
λ=4t2n−1
Given:
- Thickness t=0.50×10−6 m = 500 nm,
- Refractive index n=1.5.
λ=4×5002n−1
We will calculate the wavelengths for different values of n:
λ=20002×1−1=20001=2000 nm(Not in visible range)
λ=20002×2−1=20003≈666.67 nm(In visible range)
λ=20002×3−1=20005=400 nm(In visible range)
λ=20002×4−1=20007≈285.71 nm(Not in visible range)
The wavelengths that are strongly reflected by the glass plate in the visible range are:
- 666.67 nm (approximately 667 nm)
- 400 nm
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Objective -117videos
LIGHT WAVES
- BOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Objective -210videos
LIGHT WAVES
- BOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer11videos
LIGHT WAVES
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer18videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective29videos
MAGNETIC FIELD
Similar Questions
White light is incident normally on a glass plate (in air) of thickness 500nm and refractive index of 1.5. The wavelength (in nm) in the visible region (400nm−700nm) that is strongly reflected by the plate is:
View SolutionA parallel beam of white light is incident normally on a water film1.0x10−4 cm thick. Find the wavelength in the visible range (400 nm-700 nm) which are strongly transmitted by the film Refractive index of water = 1.33.
View SolutionWhen white light is incident normally an oil film of thickness 10−4cm and refractive index. 1.4 then the wavelength which will not be seen in the reflected system of light is
View SolutionA glass surface is coated by an oil film of uniform thickness 1.00×10−4cm. The index of refraction of the oil is 1.25 and that of the glass is 1.50. Find the wavelengths of light in the visible region (400nm-750nm) which are completely transmitted by the oil film under normal incidence.
View SolutionA film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?
View SolutionLight is incident on a glass plate of refractive index 3.0 such that angle of refraction is 60° .Dark band is observed corresponding to the wavelength of 12000 A .If the thickness of glass plate is 1.2x10−3 mm. Calculate the order of the interference band for reflected system.
View SolutionLight is incident on a glass plate of refractive index 3.0 such that angle of refraction is 60° .Dark band is observed corresponding to the wavelength of 6000 A .If the thickness of glass plate is 1.2x10−3 mm. Calculate the order of the interference band for reflected system.
View SolutionA YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
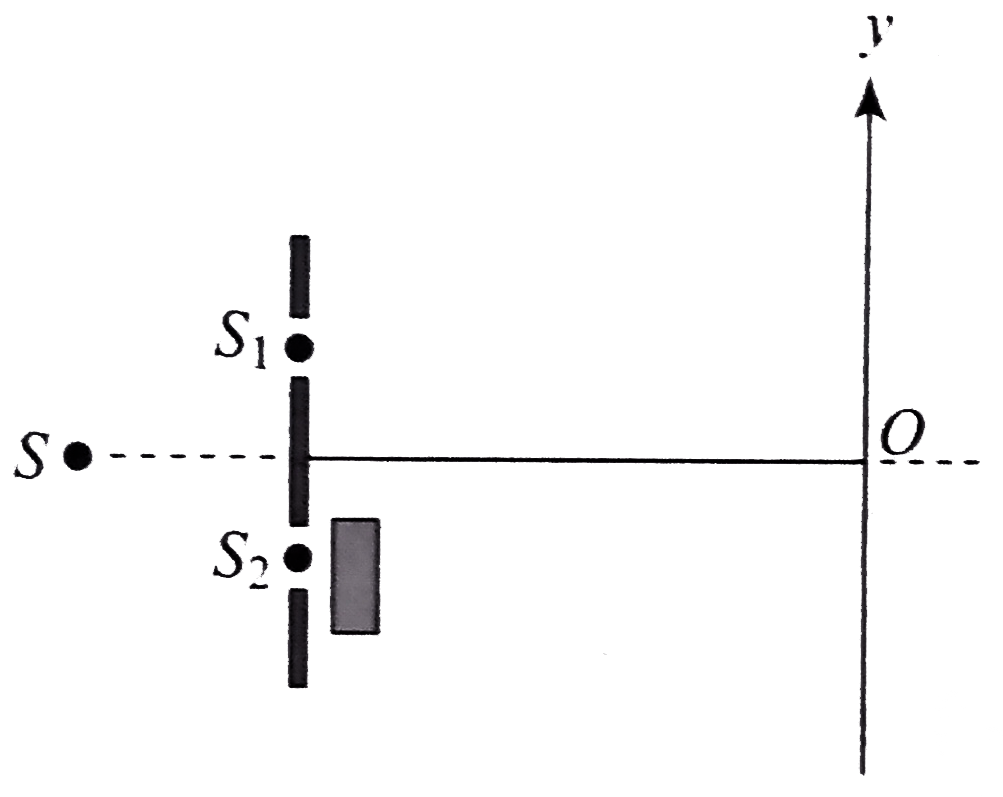
Now, if 600 nm, find the wavelength of the ligth that forms maximum exactly at point O.View SolutionA thin film of refractive index 1.5 and thickness 4×10−5 cm is illuminated by light normal to the surface. What wavelength within the visible spectrum will be intensified in the reflected beam?
View SolutionA YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
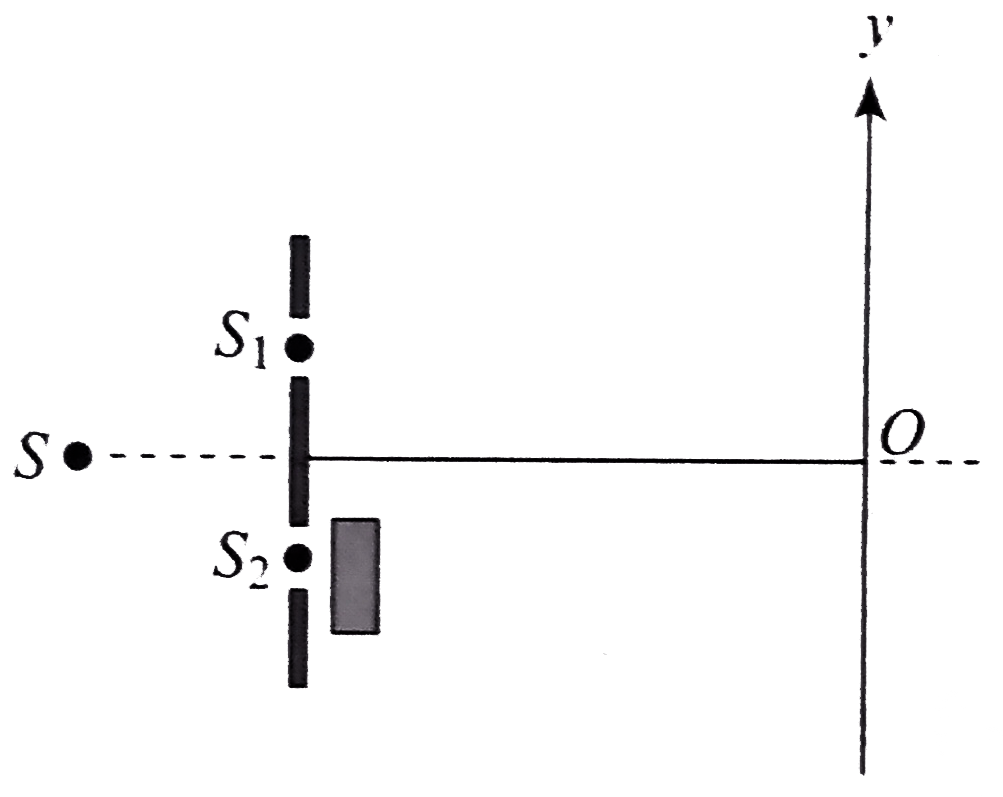
Find the light intensity at point O relative t maximum fringe intensity.View Solution

