A film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?
150 nm
200 nm
300 nm
450 nm
The correct Answer is:b
To find the minimum thickness of the thin film that will strongly reflect light with a wavelength of 600 nm, we can follow these steps:
Step 1: Understand the Condition for Strong Reflection
When light reflects off a thin film, constructive interference occurs when the path difference between the two reflected waves is an integer multiple of the wavelength. However, since the light reflects off a medium with a higher refractive index (from the film to the glass), there is a phase change of 180 degrees (or half a wavelength) for the wave reflecting from the top surface of the film.
Step 2: Determine the Effective Wavelength in the Film
The wavelength of light in the film is given by the formula:
λ′=λn
where:
- λ is the wavelength of light in vacuum (600 nm),
- n is the refractive index of the film (1.50).
Calculating the effective wavelength:
λ′=600nm1.50=400nm
Step 3: Set Up the Condition for Constructive Interference
For constructive interference in a thin film, the condition is given by:
2T=mλ′
where:
- T is the thickness of the film,
- m is the order of interference (an integer).
Since we want the minimum thickness, we set m=1:
2T=1⋅λ′
Substituting the effective wavelength:
2T=400nm
Step 4: Solve for the Thickness T
Now, we can solve for T:
T=400nm2=200nm
Conclusion
The minimum thickness of the thin film that will strongly reflect light with a wavelength of 600 nm is:
200nm
---
When light reflects off a thin film, constructive interference occurs when the path difference between the two reflected waves is an integer multiple of the wavelength. However, since the light reflects off a medium with a higher refractive index (from the film to the glass), there is a phase change of 180 degrees (or half a wavelength) for the wave reflecting from the top surface of the film.
The wavelength of light in the film is given by the formula:
λ′=λn
where:
- λ is the wavelength of light in vacuum (600 nm),
- n is the refractive index of the film (1.50).
λ′=600nm1.50=400nm
For constructive interference in a thin film, the condition is given by:
2T=mλ′
where:
- T is the thickness of the film,
- m is the order of interference (an integer).
2T=1⋅λ′
Substituting the effective wavelength:
2T=400nm
Now, we can solve for T:
T=400nm2=200nm
The minimum thickness of the thin film that will strongly reflect light with a wavelength of 600 nm is:
200nm
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Integer3videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Archives4videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning13videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type12videos
SOURCES OF MAGNETIC FIELD
Similar Questions
A thin film with index of refraction 1.33 coats a glass lens with index of refraction 1.50. Which of the following choices is the smallest film thickness that will not reflect light with wavelength 640 nm?
View SolutionA thin film of thickness t and index of refractive 1.33 coats a glass with index of refraction 1.50.What is the least thickness t that will strong reflect light with wavelength 600nm incident normally?
View SolutionHigh-quality camera lenses are often coated to prevent reflection. A lens has an optical index of refraction of 1.72 and a coating with an optical index os refraction 1.31. For near normal incidence, the minimum thickness of the coating to prevent reflection for wavelength of 5.3×10−7m is
View SolutionA parallel beam of light of wavelength 560 nm falls on a thin film of oil (refractive index = 1.4). What should be the minimum thickness of the film so that it strongly transmit the light ?
View SolutionA material having an index of refraction of 1.30 is used as an antireflective coating on a piece of glass (n = 1.50). What should be the minimum thickness of this film in order to minimize reflection of 500 nm light?
View SolutionA thin uniform film of refractive index 1.75 is placed on a sheet of glass of refractive index 1.5. At room temperature (20∘C) this film is just thick enoungh for light with wavelength 600 nm reflected off the top of the film to be canceled by light reflected from the top the glass. After the glass is placed in on oven and slowly heated to 170∘C, the film conceals reflected light with wavelength 606 nm. The coefficient of linear expansion of the film is (ignore any changes in the refractive index of the film due to the temperature change)
View SolutionRefractive index of a thin soap film of a uniform thickness is 1.34. Find the smallest thickness of the film that gives in interference maximum in the reflected light when light of wavelength 5360Å fall at normal incidence.
View SolutionA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
View SolutionRefractive index of a thin soap film of a uniform thickness is 1.38. The smallest thickness of the film that gives an interference maxima in the reflected light when light of wavelength 5520 Å falls at normal incidence is
View SolutionA ray of light is incident at an angle of 45∘ (ralative to the normal) on a thin film of a liquid with an index of refraction of √2. Assuming that the medium of both sides of the film is air, find the minimum thickness of the film required for constructive interference in the reflected light for sodium light of wavelenght 600 nm.
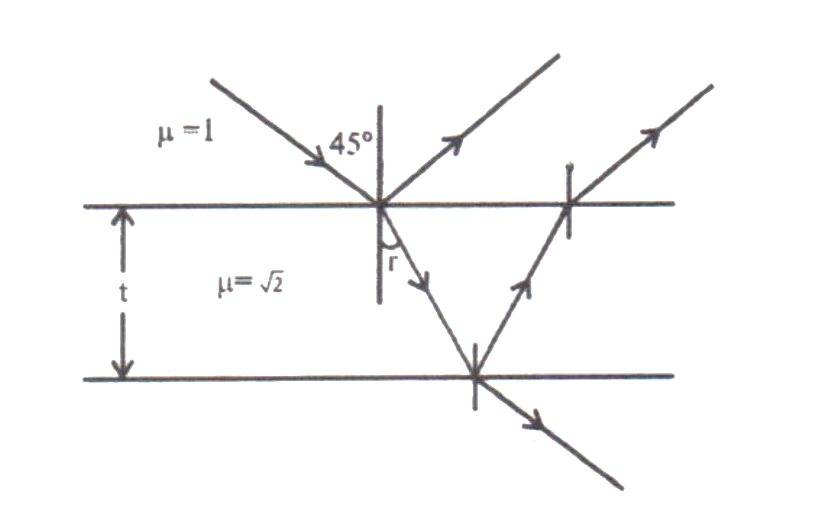 View Solution
View Solution

