A point source S is placed at a distance of 15 cm from a converging lens of focal length 10 cm on its principal axis. Where should a diverging miror of focal length 12 cm be placed to that a real image is formed on the source itself.
To solve the problem step by step, we will use the lens formula and the properties of mirrors.
Step 1: Identify the given values
- Distance of the point source (object distance from the lens), u=−15 cm (negative because it is on the same side as the incoming light).
- Focal length of the converging lens, f=10 cm.
- Focal length of the diverging mirror, fmirror=−12 cm (negative because it is a diverging mirror).
Step 2: Use the lens formula to find the image distance from the lens
The lens formula is given by:
1f=1v−1u
Where:
- f is the focal length of the lens,
- v is the image distance from the lens,
- u is the object distance from the lens.
Substituting the known values into the lens formula:
110=1v−1−15
This simplifies to:
110=1v+115
Step 3: Solve for 1v
To combine the fractions, we find a common denominator (30):
110=330and115=230
Thus, we rewrite the equation:
330=1v+230
Now, subtract 230 from both sides:
330−230=1v
This gives us:
130=1v
So, v=30 cm.
Step 4: Determine the position of the diverging mirror
The image formed by the lens is at a distance of 30 cm from the lens. For the real image to be formed at the source itself, the image formed by the diverging mirror must coincide with the position of the source (which is at -15 cm).
Step 5: Use the mirror formula to find the object distance for the mirror
The mirror formula is given by:
1fmirror=1vmirror−1umirror
Where:
- fmirror=−12 cm,
- vmirror is the image distance from the mirror,
- umirror is the object distance from the mirror.
Since the image formed by the lens (30 cm) is the object for the mirror, we have:
umirror=−30 cm (as it is on the same side as the incoming light)
Substituting into the mirror formula:
1−12=1vmirror−1−30
This simplifies to:
1−12=1vmirror+130
Step 6: Solve for vmirror
Rearranging gives:
1vmirror=1−12−130
Finding a common denominator (60):
1−12=−560and130=260
Thus:
1vmirror=−560−260=−760
So:
vmirror=−607≈−8.57 cm
Step 7: Find the distance between the lens and the mirror
The distance between the lens and the mirror is given by:
Distance=30 cm−8.57 cm≈21.43 cm
However, since the mirror is on the opposite side of the lens, we need to adjust this distance accordingly.
Final Answer
The diverging mirror should be placed approximately 6 cm from the lens on the opposite side.
---
- Distance of the point source (object distance from the lens), u=−15 cm (negative because it is on the same side as the incoming light).
- Focal length of the converging lens, f=10 cm.
- Focal length of the diverging mirror, fmirror=−12 cm (negative because it is a diverging mirror).
The lens formula is given by:
1f=1v−1u
Where:
- f is the focal length of the lens,
- v is the image distance from the lens,
- u is the object distance from the lens.
110=1v−1−15
This simplifies to:
110=1v+115
To combine the fractions, we find a common denominator (30):
110=330and115=230
Thus, we rewrite the equation:
330=1v+230
Now, subtract 230 from both sides:
330−230=1v
This gives us:
130=1v
So, v=30 cm.
The image formed by the lens is at a distance of 30 cm from the lens. For the real image to be formed at the source itself, the image formed by the diverging mirror must coincide with the position of the source (which is at -15 cm).
The mirror formula is given by:
1fmirror=1vmirror−1umirror
Where:
- fmirror=−12 cm,
- vmirror is the image distance from the mirror,
- umirror is the object distance from the mirror.
umirror=−30 cm (as it is on the same side as the incoming light)
1−12=1vmirror−1−30
This simplifies to:
1−12=1vmirror+130
Rearranging gives:
1vmirror=1−12−130
Finding a common denominator (60):
1−12=−560and130=260
Thus:
1vmirror=−560−260=−760
So:
vmirror=−607≈−8.57 cm
The distance between the lens and the mirror is given by:
Distance=30 cm−8.57 cm≈21.43 cm
However, since the mirror is on the opposite side of the lens, we need to adjust this distance accordingly.
The diverging mirror should be placed approximately 6 cm from the lens on the opposite side.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -1
15 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
An object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the image
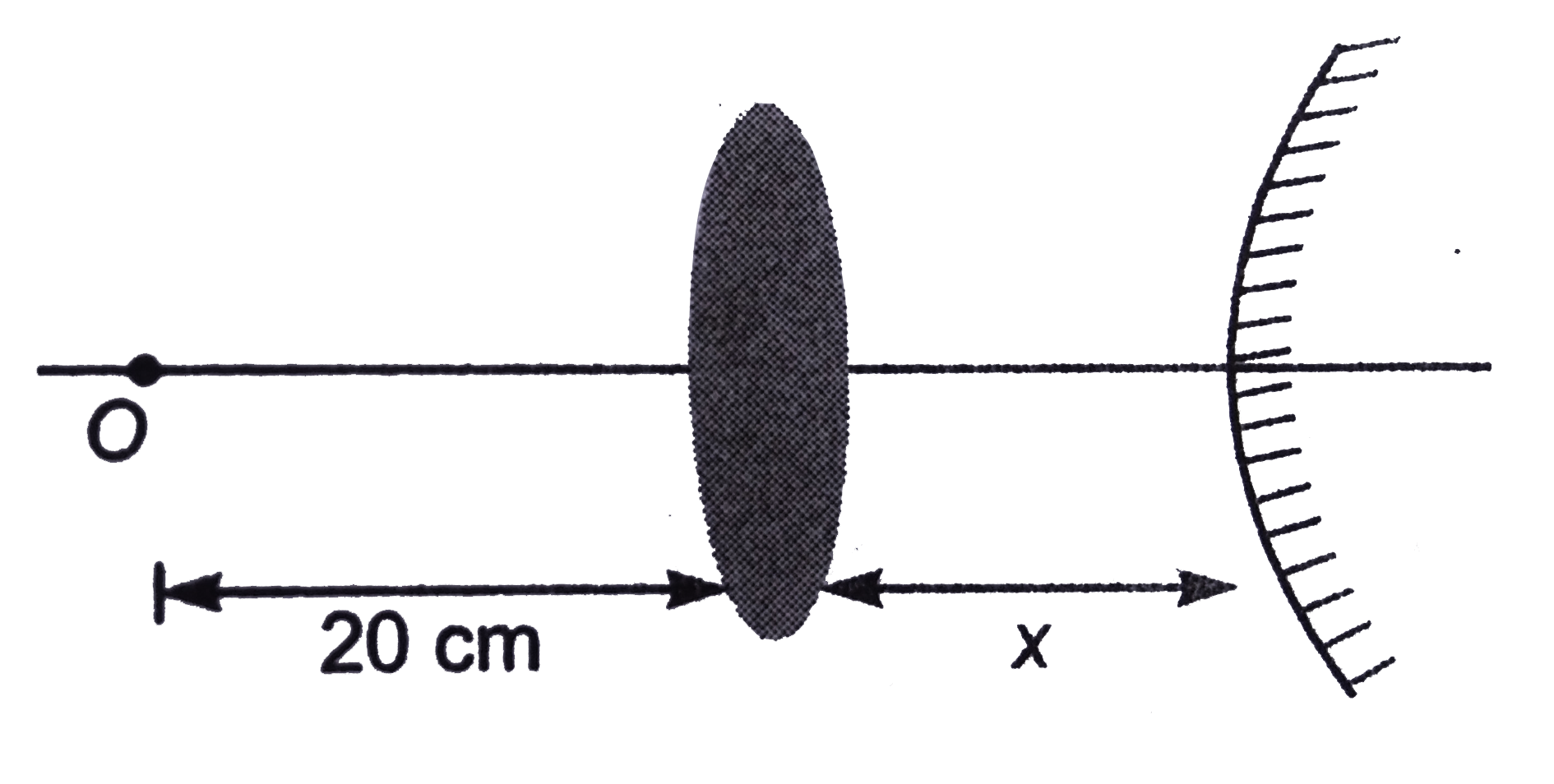
View SolutionA point object O is placed at a distance of 20cm from a convex lens of focal length 10cm as shown in the figure. At what distance x from the lens should a convex mirror of focal length 60cm, be placed so that final image coincide with the object?
View SolutionA point object O is placed at a distance of 20 cm from a convex lens of focal length 10 cm as shown in figure. At what distance x from the lens should a concave mirror of focal length 60 cm, placed so that final image coincides with the object ?

View SolutionA point object is placed at distance of 30 cm in front of a convex mirror of Focal length 30 cm. The image will form at
View SolutionA converging lens of focal length 40 cm is kept in contact with a diverging lens of focal length 30 cm. Find the focal length of the combination.
View SolutionA point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionA converging lens of focal length 5.0cm is placed in contact
with a diverging lens of focal length 10.0cm. Find the combined focal length of
the system.
View SolutionAn upright object is placed at a distance of 40cm in front of a convergent less of focal length 20cm. A convergent mirror of focal length 10cm is placed at a distance of 60cm on the other side of the lens. The position and size of the final image will be:
View SolutionA diverging lens of focal length 20 cm and a converging mirror of focal length 10 cm are placed coaxially at a separation of 5 cm. Where should an object be placed so that a real image is formed at the object itself ?
View Solution
