A slide projector produces 500 times enlarged image of a slide on a screen 10 m away. Assume that the projector consists of a single convex lens used for magnification. If the screen is moved 2.0 m closer, by what distance should the slide be moved towards or away from the lens so that the image remains focused on the screen ? What is the magnification in this case ?
To solve the problem step by step, we will follow the principles of optics, particularly using the lens formula and the magnification formula.
Step 1: Identify the given values
- Initial magnification (M) = 500
- Initial image distance (V) = 10 m
- The screen is moved 2 m closer.
Step 2: Calculate the initial object distance (U)
Using the magnification formula:
M=VU
We can rearrange this to find U:
U=VM
Substituting the known values:
U=10m500=0.02m
Step 3: Use the lens formula to find the focal length (f)
The lens formula is given by:
1f=1V−1U
Substituting the values we have:
1f=110−10.02
Calculating:
1f=110−50=1−50010=−49910
Thus,
f=−10499≈−0.02004m
Step 4: Calculate the new image distance (V')
Since the screen is moved 2 m closer:
V′=10m−2m=8m
Step 5: Find the new object distance (U')
Using the lens formula again:
1f=1V′−1U′
Rearranging gives:
1U′=1V′−1f
Substituting the known values:
1U′=18−1−0.02004
Calculating:
1U′=18+49.9
1U′=0.125+49.9=50.025
Thus,
U′=150.025≈0.01999m
Step 6: Determine the distance the slide should be moved
The initial object distance was 0.02 m, and the new object distance is approximately 0.01999 m. The distance the slide should be moved is:
ΔU=U′−U=0.01999m−0.02m=−0.00001m
This means the slide should be moved approximately 0.00001 m (or 0.01 mm) towards the lens.
Step 7: Calculate the new magnification (M')
Using the magnification formula:
M′=V′U′
Substituting the new values:
M′=8m0.01999m≈400
Summary of the Results
- The slide should be moved approximately 0.00001 m (0.01 mm) towards the lens.
- The new magnification is approximately 400.
- Initial magnification (M) = 500
- Initial image distance (V) = 10 m
- The screen is moved 2 m closer.
Using the magnification formula:
M=VU
We can rearrange this to find U:
U=VM
Substituting the known values:
U=10m500=0.02m
The lens formula is given by:
1f=1V−1U
Substituting the values we have:
1f=110−10.02
Calculating:
1f=110−50=1−50010=−49910
Thus,
f=−10499≈−0.02004m
Since the screen is moved 2 m closer:
V′=10m−2m=8m
Using the lens formula again:
1f=1V′−1U′
Rearranging gives:
1U′=1V′−1f
Substituting the known values:
1U′=18−1−0.02004
Calculating:
1U′=18+49.9
1U′=0.125+49.9=50.025
Thus,
U′=150.025≈0.01999m
The initial object distance was 0.02 m, and the new object distance is approximately 0.01999 m. The distance the slide should be moved is:
ΔU=U′−U=0.01999m−0.02m=−0.00001m
This means the slide should be moved approximately 0.00001 m (or 0.01 mm) towards the lens.
Using the magnification formula:
M′=V′U′
Substituting the new values:
M′=8m0.01999m≈400
- The slide should be moved approximately 0.00001 m (0.01 mm) towards the lens.
- The new magnification is approximately 400.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -1
15 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
A concave lens has focal length of 15 cm. At what distance should an object from the lens be placed so that it forms an image at 10 cm from the lens ? Also, find the magnification of the lens.
View SolutionA convex lens forms an image of an object on a screen 30 cm from the lens. When the lens is moved 90 cm towards the object, then the image is again formed on the screen. Then, the focal length of the lens is
View Solution
Knowledge Check
An object is place 20 cm from a diverging lens. If the distance between the lens and the image is 8 cm, what is the magnification?
A115B25C12D2
(i) A diverging lens of focal length 15 cm, forms an image at distance of 10 cm from the lens. How far is the object placed from the lens ?
(ii) What is the linear magnification of the image?
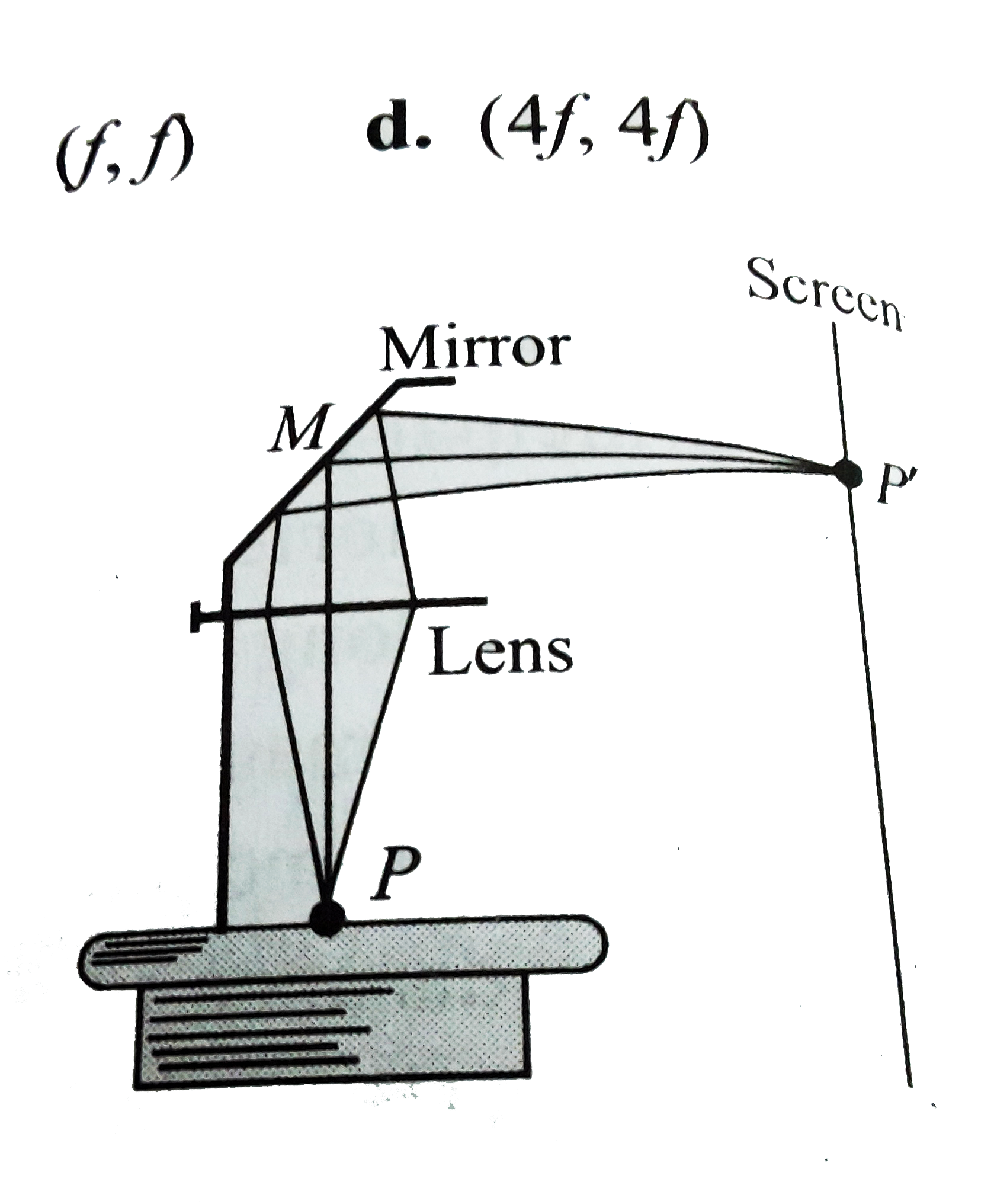
View SolutionThe lens in an overhead projector forms an image P′ of a point P on an overhead tranparency, If the screen is moved closer to the projector to keep the image on the screen in focus, the lens must be
View SolutionA convex lens produces an image of a real object on a screen with a magnification of 1/2. When the lens is moved 30 cm away from the object, the magnification of the image on the screen is 2. The focal length of the lens is
View SolutionThe photograph of a house occupies an area of 1.7cm2 on a 35 slide. The slide is projected on to a screen, and the area of the house on the screen is 1.55m2 What is the liner magnification of the projector screen arrangement?
View SolutionA convex lens is used to obtain a magnified image of an object on a screen . The object is at a distance 10 m from the lens . If the magnification is 19. the focal length of the lens is
View SolutionA convex lens forms an image of an object placed 20cm away from it at a distance of 20cm on the other side of the lens. If the object is moves 5cm toward the lens, the image will be
View SolutionThe magnification of an image by a convex lens is positive only when the object is placed
View Solution
