A 5.0 diopter lens forms a virtual image which is 4 times the object placed perpendicularly on the principal axis of the lens. Find the distance of the object from the lens.
The correct Answer is:A, C
To solve the problem step by step, we will use the lens formula and the magnification formula.
Step 1: Understand the given information
- The power of the lens (P) = 5.0 diopters
- The magnification (m) = -4 (since the image is virtual and upright)
- We need to find the object distance (u).
Step 2: Calculate the focal length (f) of the lens
The focal length (f) can be calculated using the formula:
f=1P
Given that P = 5.0 diopters, we convert this to focal length in centimeters:
f=1005=20 cm
Step 3: Relate the magnification to object and image distances
The magnification (m) is given by the formula:
m=vu
Where:
- v = image distance
- u = object distance
Since the magnification is -4, we can write:
−4=vu
This implies:
v=−4u
Step 4: Use the lens formula
The lens formula is given by:
1f=1v−1u
Substituting the values we have:
120=1−4u−1u
Step 5: Simplify the equation
We can rewrite the right side:
120=−14u−1u
To combine the fractions on the right, we find a common denominator:
120=−14u−44u=−54u
Now we have:
120=−54u
Step 6: Cross-multiply to solve for u
Cross-multiplying gives:
1⋅4u=−5⋅20
This simplifies to:
4u=−100
Therefore:
u=−1004=−25 cm
Step 7: Conclusion
The distance of the object from the lens is u=−25 cm. The negative sign indicates that the object is placed on the same side as the incoming light, which is typical for real objects.
- The power of the lens (P) = 5.0 diopters
- The magnification (m) = -4 (since the image is virtual and upright)
- We need to find the object distance (u).
The focal length (f) can be calculated using the formula:
f=1P
Given that P = 5.0 diopters, we convert this to focal length in centimeters:
f=1005=20 cm
The magnification (m) is given by the formula:
m=vu
Where:
- v = image distance
- u = object distance
−4=vu
This implies:
v=−4u
The lens formula is given by:
1f=1v−1u
Substituting the values we have:
120=1−4u−1u
We can rewrite the right side:
120=−14u−1u
To combine the fractions on the right, we find a common denominator:
120=−14u−44u=−54u
Now we have:
120=−54u
Cross-multiplying gives:
1⋅4u=−5⋅20
This simplifies to:
4u=−100
Therefore:
u=−1004=−25 cm
The distance of the object from the lens is u=−25 cm. The negative sign indicates that the object is placed on the same side as the incoming light, which is typical for real objects.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXAMPLE9videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer18videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -27videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question7videos
GAUSS LAW
- BOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer11videos
LIGHT WAVES
Similar Questions
A lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find the distance of the object from the lens.
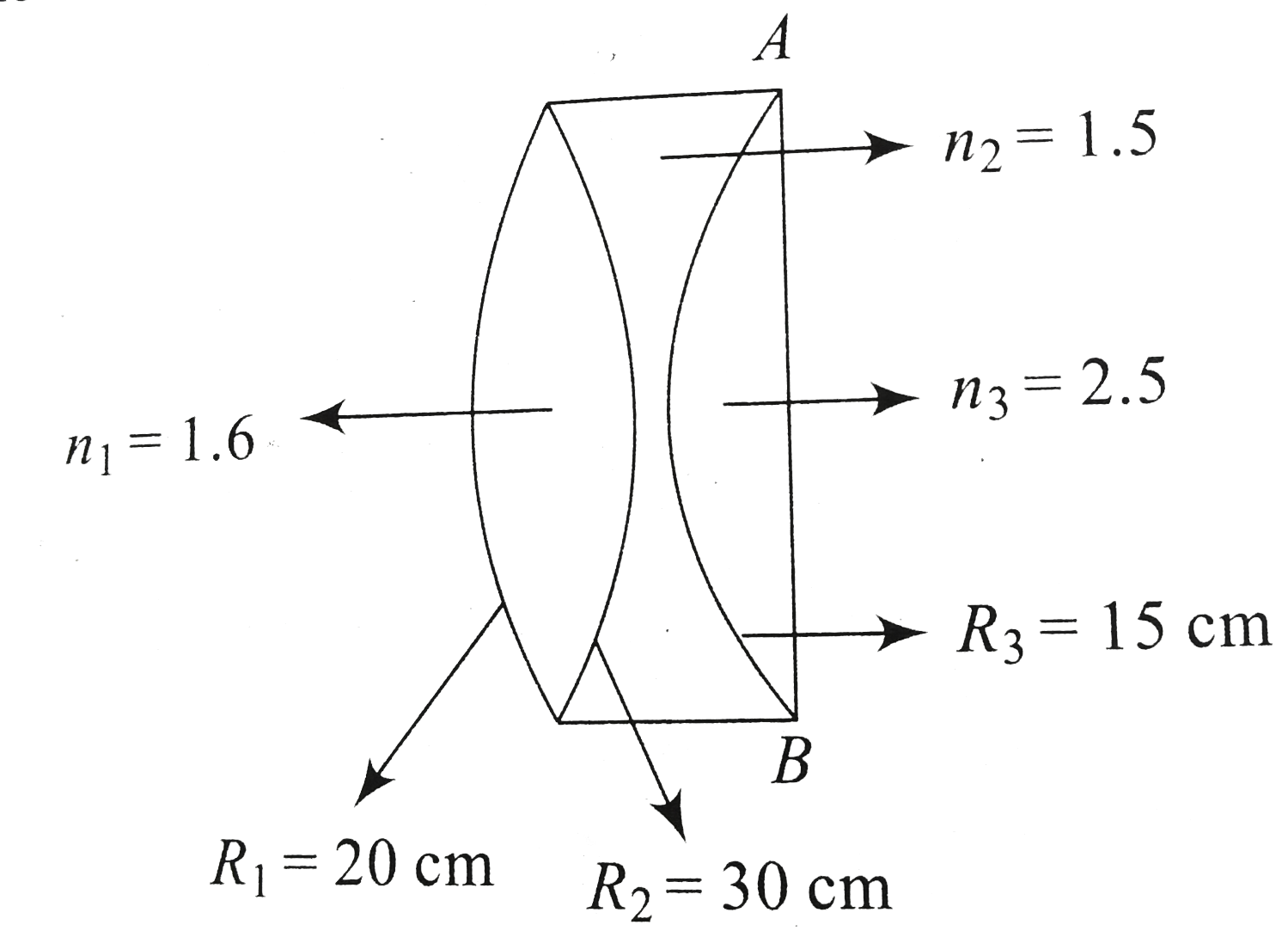 View Solution
View SolutionA 2.0cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 10 cm. The distance of the object from the lens is 15 cm. Find the nature, position and size of the image. Also, find its magnification.
View Solution
Knowledge Check
- Question 1 - Select One
A virtual, diminished image is formed when an object is placed between the optical centre and the principal focus of a lens.
Name the type of lens which forms the above image.AconvexBconcaveCbothDnone
One half of a convex lens of focal length 10 cm is covered with a black paper. Can such
a lens produce an image of a complete object placed at a distance of 30 cm from the
lens? Draw a ray diagram to justify your answer. A 4 can tall object is placed
perpendicular to the perpendicular to the principal axis of a convex lens of focal length 20 cm. The distince
of the object from the less is 15 cm. Find nature, position and size of the image.View SolutionA convex forms a real image of a poinnt object placed on its principal axis. If the upper half of the lens is painted black
View SolutionA 1.5 cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 15 cm. Distance of object from the lens is 20 cm. Use lens formula to find the nature, position and size of the image.
View SolutionA convex lens produces a real image m times the size of the object. What will be the distance of the object from the lens ?
View SolutionAn object of length 2.0 cm is placed perpendicular to the principal axis of a convex lens of focal length 12 cm. Find the size of the image of the object is at a distance of 8.0 cm from the lens.
View SolutionA lens produces a virtual image between the object and the lens.
Name the lens.View SolutionAn extended object of size 2mm is placed on the principal axis of a converging lens of focal length 10cm. It is found that when the object is placed perpendicular to the principal axis the image formed is 4mm in size. The size of image when it is placed along the principal axis is ____________ mm.
View Solution

