The separation between the plates of a charged parallel-plate capacitor is increased. Which of the following quantities will change?
Charge on the capacitor
potential difference across the capacitor
Energy of the capacitor
Energy density between the plates
The correct Answer is:B, C
To solve the question regarding the changes in quantities when the separation between the plates of a charged parallel-plate capacitor is increased, we can analyze each quantity step by step.
1. Understanding Charge on the Capacitor:
- The charge Q on a capacitor is conserved for an isolated capacitor. This means that if the capacitor is disconnected from any voltage source, the charge will remain constant regardless of the distance between the plates.
- Conclusion: The charge on the capacitor will not change.
2. Analyzing Potential Difference (Voltage):
- The capacitance C of a parallel-plate capacitor is given by the formula:
C=K⋅A⋅ϵ0D
where K is the dielectric constant, A is the area of the plates, ϵ0 is the permittivity of free space, and D is the separation between the plates.
- As the separation D increases, the capacitance C decreases.
- The relationship between charge, capacitance, and potential difference is given by:
V=QC
- Since Q is constant and C decreases, it follows that V must increase.
- Conclusion: The potential difference across the capacitor will change.
3. Calculating Energy of the Capacitor:
- The energy E stored in a capacitor can be expressed as:
E=12CV2
- Since we established that C decreases and V increases, we need to analyze how these changes affect energy.
- Given that V increases more significantly than C decreases (since V is proportional to 1C), the overall energy stored in the capacitor will increase.
- Conclusion: The energy of the capacitor will change.
4. Examining Energy Density:
- The energy density u between the plates of a capacitor is given by:
u=12ϵ0E2
- The electric field E between the plates of a parallel-plate capacitor is given by:
E=VD
- Although D increases, the relationship between V and E indicates that the electric field remains constant for a charged capacitor since the charge remains the same.
- Therefore, the energy density, which depends on the electric field, will also remain constant.
- Conclusion: The energy density between the plates will not change.
Final Answer:
- The quantities that will change are:
- Potential Difference (V): Will change.
- Energy (E): Will change.
- The quantities that will not change are:
- Charge (Q): Will not change.
- Energy Density (u): Will not change.
- The charge Q on a capacitor is conserved for an isolated capacitor. This means that if the capacitor is disconnected from any voltage source, the charge will remain constant regardless of the distance between the plates.
- Conclusion: The charge on the capacitor will not change.
- The capacitance C of a parallel-plate capacitor is given by the formula:
C=K⋅A⋅ϵ0D
where K is the dielectric constant, A is the area of the plates, ϵ0 is the permittivity of free space, and D is the separation between the plates.
- As the separation D increases, the capacitance C decreases.
- The relationship between charge, capacitance, and potential difference is given by:
V=QC
- Since Q is constant and C decreases, it follows that V must increase.
- Conclusion: The potential difference across the capacitor will change.
- The energy E stored in a capacitor can be expressed as:
E=12CV2
- Since we established that C decreases and V increases, we need to analyze how these changes affect energy.
- Given that V increases more significantly than C decreases (since V is proportional to 1C), the overall energy stored in the capacitor will increase.
- Conclusion: The energy of the capacitor will change.
- The energy density u between the plates of a capacitor is given by:
u=12ϵ0E2
- The electric field E between the plates of a parallel-plate capacitor is given by:
E=VD
- Although D increases, the relationship between V and E indicates that the electric field remains constant for a charged capacitor since the charge remains the same.
- Therefore, the energy density, which depends on the electric field, will also remain constant.
- Conclusion: The energy density between the plates will not change.
- The quantities that will change are:
- Potential Difference (V): Will change.
- Energy (E): Will change.
- The quantities that will not change are:
- Charge (Q): Will not change.
- Energy Density (u): Will not change.
Topper's Solved these Questions
- CAPACITORSBOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - Exercise
65 Videos
- CAPACITORSBOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - short answer
7 Videos
- CAPACITORSBOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - Objective 1
12 Videos
- BOHR'S MODEL AND PHYSICS OF THE ATOMBOOK - HC VERMA ENGLISHCHAPTER - BOHR'S MODEL AND PHYSICS OF THE ATOMEXERCISE - Short Answer
10 Videos
- DISPERSION AND SPECTRABOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer
6 Videos
Similar Questions
Knowledge Check
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.In a parallel plate capacitor , the capacity increases if
Aarea of the plate is decreasedBdistance between the plates increasesCarea of the plate is increasedDdielectric constant decreases.
A dielectric slab is inserted between the plates of an isolatted charged capacitor. Which of the following quantities will remain the same?
View SolutionThe separation between the plates of a parallel-plate capacitor is 2 mm and the area of its plates is 5cm2. If the capacitor is charged such that it has 0.01J energy stored in it, the electrostatic force of attraction between its plates is _______N.
View SolutionYou have a parallel plate capacitor, a spherical capacitor and a cylindrical capacitor. Each capacitor is charged and then removed from the same battery. Consider the following situations :
I:· Separation between the plates of parallel plate capacitor is reduced
II: Radius of the outer spherical shell of the spherical capacitor is increased
Ill: Radius of the outer cylinder of cylindrical capacitor is increased.
Which of the following is correct?
View SolutionA parallel plate capacitor is charged. If the plates are pulled apart
View SolutionThe force between the plates of a parallel plate capacitor of capacitance C and distance of separation of the plates d with a potential difference V between the plates, is.
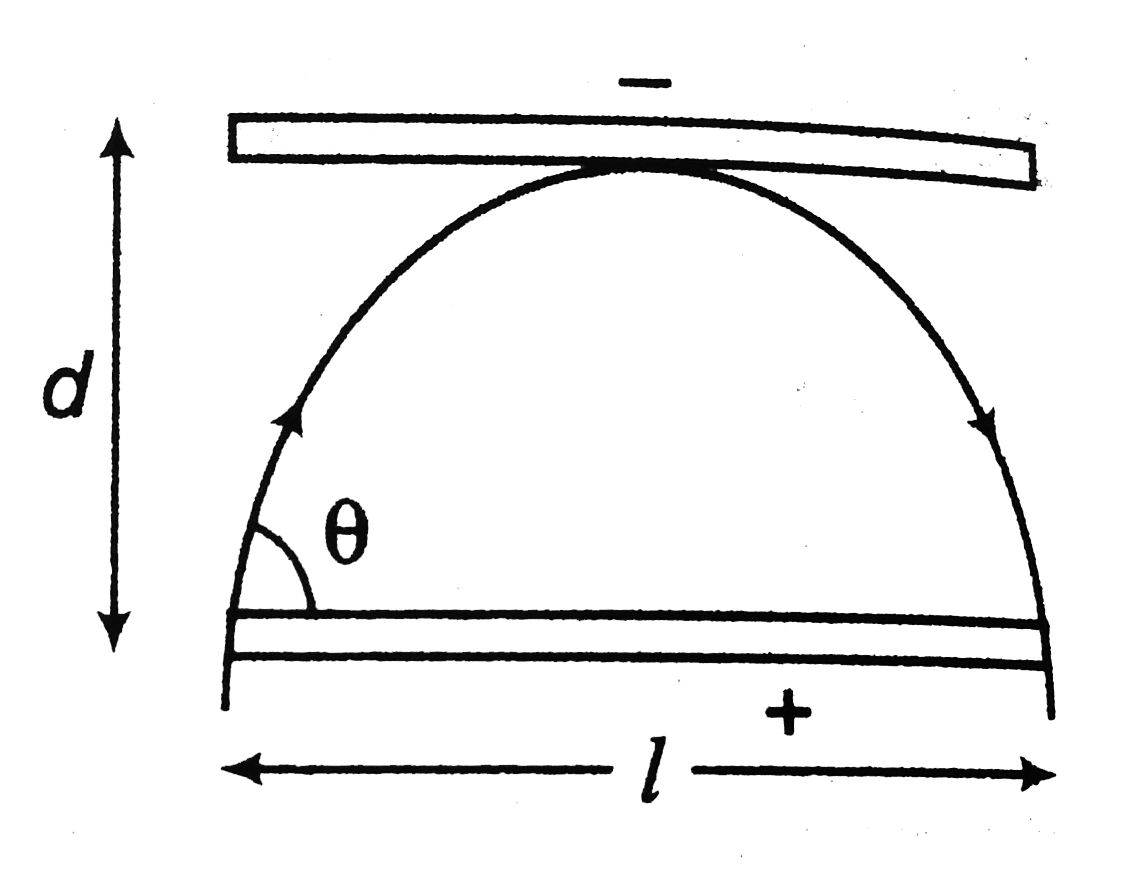
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at an angle θ to the plates. The plate width is l, the plate separation is d. The electron follows the path shown just missing the upper plate. Neglect gravity. Then,
View Solution
