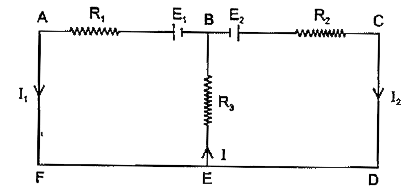
Find the currents going through the three resistors R1,R2 and R3 in the circuit of figure.

Lets us take the potential of the point A to be zero. The potential at C will be (epsilon)_(1)and that at D will be (ε)2.Let the potential at B be V . The currents through the three resistors are i1,i2andi1+i2as shown in figure. Note that the current directed towards B equals the current directed away from B.
Applying Ohm's law to the three resistors R1,R2andR3,
we get,
(ε)1−V=R1i1...(i)
(ε)2−V=R2i2...(ii)
andV−0=R3(i1+I2)....(iii)
Adding (i) and (iii),
(ε)1=R1i1+R3(i1+i2)
=(R1+R3)i1+R3i2...(iv)
andadd∈g(ii)and(iii)
(ε)2=R2i2+R3(i1+I2)
=(R2+R3)i2+R3i1....(v)
Equations (iv) and (v) may be directly written from Kirchhoff's loop applied to the left half and the right half of the circuit.
Multiply (iv)by (R2+R3),(v)byR3and⊂tract→elim∈atei2.This gives
i1=((ε1(R2+R3)−ε2R3R1+R3(R2+R3)−R23).
(ε1)(R2+R3)−(ε2)R3R1R2+R2R3+R3R1.
Similarly, eliminating i1from (iv) and (v) we obtain,
i2=(ε2)(R1+R3)−(ε1)R3R1R2+R2R3+R3R1
Andso
i1+i2=((ε1)R2+ε2R1R1R2+R2R3+R3R1.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Objective 115videos
ELECTRIC CURRENT IN CONDUCTORS
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - objective 29videos
ELECTRIC CURRENT IN CONDUCTORS
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - questions for short answer17videos
ELECTRIC CURRENT IN CONDUCTORS
- BOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer6videos
DISPERSION AND SPECTRA
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer6videos
ELECTRIC CURRENT THROUGH GASES
Similar Questions
In the circuit shown below, E1=17V,E2=21V,R1=2Ω,R2=3ΩandR3=5Ω. Using Kirchhoff.s laws, find the currents flowing through the resistors R1,R2 and R3. (Internal resistance of each of the batteries is negligible.)
 View Solution
View SolutionFind the current through resistor R.
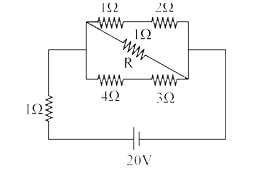 View Solution
View SolutionFind the currents through the three resistors shown in Figure
 View Solution
View SolutionFind the current through resistor R
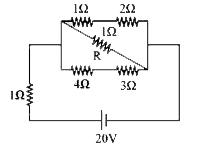 View Solution
View SolutionFind RAB in the circuit shown in figure.
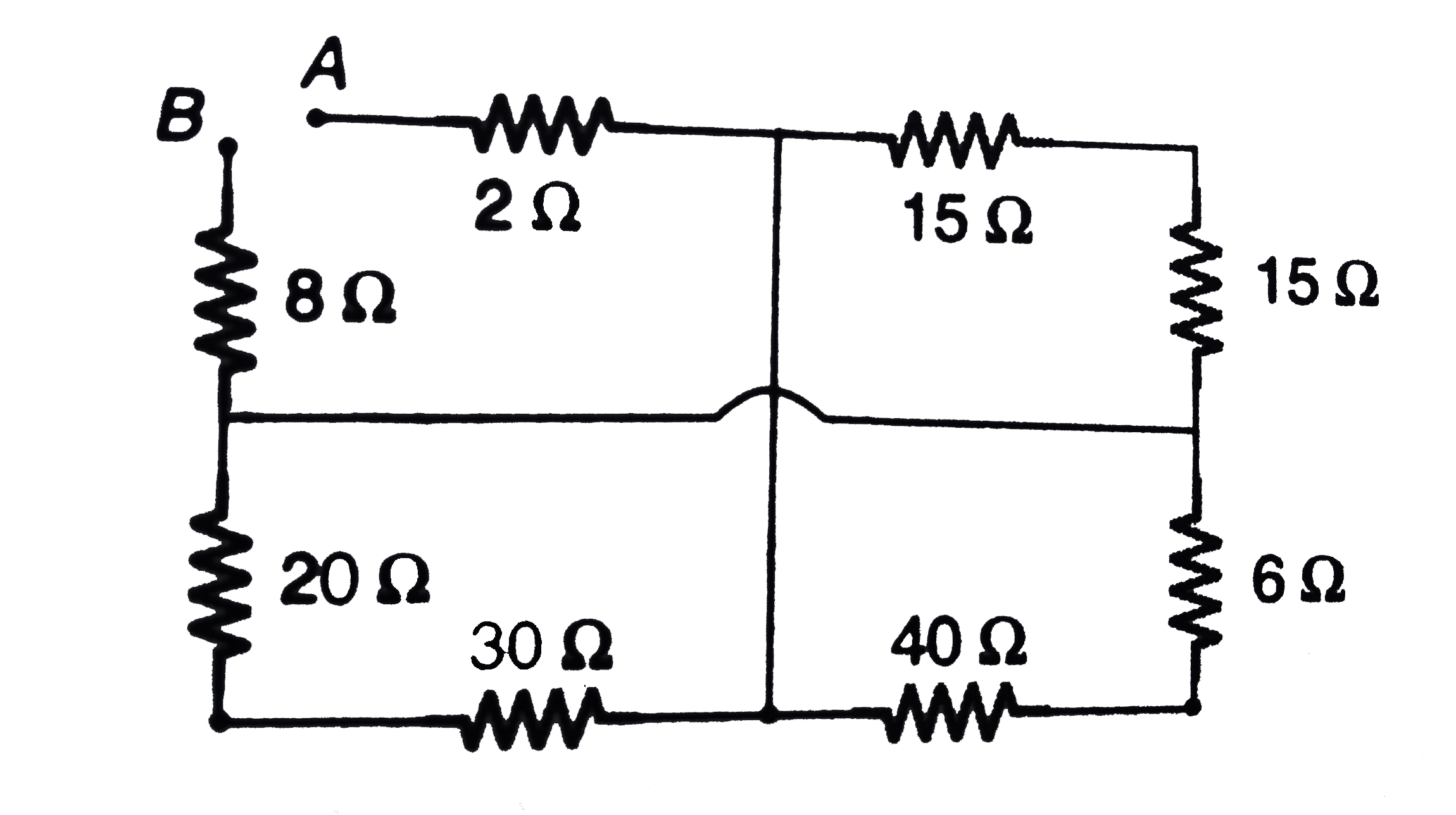 View Solution
View SolutionThe circuit is shown in the following figure. The potential at points A, B, C, D and O are given. The currents in the resistance R1,R2 and R3 are in the ratio of 4:2:1. What is the ratio of resistance R1,R2,R3 and R4 ?
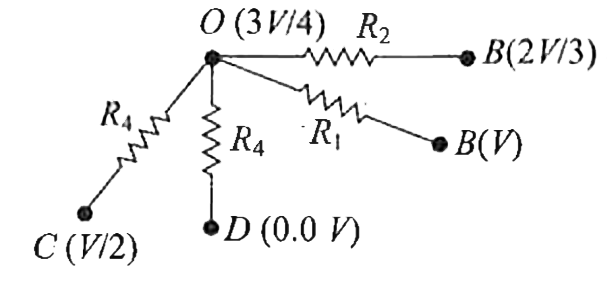 View Solution
View SolutionThe current in the resistor R3 in the circuit as shown in the figure is
 View Solution
View SolutionFind the current through 4Ω resistor in the circuit shown in figure.
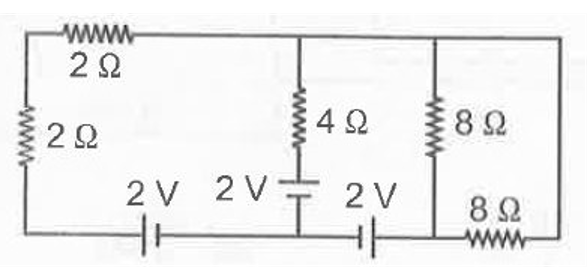 View Solution
View SolutionIn the circuit diagram given below, three resistors R1,R2, and R3 of 5Ω,10Ω and 30Ω, respectively are connected as shown.
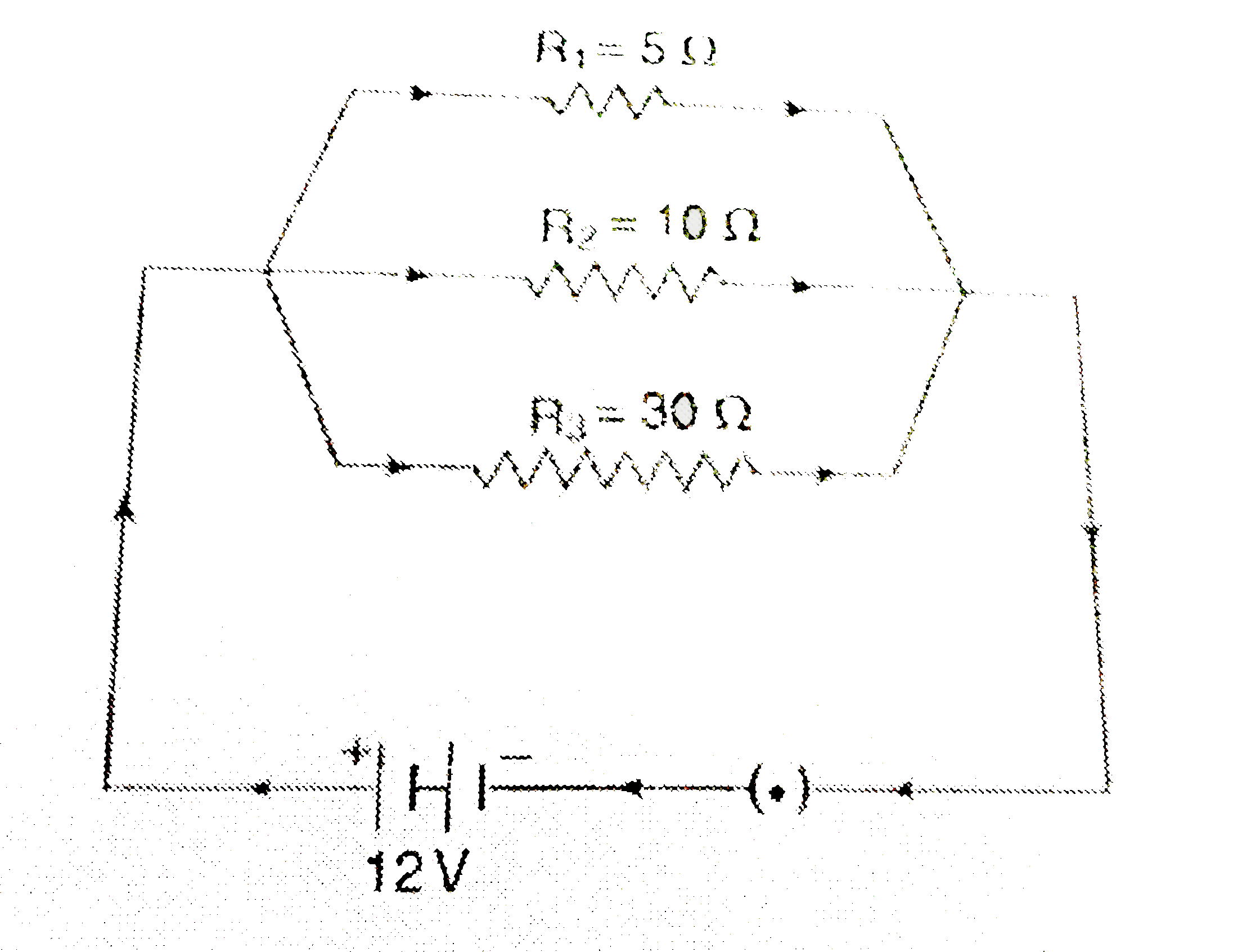
Calculate:
(a) current through each resistor.
(b) total current in the circuit.
(c) total resistance in the circuit.View SolutionFigure shows an L-R circuit. When the switch S is closed, the current through resistor R1,R2 and l3 are l1,l2 and l3 respectively. The value of l1,l2 and l3 at t=0 s is
View Solution

