A long, straight wire carries a current i. Let B_1 be the magnetic field at a point P at a distance d from the wire. Consider a section of length l of this wire such that the point P lies on a perpendicular bisector of the section. Let B_2 be the magnetic field at this point due to this section only. Find the value of d/l so that B_2 differs from B_1 by 1 %.
To solve the problem, we need to find the ratio dl such that the magnetic field B2 at point P due to a section of length l of the wire differs from the magnetic field B1 at point P due to the entire wire by 1%.
1. Magnetic Field Due to a Long Straight Wire:
The magnetic field B1 at a distance d from a long straight wire carrying a current I is given by:
B1=μ0I2πd
2. Magnetic Field Due to a Finite Section of Wire:
For a section of length l of the wire, where point P lies on the perpendicular bisector of the section, the magnetic field B2 can be calculated using the formula:
B2=μ0I4π⋅l√(l2)2+d2
Here, the distance from the midpoint of the section to point P is √(l2)2+d2.
3. Setting Up the Condition for 1% Difference:
We want B2 to differ from B1 by 1%. This can be expressed mathematically as:
B1−B2B1=0.01
This implies:
B2=0.99B1
4. Substituting the Expressions for B1 and B2:
Substitute the expressions for B1 and B2 into the equation:
μ0I4π⋅l√(l2)2+d2=0.99⋅μ0I2πd
5. Canceling Common Terms:
Cancel out μ0I4π from both sides:
l√(l2)2+d2=0.99⋅2d1
Simplifying gives:
l=1.98d√(l2)2+d2
6. Squaring Both Sides:
Square both sides to eliminate the square root:
l2=(1.982d2)(l24+d2)
7. Expanding and Rearranging:
Expanding the right side:
l2=3.9204d2⋅l24+3.9204d4
Rearranging gives:
l2−0.9801d2l2=3.9204d4
l2(1−0.9801d2)=3.9204d4
8. Finding the Ratio dl:
Let x=dl, then d=xl. Substitute d in terms of l:
l2(1−0.9801x2)=3.9204x4l4
Dividing both sides by l2:
1−0.9801x2=3.9204x4l2
Rearranging gives:
3.9204x4l2+0.9801x2−1=0
9. Solving the Quadratic Equation:
This is a quadratic in x2. Using the quadratic formula:
x2=−b±√b2−4ac2a
where a=3.9204l2,b=0.9801,c=−1.
10. Calculating the Ratio:
After solving, we find:
dl≈0.07
Final Result:
Thus, the value of dl such that B2 differs from B1 by 1% is approximately:
dl≈0.07
The magnetic field B1 at a distance d from a long straight wire carrying a current I is given by:
B1=μ0I2πd
For a section of length l of the wire, where point P lies on the perpendicular bisector of the section, the magnetic field B2 can be calculated using the formula:
B2=μ0I4π⋅l√(l2)2+d2
Here, the distance from the midpoint of the section to point P is √(l2)2+d2.
We want B2 to differ from B1 by 1%. This can be expressed mathematically as:
B1−B2B1=0.01
This implies:
B2=0.99B1
Substitute the expressions for B1 and B2 into the equation:
μ0I4π⋅l√(l2)2+d2=0.99⋅μ0I2πd
Cancel out μ0I4π from both sides:
l√(l2)2+d2=0.99⋅2d1
Simplifying gives:
l=1.98d√(l2)2+d2
Square both sides to eliminate the square root:
l2=(1.982d2)(l24+d2)
Expanding the right side:
l2=3.9204d2⋅l24+3.9204d4
Rearranging gives:
l2−0.9801d2l2=3.9204d4
l2(1−0.9801d2)=3.9204d4
Let x=dl, then d=xl. Substitute d in terms of l:
l2(1−0.9801x2)=3.9204x4l4
Dividing both sides by l2:
1−0.9801x2=3.9204x4l2
Rearranging gives:
3.9204x4l2+0.9801x2−1=0
This is a quadratic in x2. Using the quadratic formula:
x2=−b±√b2−4ac2a
where a=3.9204l2,b=0.9801,c=−1.
After solving, we find:
dl≈0.07
Thus, the value of dl such that B2 differs from B1 by 1% is approximately:
dl≈0.07
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - questions for short answer12videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 27videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective29videos
MAGNETIC FIELD
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Short Answer6videos
MAGNETIC PROPERTIES OF MATTER
Similar Questions
A long straight wire carries a current of 35A. What is the magnitude of the field →B at a point 20cm from the wire?
View SolutionA long, straight wire carries a current i. The magnetizing field intensity H is measured at a point P close to the wire. A long, cylindrical iron rod is brought close to the wire so that the point P is at the centre of the rod. The value of H at P will
View Solution
Knowledge Check
- Question 1 - Select One
If a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
Magnetic field at a distance a from long current carrying wire is proportional to
View SolutionA wire of infinite length bent as shown in figure carries current l. What is the magnetic field at the point P ?
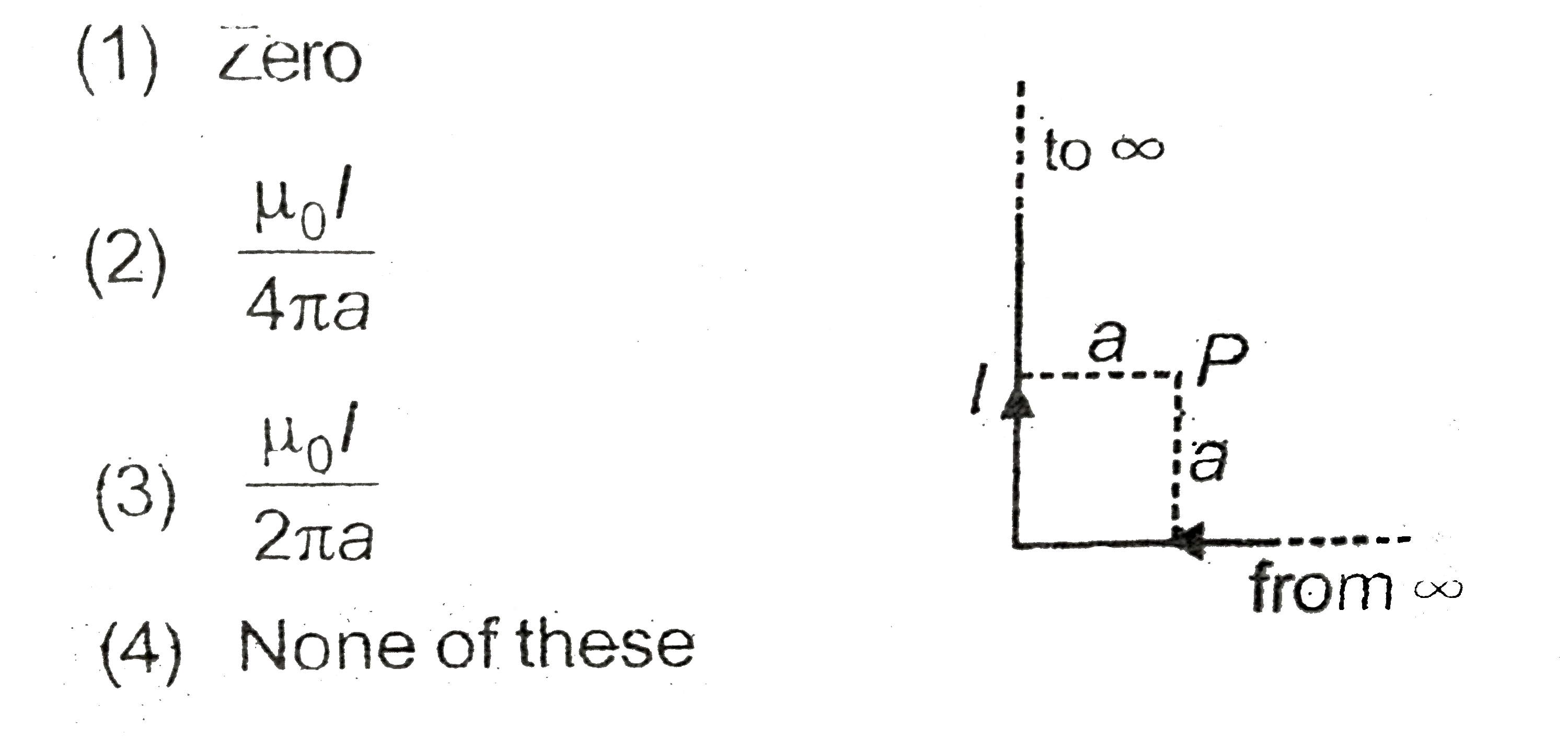 View Solution
View SolutionFind magnetic field at point P shown in Fig. point P is on the bisector of angle between the wires.
View SolutionDetermine the magnetic field at point P located a distance x from the corner of an infinitely long 2. Wire bent at right angle as shown in figure. The wire carries a steady current i.
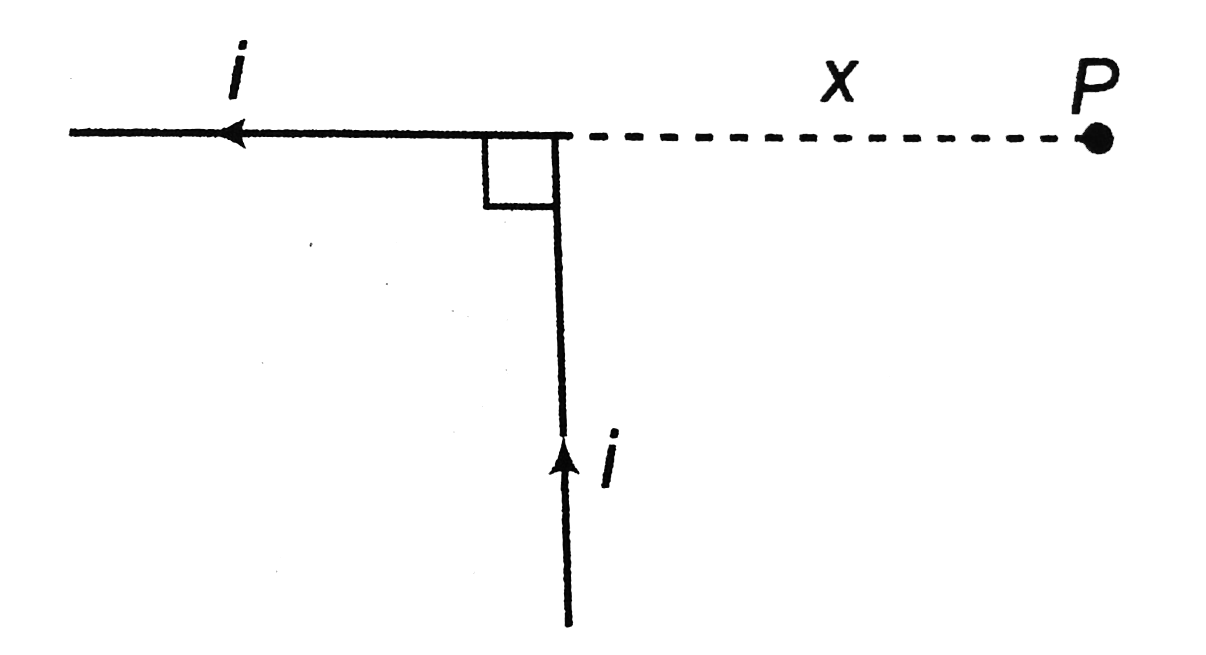 View Solution
View SolutionFind the magnetic field at the point P in figure. The curved portion is a semicircle and the straight wires are long.
 View Solution
View SolutionA long conducting wire carrying a current I is bent at 120∘( see figure). The magnetic field B at a point P on the right bisector of bending angle at a distance d from the bend is
(μ0 is the permeability of free space)
 View Solution
View SolutionFind the direction of magnetic field at a point P due to two infinite current carrying wires shown in the figure below
 View Solution
View Solution

