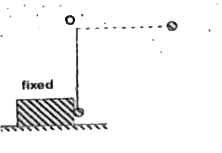
Figure shows a small, plane strip suspended from a fixed support through a string of length l. A continuous beam of monochromati clight is incident horizontally on the strip and is completely absorbed. The energy falling on the strip per unit time is W. (a) Find the deflection of the string from the vertical if the mirror stays in equilibrium. (b) If the strip is deflected slightly from its equilibrium position in the plane of the figure, what will be the time period of the resulting oscillations?

Text Solution

Verified by Experts

(a) The linear momentum of the light falling per unit time on the strip is W/c. As the light is incident on the strip, its momentum is absorbed by the mirror. The change in momentum imparted to the strip per unit time is thus W/c. This is equal to the force on the strip by the light beam, the weight of the strip and the force due to tension add to zero. If the string makes an angle theta with the vertical,
T cos theta = mg
$and T sin θ = \frac{W}{c}$ .
Thus, $tan (θ) = (\frac{W}{m} g c) .$
(b) In equilibrium, the tension is
$T = ⎛ ⎜ ⎝ {[{(m g)}^{2} + {(\frac{W}{c})}^{2}]}^{\frac{1}{2}} ⎞ ⎟ ⎠$
$or, \frac{T}{m} = ⎛ ⎜ ⎝ {[(g^{2}) + {(\frac{W}{m} c)}^{2}]}^{\frac{1}{2}} ⎞ ⎟ ⎠ .$
This plays the role of effective g. The time period of
small oscillations is
$t = (2 π (\sqrt{\frac{l}{t / m}})) = 2 π ⎛ ⎜ ⎜ ⎜ ⎜ ⎜ ⎝ \frac{\sqrt{l}}{\frac{{[(g^{2}) + {(\frac{W}{m} c)}^{2}]}^{1}}{4}} .$

Updated on:21/07/2023

A particle of mass m is suspended from a fixed O by a string of length L. At $t = 0$ , it is displaced from its equilibrium position and released. The graph which shows the variation of the tension T in the string with time t is :

A
B
C
D
A small ball $B$ of mass $m$ is suspended with light inelastic string of length $L$ from a block $A$ of same mass in which can move on smooth horizontal surface as shown in the figure. The ball is displaced by angle $θ$ from equilibrium position and then released.

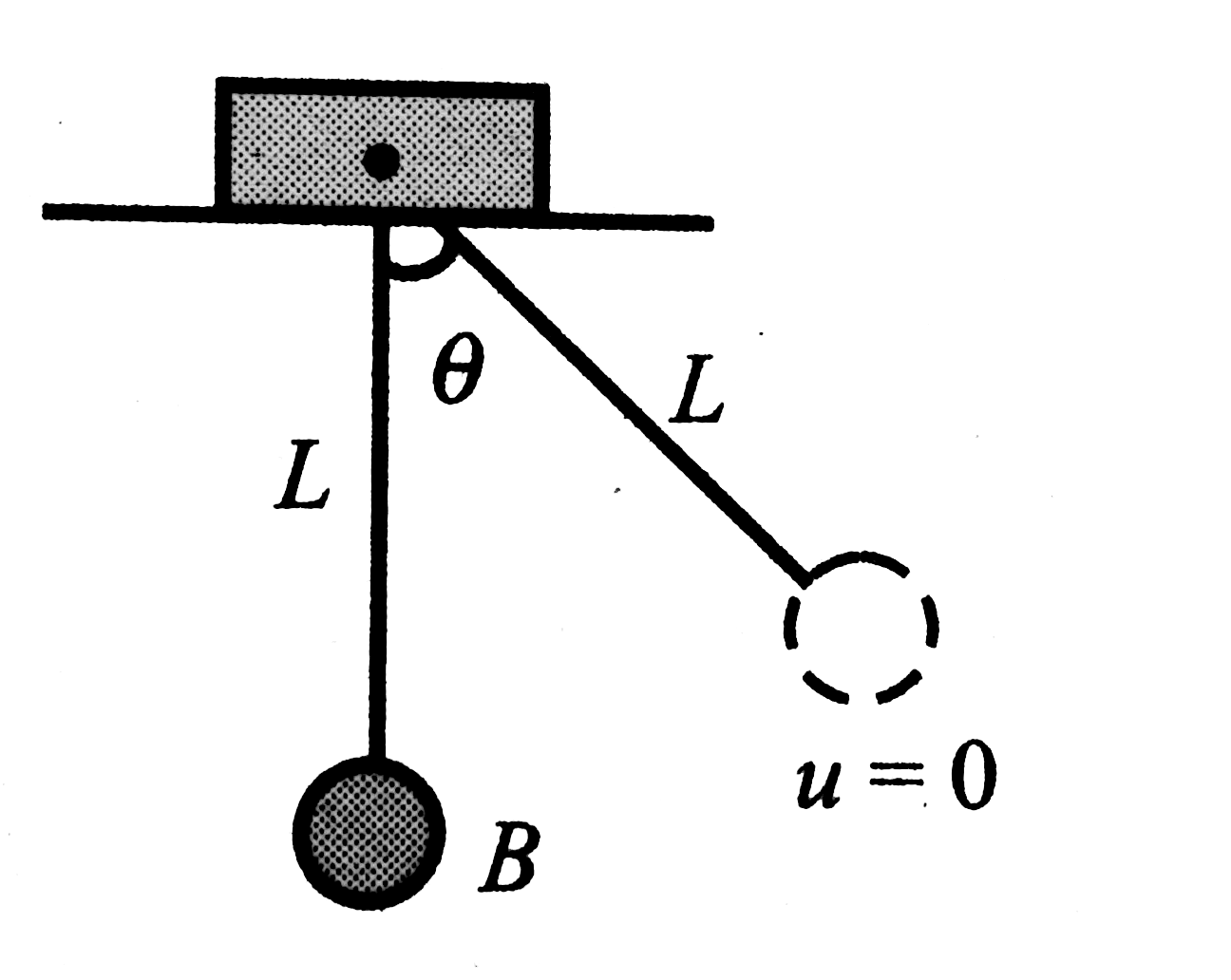
Tension in string when it is vertical, is
A $m g$
B $m g (2 - cos θ)$
C $m g (3 - 2 cos θ)$
Dnone of these
The strips of iron and zinc are riveted together to from a bimetallic strip which bends when heated . The iron is on the inside of the bend because
Ait has a higher coefficient of linear expansion .
Bit has a lower coefficient of linear expansion .
Cit has a higher specific heat .
Dit has a lower specific heat .