A perfectly reflecting solid sphere of radius r is kept in the path of a parallel beam of light of large aperture. If the beam carries an intensity I, find the force exerted by the beam on the sphere.

Let O be the centre of the sphere and OZ be the line
opposite to the incident beam. Consider
a radius OP of the sphere making an angle theta with OZ.
Rotate this radius about OZ to get a circle on the sphere.
Change theta to theta + d theta and rotate the radius about OZ to
get another circle on the sphere. The part of the sphere
between these circles is a ring of area (2π(r2)sinθdθ).
Consider a small part ΔA of this ring at P. Energy of
the light falling on this part in time (Δt) is
ΔU=I(Δt)(ΔAcosθ).
Themomentumofthislightfall∈gonΔAis(ΔUc)along<br>QP.Thelightisref≤ctedbythespherealongPR.The<br>chan≥∈momentumis<br> (Delta p = 2 (Delta U/ c ) cos theta = (2/c) I Delta t (Delta A (cos^2) theta)).<br>alongthe∈ward∥a∥l.Theforceon(ΔA)due→the<br>lightfall∈gonit,is<br> (Delta p/ Delta t ) = 2/c I (Delta A(cos^2)theta)<br>ThisforceisalongPO,therestant–––––forceonther∈g<br>aswellasonthesphereisalongZObysymmetry.The<br>compo≠ntoftheforceon Delta A,alongZOis.<br> (Delta p/ Delta t) cos theta = 2/c (I (Delta A)cos ^3) theta. <br> The force acting on the ring is ,
dF=(2c)I(2π(r2)(sinθ)dθ)cos3θ.
The force on the entire sphere is
F=(∫π20(4π(r2)Ic)(cos3)θsinθdthη)
=−(∫π2θ=0(4π(r2)Ic)cos3θd(cosθ).
=−(4π(r2)Ic)([cos4θ4]π20=(π(r2)Ic).
Note that integration is done only for the hemisphere
that faces the incident beam.
Topper's Solved these Questions
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - objective I
14 Videos
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - objective II
7 Videos
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - question for short answer
13 Videos
- PERMANENT MAGNETSBOOK - HC VERMA ENGLISHCHAPTER - PERMANENT MAGNETSEXERCISE - Short Answer
11 Videos
- PHOTOMETRYBOOK - HC VERMA ENGLISHCHAPTER - PHOTOMETRYEXERCISE - All Questions
38 Videos
Similar Questions
Knowledge Check
A perfectly reflecting solid hemisphere of radius R is placed in the path of a parallel beam of light of large aperture. If the beam carrries an intensity l, find the force exerted by the beam on the hemisphere.
A2πR2lcBπR2lcC4πR2lcDNone of theseA parallel beam of light falls on a solid tranparent sphere.
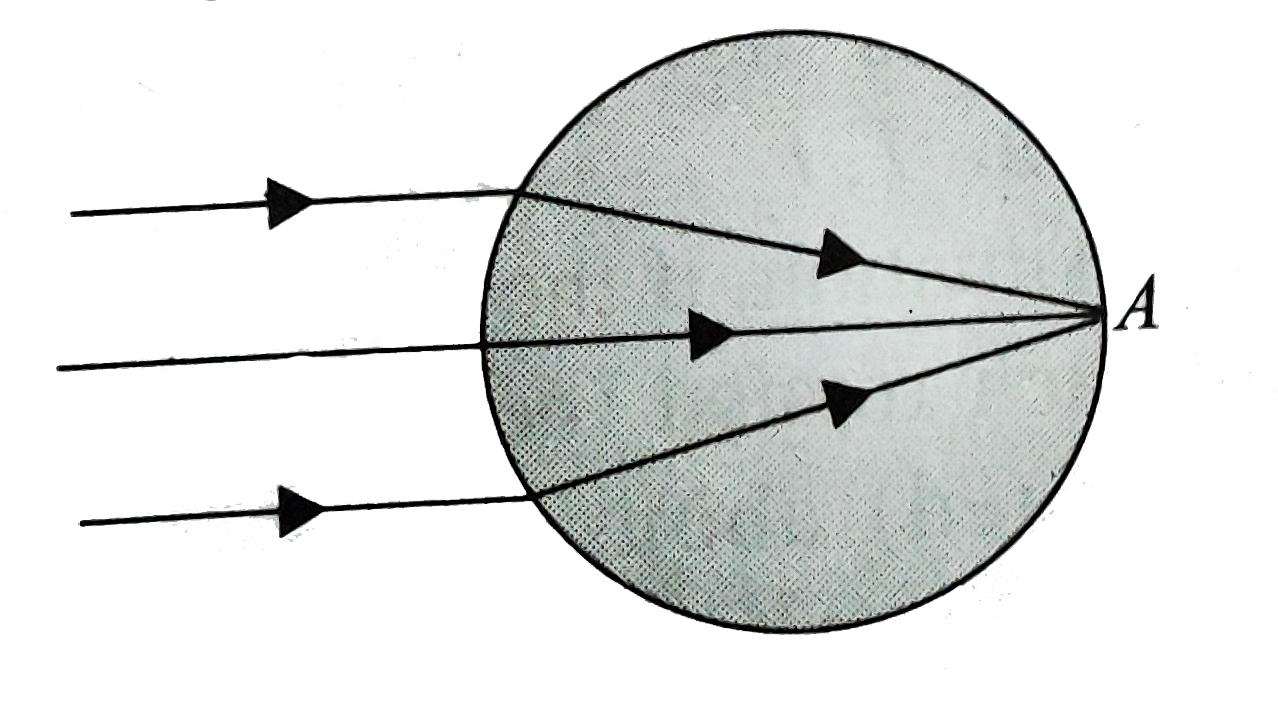
Q. If however thin beam is focussed at A, then find the refractive index of the sphere,A1.5B1.7C2.0D2.5A parallel beam of the light is incident normally on a plane surface absorbing 40% of the light and reflecting the rest. If the incident beam carries 10 watt power, find the force exerted by it on the surface.
A3.2×10−8NB3.2×10−7NC5.12×10−7ND5.12×10−8N
There is a parallel beam of light of larger aperture . Let I be the intensity of light . Perfectly reflecting sphere of radius r is kept in the path of this parallel beam . Find the force exerted by the light beam on sphere .
View SolutionA sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.50 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
View SolutionA sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.50 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
View SolutionForce Exerted By A Light Beam On A Surface
View SolutionA parallel beam of light falls on a solid tranparent sphere.

Q. If however thin beam is focussed at A, then find the refractive index of the sphere,
View Solution
