In diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and tin also crystallise in same way as diamond (NA=6×1023)
The side length of diamond unit cell is (in pm):
154
1422.63
711.32
355.66
The correct Answer is:D
To find the side length (edge length) of the diamond unit cell, we can follow these steps:
1. Understand the Structure:
- In a diamond structure, carbon atoms form a face-centered cubic (FCC) lattice.
- 50% of the tetrahedral voids in the FCC lattice are occupied by carbon atoms.
2. Bond Length Information:
- The bond length between two carbon atoms in diamond is given as 154 pm.
- This bond length represents the distance between the centers of two adjacent carbon atoms.
3. Relationship Between Bond Length and Atomic Radius:
- Since the bond length (distance between two atoms) is 2r, we can express the atomic radius (r) as:
2r=154 pm⟹r=1542=77 pm
4. Determine the Relationship Between Edge Length and Atomic Radius:
- For diamond, the relationship between the edge length (a) of the unit cell and the atomic radius (r) is given by:
√3a4=2r
- Rearranging this gives:
a=8r√3
5. Substituting the Radius:
- Now substitute the value of r into the equation:
a=8×77 pm√3
6. Calculate the Edge Length:
- First, calculate 8×77:
8×77=616 pm
- Next, divide by √3≈1.732:
a=616 pm1.732≈355.66 pm
7. Final Result:
- The edge length of the diamond unit cell is approximately:
a≈355.66 pm
Conclusion:
The side length of the diamond unit cell is 355.66 pm.
---
- In a diamond structure, carbon atoms form a face-centered cubic (FCC) lattice.
- 50% of the tetrahedral voids in the FCC lattice are occupied by carbon atoms.
- The bond length between two carbon atoms in diamond is given as 154 pm.
- This bond length represents the distance between the centers of two adjacent carbon atoms.
- Since the bond length (distance between two atoms) is 2r, we can express the atomic radius (r) as:
2r=154 pm⟹r=1542=77 pm
- For diamond, the relationship between the edge length (a) of the unit cell and the atomic radius (r) is given by:
√3a4=2r
- Rearranging this gives:
a=8r√3
- Now substitute the value of r into the equation:
a=8×77 pm√3
- First, calculate 8×77:
8×77=616 pm
- Next, divide by √3≈1.732:
a=616 pm1.732≈355.66 pm
- The edge length of the diamond unit cell is approximately:
a≈355.66 pm
The side length of the diamond unit cell is 355.66 pm.
Topper's Solved these Questions
- SOLID STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - SOLID STATEEXERCISE - One or More Anwer is / correct
20 Videos
- SOLID STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - SOLID STATEEXERCISE - Match the column
10 Videos
- SOLID STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - SOLID STATEEXERCISE - Passage-6
2 Videos
- IONIC EEQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - IONIC EEQUILIBRIUMEXERCISE - Subjective problems
1 Video
- STOICHIOMETRYBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Match the Colum-II
6 Videos
Similar Questions
In diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and grey tin also crystallise in same way as diamond (NA=6×1023)
The side length of diamond unit cell (in pm):
a. 154
b. 1422.63
c. 711.32
d. 355.66
View SolutionIn diamond structure ,carbon atoms form fcc lattic and 50% tetrahedral voids occupied by acrbon atoms . Evergy carbon atoms is surrounded tetrachedral by four carbon atom with bond length 154 pm . Germanium , silicon and grey tin also crystallise in same way as diamond (NA=6×1023)
The mass of diamond unit cell is:
View SolutionPercentage of tetrahedral voids occupied by carbon atoms per unit cell of diamond is
View SolutionOnly those atoms which four covalent bonds produce a repetitive three dimesional structure using only covalent bonds., e.g. , diamond structure.The latter is based on a FCC lattice where lattice points are occupied by carbon atoms.Every atom in this structure is surrounded tetrahedrally by four others.Germanium, silicon and grey tin also crystallize in the same way as diamond. (Given :NA=6×1023sin54∘44'=0.8164)
If the edge length is 3.60Å, density of diamond crystal is
View SolutionOnly those atoms which four covalent bonds produce a repetitive three dimesional structure using only covalent bonds., e.g. , diamond structure.The latter is based on a FCC lattice where lattice points are occupied by carbon atoms.Every atom in this structure is surrounded tetrahedrally by four others.Germanium, silicon and grey tin also crystallize in the same way as diamond. (Given :NA=6×1023sin54∘44'=0.8164)
If edge length of the cube is 3.60Å, then radius of carbon atom is
View SolutionNumber of octahedral void(s) occupied by carbon atoms in the unit cell of diamond is
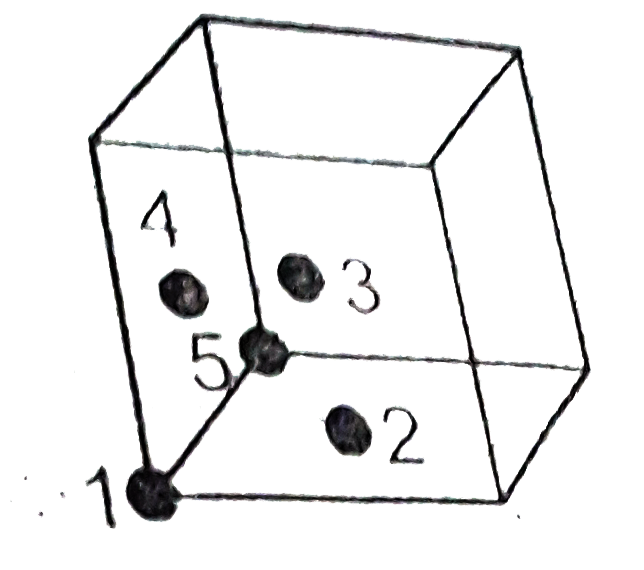
View SolutionTetrahedral voids is surrounded by four atoms. Which atoms in the given figure are around the tetrahedral voids ?
View SolutionIn a crystal of diamond :
How many carbon atoms surround each carbon atom
View SolutionIn a diamond, each carbon atom is bonded to four other carbon atoms tetrahedrally. Alternate tetrahedral voids are occupied by carbon atoms. The number of carbon atoms per unit cell is:
View SolutionNumber of tetrahedral voids occupied in a unit cell of diamond is
View Solution