The van der Waals' constant 'b' of a gas is 4π×10−4L/mol. How near can the centeres of the two molecules approach each other? [Use :NA=6×1023]
10−7 m
10−10 m
5×10−11 m
5×10−9 m
The correct Answer is:b
To solve the problem, we need to determine how near the centers of two gas molecules can approach each other, given the van der Waals constant 'b' and Avogadro's number.
1. Understand the van der Waals constant 'b':
The van der Waals constant 'b' is related to the volume occupied by one mole of gas molecules. It is given by the equation:
b=NA⋅43πR3
where R is the radius of a gas molecule, and NA is Avogadro's number.
2. Substitute the given values:
We have:
b=4π×10−4L/mol
and
NA=6×1023molecules/mol
3. Rearranging the equation for R:
Rearranging the equation for R:
R3=3b4πNA
Now substitute the values of b and NA:
R3=3×(4π×10−4)4π×(6×1023)
4. Simplifying the equation:
The 4π terms cancel out:
R3=3×10−46×1023=36×10−4×10−23=12×10−27
R3=0.5×10−27
5. Calculating R:
Now, take the cube root:
R=(0.5×10−27)1/3
This can be calculated as:
R=1√(3)2×10−9m≈0.7937×10−9m≈7.937×10−10m
6. Finding the distance between two molecules:
The distance between the centers of two molecules when they are just touching each other is:
d=2R≈2×7.937×10−10m≈1.5874×10−9m≈1.59×10−9m
Final Answer:
The centers of the two molecules can approach each other to a distance of approximately 1.59×10−9m.
The van der Waals constant 'b' is related to the volume occupied by one mole of gas molecules. It is given by the equation:
b=NA⋅43πR3
where R is the radius of a gas molecule, and NA is Avogadro's number.
We have:
b=4π×10−4L/mol
and
NA=6×1023molecules/mol
Rearranging the equation for R:
R3=3b4πNA
Now substitute the values of b and NA:
R3=3×(4π×10−4)4π×(6×1023)
The 4π terms cancel out:
R3=3×10−46×1023=36×10−4×10−23=12×10−27
R3=0.5×10−27
Now, take the cube root:
R=(0.5×10−27)1/3
This can be calculated as:
R=1√(3)2×10−9m≈0.7937×10−9m≈7.937×10−10m
The distance between the centers of two molecules when they are just touching each other is:
d=2R≈2×7.937×10−10m≈1.5874×10−9m≈1.59×10−9m
The centers of the two molecules can approach each other to a distance of approximately 1.59×10−9m.
Topper's Solved these Questions
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 1
4 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 2
6 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Subjective problems
15 Videos
- ELECTROCHEMISTRYBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Subjective problems
14 Videos
- IONIC EEQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - IONIC EEQUILIBRIUMEXERCISE - Subjective problems
1 Videos
Similar Questions
The van der Waals constant ‘b’ for oxygen is 0.0318 L mol−1. Calculate the diameter of the oxygen molecule.
View SolutionThe van der Waals constant b of Ar is 3.22×10−5m3mol−1. Calculate the molecular diameter of Ar.
View SolutionThe vander waals constatn 'b' of a gas is 4π×10−4L/mol. The radius of gas atom can be expressed in secientific notation as z=10−9cm. Calculate the value fo z.(Given NA=6×1023)
View SolutionThe hydronium lon concentration of a fruit juice is 4.6×10−4 mol L−1 . What is the pH of the juice?
View SolutionThe van der Waals constant for hydrogen is 0.025N−m4/mol2. What is the internal pressure of hydrogen if V=22.4×10−3m3 ?
View SolutionEach molecular of a compound contains 5 carbon atoms, 8 hydrogen atoms and 4×10−23gm of other element. The molecular mass of compounds is (NA=6×1023)
View SolutionIf NaCl is doped with 10−4mol%of SrCl2 the concentration of cation vacancies will be (NA=6.02×1023mol−1)
View Solution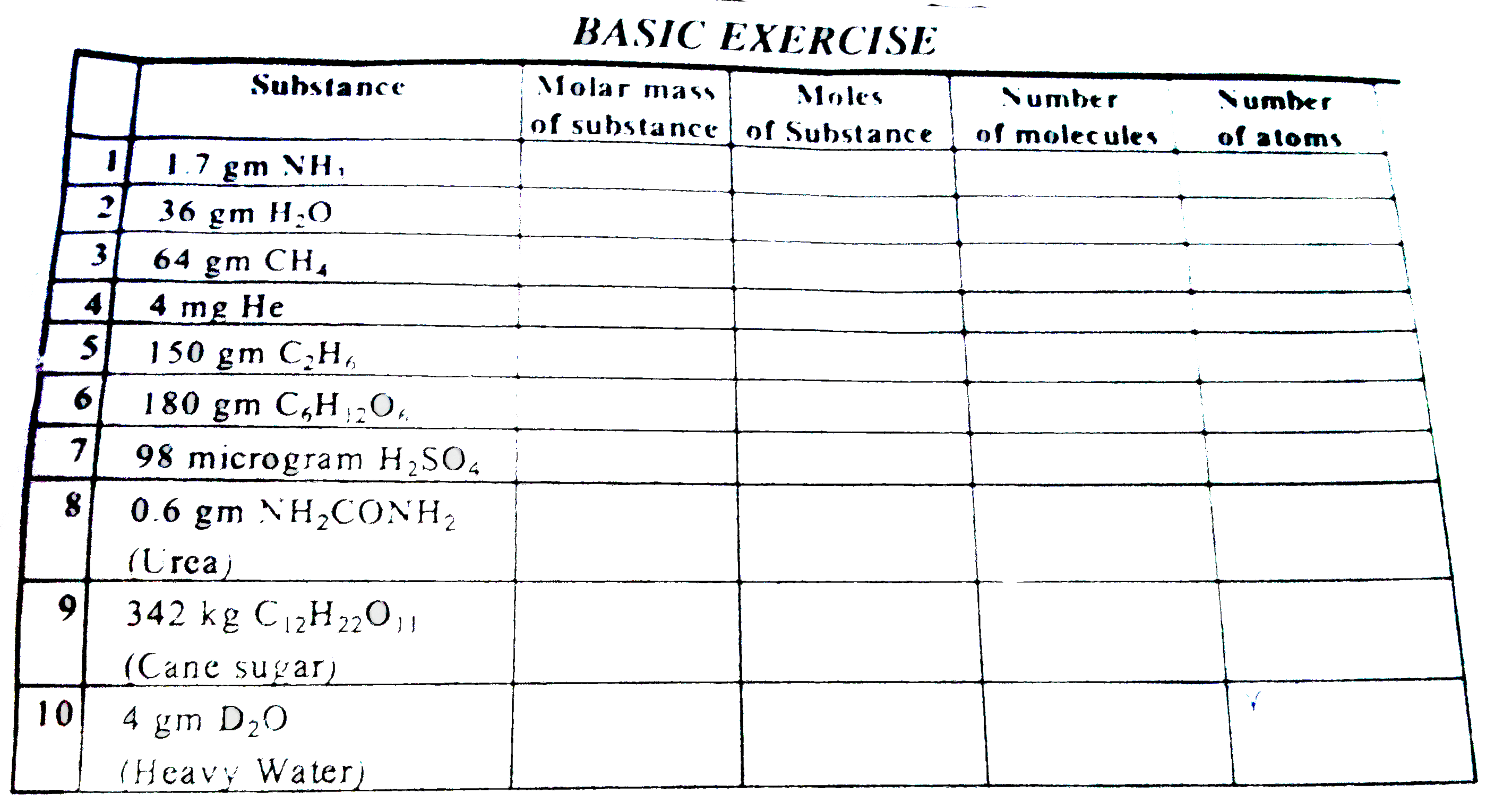
If a closed container contain 5 moles of CO2 gas, then find total number of CO2 molecules in the container (NA=6×1023)
View Solution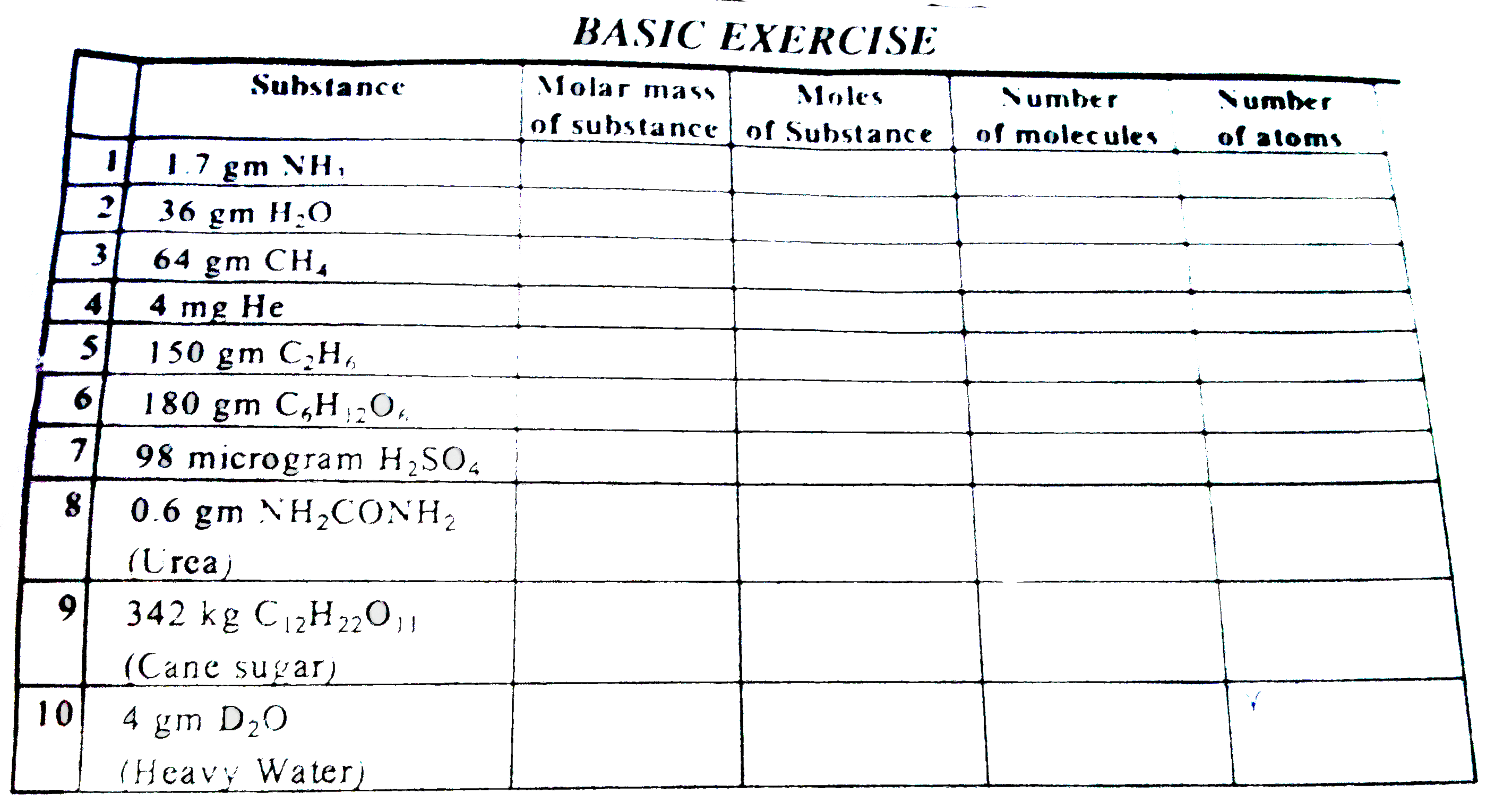
How many moles of O-atoms are in 2.7×1025 molecules of CO2(NA=6×1023)
View Solutionvan der Waal's equation for calculating the pressure of a non ideal gas is
(P+an2V2)(V−nb)=nRT
van der Waal's suggested that the pressure exerted by an ideal gas , Pideal, is related to the experiventally measured pressure, Pideal by the equation
Pideal=Preal↑observed pressure+an2V2↑currection term
Constant 'a' is measure of intermolecular interaction between gaseous molecules that gives rise to nonideal behavior. It depends upon how frequently any two molecules approach each other closely. Another correction concerns the volume occupied by the gas molecules. In the ideal gas equation, V represents the volume of the container. However, each molecule does occupy a finite, although small, intrinsic volume, so the effective volume of the gas vecomes (V-nb), where n is the number of moles of the gas and b is a constant. The term nb represents the volume occupied by gas particles present in n moles of the gas .
Having taken into account the corrections for pressure and volume, we can rewrite the ideal gas equation as follows :
(P+an2V2)corrected pressure(V−nb)corrected volume=nRT
AT relatively high pressures, the van der Waals' equation of state reduces to
View Solution
