If the slope of 'Z' (compressibility factor) vs. 'p' curve is constant (slope=π492.6atm−1) at a particular temperature (300 K) abd very high pressure, then calculate diameter of the molecules.
(Given : NA=6.0×1023,R=0.0821atm.Lmol−1K−1)
7.5 Å
5 Å
2.5 Å
1.25 Å
The correct Answer is:b
To solve the problem, we need to calculate the diameter of the molecules based on the given slope of the compressibility factor (Z) vs. pressure (P) curve. The slope is given as π492.6atm−1 at a temperature of 300 K and very high pressure.
1. Understanding the Compressibility Factor (Z):
The compressibility factor Z is defined as:
Z=PVnRT
where P is the pressure, V is the volume, n is the number of moles, R is the universal gas constant, and T is the temperature.
2. Using the Van der Waals Equation:
For real gases, the Van der Waals equation is:
(P+aV2)(V−b)=RT
where a accounts for intermolecular forces and b accounts for the volume occupied by the gas molecules.
3. At High Pressure:
At very high pressure, the term a becomes negligible, and we can simplify the equation to:
PV≈nRT
This leads to:
Z≈1+PBRT
where B is the volume excluded due to the finite size of the molecules.
4. Finding the Slope:
The slope of the Z vs. P curve is given as:
slope=π492.6atm−1
From the equation Z=1+PBRT, we can see that the slope is equal to BRT.
5. Calculating B:
Rearranging the slope equation gives:
B=slope×RT
Substituting the values:
B=(π492.6)×(0.0821atm L mol−1K−1)×(300K)
6. Calculating B Numerically:
B=π×0.0821×300492.6
B≈77.439492.6≈0.157
7. Relating B to Molecular Volume:
The value of B can be expressed in terms of the volume of one molecule:
B=4×NA×Vm
where Vm is the volume of one molecule and NA is Avogadro's number (6.022×1023mol−1).
8. Finding the Volume of One Molecule:
Rearranging gives:
Vm=B4NA
Substituting the values:
Vm=0.1574×6.022×1023≈0.1572.4088×1024≈6.52×10−26m3
9. Calculating the Diameter:
The volume of a sphere is given by:
V=43πr3
Setting Vm=43πr3 and solving for r:
r3=3Vm4π
r=(3×6.52×10−264π)1/3
10. Calculating the Diameter:
d=2r
After calculating r and multiplying by 2, we find:
d≈5Å
Final Answer:
The diameter of the molecules is approximately 5Å.
The compressibility factor Z is defined as:
Z=PVnRT
where P is the pressure, V is the volume, n is the number of moles, R is the universal gas constant, and T is the temperature.
For real gases, the Van der Waals equation is:
(P+aV2)(V−b)=RT
where a accounts for intermolecular forces and b accounts for the volume occupied by the gas molecules.
At very high pressure, the term a becomes negligible, and we can simplify the equation to:
PV≈nRT
This leads to:
Z≈1+PBRT
where B is the volume excluded due to the finite size of the molecules.
The slope of the Z vs. P curve is given as:
slope=π492.6atm−1
From the equation Z=1+PBRT, we can see that the slope is equal to BRT.
Rearranging the slope equation gives:
B=slope×RT
Substituting the values:
B=(π492.6)×(0.0821atm L mol−1K−1)×(300K)
B=π×0.0821×300492.6
B≈77.439492.6≈0.157
The value of B can be expressed in terms of the volume of one molecule:
B=4×NA×Vm
where Vm is the volume of one molecule and NA is Avogadro's number (6.022×1023mol−1).
Rearranging gives:
Vm=B4NA
Substituting the values:
Vm=0.1574×6.022×1023≈0.1572.4088×1024≈6.52×10−26m3
The volume of a sphere is given by:
V=43πr3
Setting Vm=43πr3 and solving for r:
r3=3Vm4π
r=(3×6.52×10−264π)1/3
d=2r
After calculating r and multiplying by 2, we find:
d≈5Å
The diameter of the molecules is approximately 5Å.
Topper's Solved these Questions
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 1
4 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 2
6 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Subjective problems
15 Videos
- ELECTROCHEMISTRYBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Subjective problems
14 Videos
- IONIC EEQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - IONIC EEQUILIBRIUMEXERCISE - Subjective problems
1 Videos
Similar Questions
Calculate the K.E. per mole of oxygen at 27∘C. Given N=6.02×1023,k=1.38×10−23JK−1.
View SolutionCalculate the average kinetic energy of hydrogen molecule at 0∘C. Given kB=1.38×10−23JK−1.
View SolutionCalculate the average kinetic energy of hydrogen molecule at 0∘C. Given kB=1.38×10−23JK−1.
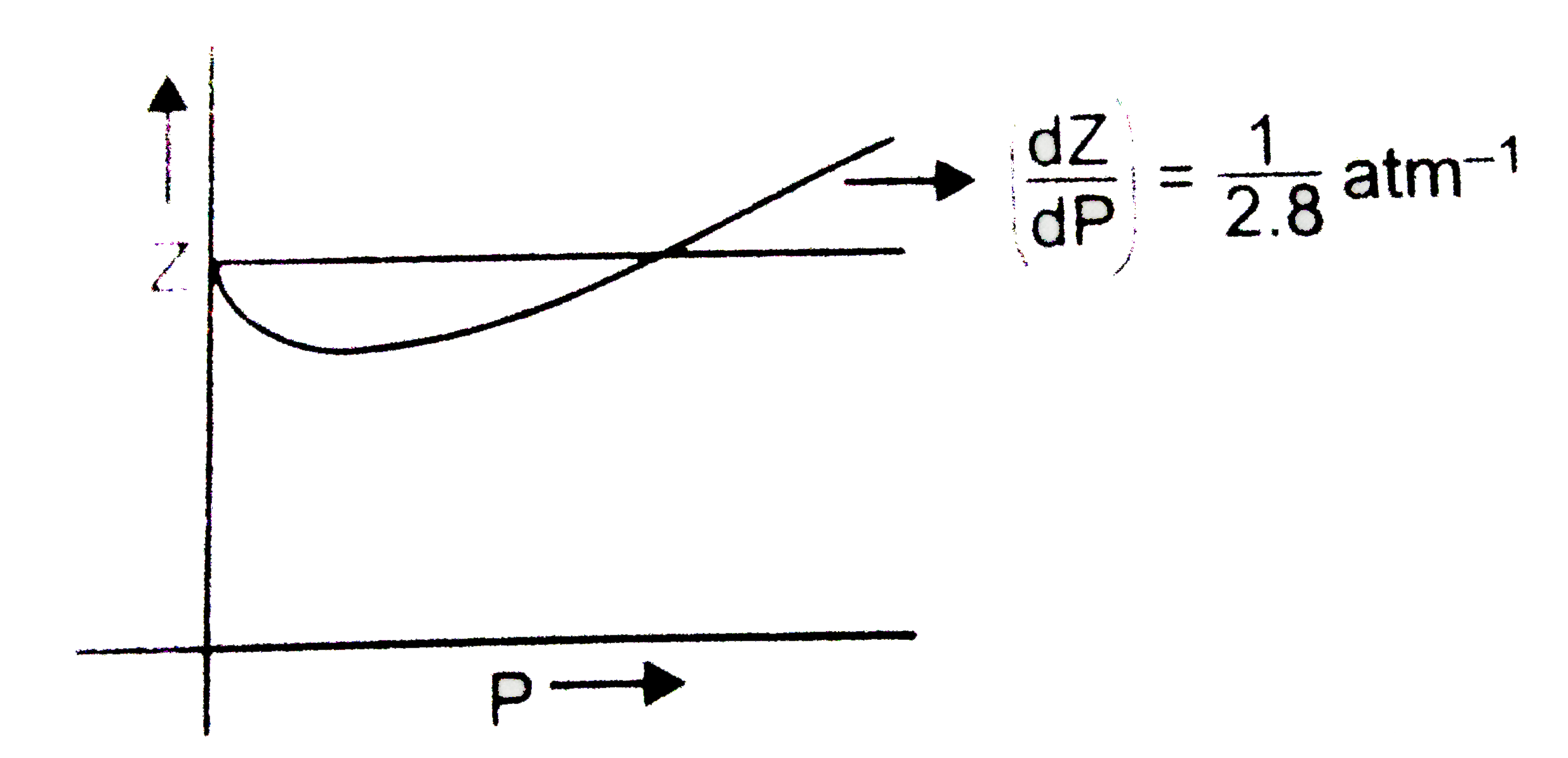
View SolutionThe graph of compressibility factor (Z) vs. P for one mole of a real gas is shown in following diagram. The graph is plotted at constant temperature 273K. If the slope of graph at very high pressure (dZdP) is (12.8)atm−1 , then calculate volume of one mole of real gas molecules (in L/mol)
Given : NA=6×1023 and R=22.4273LatmK−1mol−1
View SolutionCalculate the average kinetic energy of oxygen molecule at 0∘C. (R=8.314Jmol−1 K−1,NA=6.02×1023)
View SolutionGas molecules each of mass 10−26 kg are taken in a container of volume 1dm3 . The root mean square speed of gas molecules is 1 km sec−1 .What is the temperature fo gas molecules.
(Given : NA=6×1023,R=8J/mol.K)
View SolutionThe volume of 2.8g of CO at 27∘C and 0.821atm pressure is (R=0.0821 lit. atm mol−1K−1)
View SolutionFor a 5% solution of urea (Molar mass - 60 g/mol), calculate the osmotic pressure at 300 K. [R=0.0821LatmK−1mol−1]
View SolutionThe figure given below shows three glass chambers that are connected by valves of negligible volume. At the outset of an experiment, the valves are closed and the chambers contain the gases as detailed in the diagram. All the chambers are at the temperature of 300K and external pressure of 1.0atm.
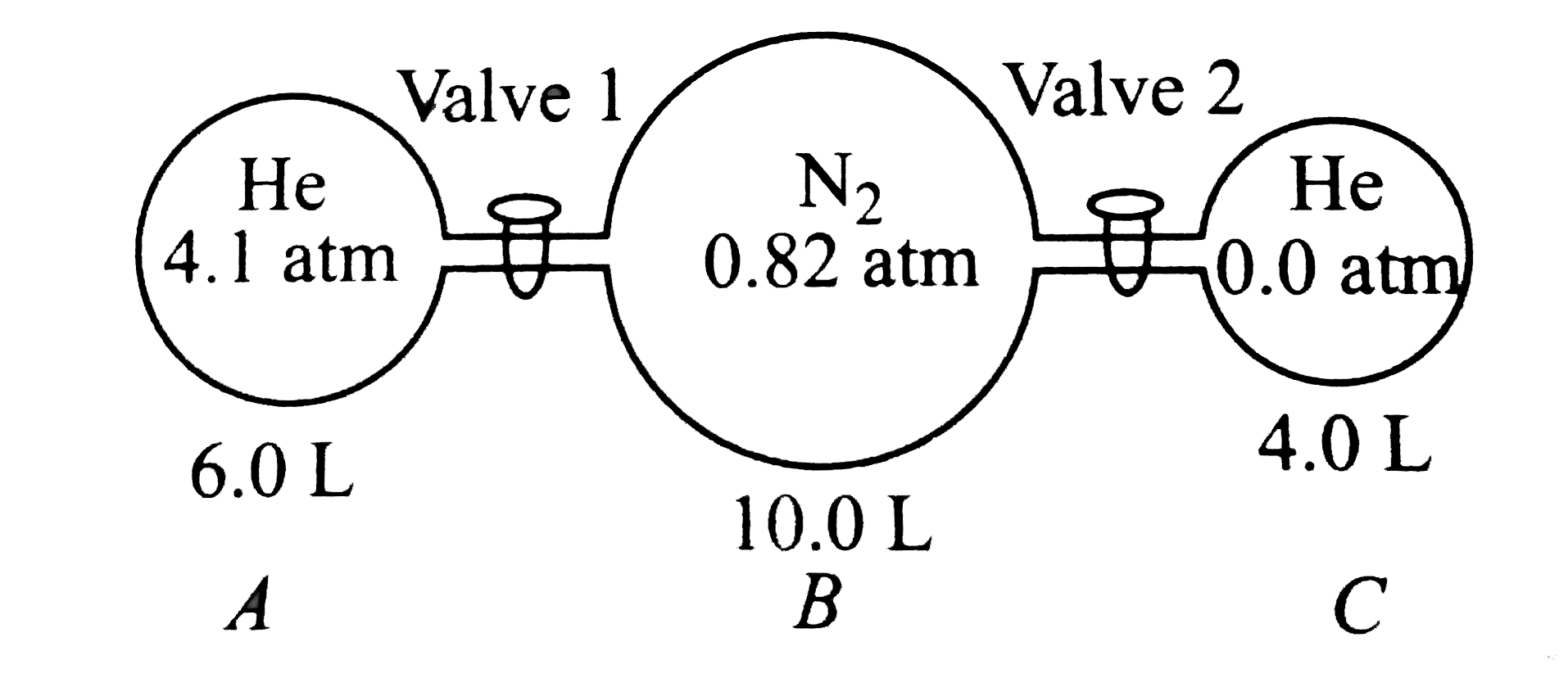
Which of the following represents the total kinetic energy of all the gas molecules after both valves are opened?
(R=0.082atmLK−1mol−1=8.314JK_1mol−1)
View SolutionWhat is the density of N2 gas at 227∘Cand5.00atm pressure? (R=0.0821atmK−1mol−1)
View Solution
