A carbon resistance with color band is 2000Ω. If red band is replaced by green band then the new resistance is : (A) 500Ω (B) 300Ω (C) 400Ω (D) 100Ω
To solve the problem, we need to understand how the color bands on a resistor indicate its resistance value. The resistance value is determined by the color bands according to a specific code.
1. Identify the Color Code: The color bands on a resistor represent digits according to the following code:
- Black = 0
- Brown = 1
- Red = 2
- Orange = 3
- Yellow = 4
- Green = 5
- Blue = 6
- Violet = 7
- Gray = 8
- White = 9
2. Determine the Original Resistance: The problem states that the original resistance is 2000ohms. The color code for 2000ohms can be broken down as:
- The first digit (2) corresponds to Red.
- The second digit (0) corresponds to Black.
- The third digit (0) corresponds to Black.
- The multiplier (10^2) corresponds to Red.
3. Replacing the Red Band with Green: If we replace the first Red band (which represents 2) with a Green band (which represents 5), we need to analyze how this affects the resistance:
- The new resistance would now start with 5 instead of 2.
- The new resistance value will be 500ohms because the new configuration will be:
- First digit (5) from Green.
- Second digit (0) from Black.
- Third digit (0) from Black.
- The multiplier remains the same (10^2).
4. Conclusion: Therefore, the new resistance after replacing the red band with the green band is 500ohms.
Final Answer:
The new resistance is (A) 500 ohms.
- Black = 0
- Brown = 1
- Red = 2
- Orange = 3
- Yellow = 4
- Green = 5
- Blue = 6
- Violet = 7
- Gray = 8
- White = 9
- The first digit (2) corresponds to Red.
- The second digit (0) corresponds to Black.
- The third digit (0) corresponds to Black.
- The multiplier (10^2) corresponds to Red.
- The new resistance would now start with 5 instead of 2.
- The new resistance value will be 500ohms because the new configuration will be:
- First digit (5) from Green.
- Second digit (0) from Black.
- Third digit (0) from Black.
- The multiplier remains the same (10^2).
The new resistance is (A) 500 ohms.
Similar Questions
Current in 3Ω resistance is

View SolutionA 200Ω resistor has a certain color code. If one replaces the red color by green in the code, the new resistance will be:
View SolutionA resistance of 2Ω is connected across one gap of a meter bridge (the length of the wire is 100cm and an unknown resistance, greater than 2Ω, is connected across the other gap. When these resistance are interchanged, the balance point shifts by 20cm. Neglecting any corrections, the unknown resistance is.
(a)3Ω
(b) 4Ω
( c) 5Ω
(d) 6Ω.
View SolutionTwo resistors having resistance 4 Ω and 6 Ω are connected in parallel. Find their equivalent resistance.
View SolutionHow can three resistors of resistances 2Ω,3Ω, and 6Ω be connected to give a total resistance of
(a) 4Ω
(b) 1Ω ?
View SolutionWhat resistance must be connected to a 15 Ω resistance to provide an effective resistance of 6Ω ?
View SolutionEquivalent resistance between the points A and B (in Ω)
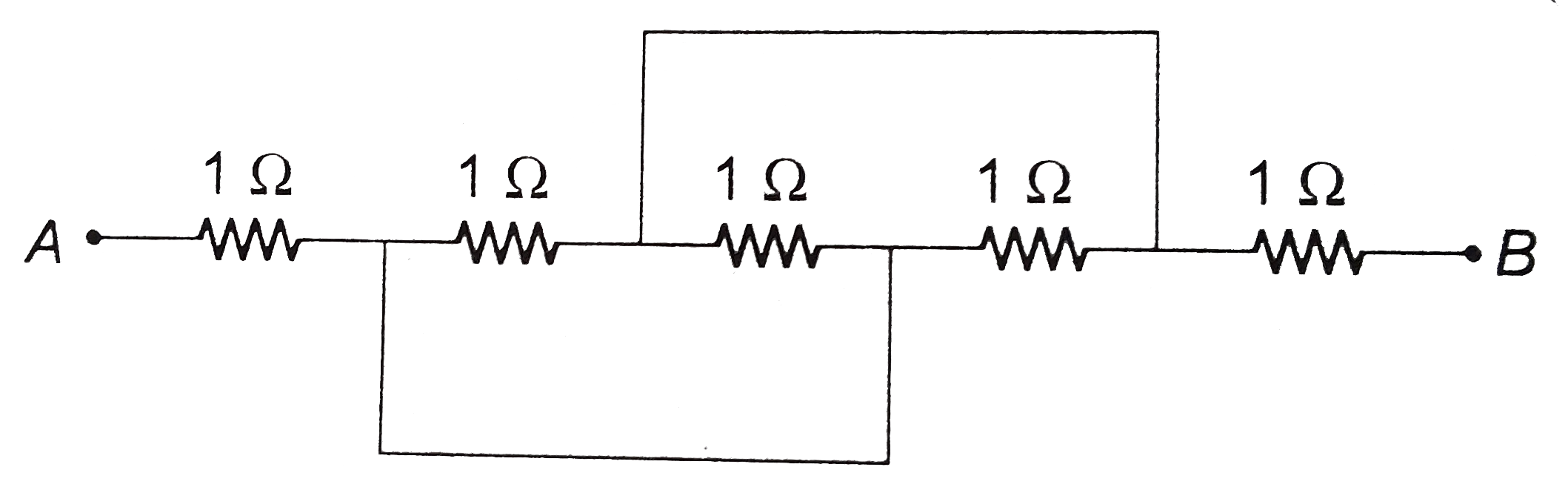 .
.
View SolutionA platinum resistance thermometer reads 0∘C when its resistance is 80Ωand100∘C when its resistance is 90Ω. Find the temperature at which the resistance is 86Ω.
View SolutionThe resistance of a wire at 20∘C is 20Ω and at 500∘C is 60Ω. At which temperature its resistance will be 25Ω?
View Solution(a) Given n resistors each of resistance R. How will you combine them to get the (i) maximum (ii) minimum effective resistance ? What is the ratio of the maximum to minimum resistance ?
(b) Given the resistances of 1Ω,2Ω,3Ω, how will you combine them to get an equivalent resistance of (i) (11/3)Ω (ii) (11/5)Ω (iii) 6Ω (iv) (6/11)Ω ?
(c) Determine the equivalent resistance of networks shown in Figure.
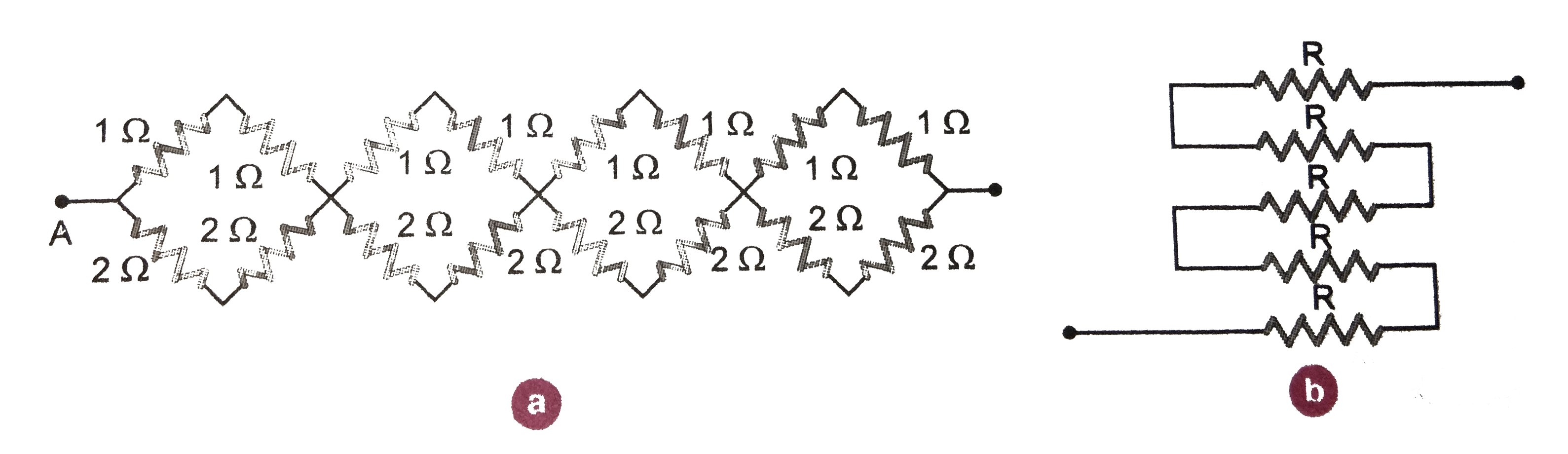
View Solution
