The bob of a simple pendulum has mass and a charge of . It is at rest in a uniform horizontal electric field of intensity . At equilibrium, the angle that the pendulum makes with the vertical is: (take )
The correct Answer is:B
To solve the problem, we need to find the angle θ that the pendulum bob makes with the vertical when it is at equilibrium in a uniform horizontal electric field.
1. Identify the Forces Acting on the Bob:
- The bob has a mass m=2g=0.002kg.
- The charge on the bob is Q=5.0μC=5.0×10−6C.
- The electric field intensity is E=2000V/m.
- The gravitational force acting on the bob is given by Fg=mg=0.002×10=0.02N.
2. Calculate the Electric Force:
- The electric force Fe acting on the bob due to the electric field is given by:
Fe=Q⋅E=(5.0×10−6)×2000=0.01N
3. Set Up the Equilibrium Condition:
- At equilibrium, the forces acting on the bob can be resolved into two components:
- The gravitational force Fg acts downward.
- The electric force Fe acts horizontally (to the right).
- The pendulum will make an angle θ with the vertical.
4. Use Trigonometry to Relate Forces:
- In the equilibrium position, we can use the tangent of the angle θ:
tanθ=OppositeAdjacent=FeFg
- Substituting the values:
tanθ=0.010.02=12
5. Calculate the Angle θ:
- Now, we can find θ using the inverse tangent function:
θ=tan−1(12)
6. Final Answer:
- Using a calculator to find tan−1(0.5):
θ≈26.57∘
Conclusion:
The angle that the pendulum makes with the vertical at equilibrium is approximately 26.57∘.
- The bob has a mass m=2g=0.002kg.
- The charge on the bob is Q=5.0μC=5.0×10−6C.
- The electric field intensity is E=2000V/m.
- The gravitational force acting on the bob is given by Fg=mg=0.002×10=0.02N.
- The electric force Fe acting on the bob due to the electric field is given by:
Fe=Q⋅E=(5.0×10−6)×2000=0.01N
- At equilibrium, the forces acting on the bob can be resolved into two components:
- The gravitational force Fg acts downward.
- The electric force Fe acts horizontally (to the right).
- The pendulum will make an angle θ with the vertical.
- In the equilibrium position, we can use the tangent of the angle θ:
tanθ=OppositeAdjacent=FeFg
- Substituting the values:
tanθ=0.010.02=12
- Now, we can find θ using the inverse tangent function:
θ=tan−1(12)
- Using a calculator to find tan−1(0.5):
θ≈26.57∘
The angle that the pendulum makes with the vertical at equilibrium is approximately 26.57∘.
Topper's Solved these Questions
Similar Questions
A pendulum bob of mass 80mg and carrying a charge of 2×10−8C is at rest in a uniform, horizontal electric field of 20k Vm−1. Find the tension in the thread.
View SolutionA pendulum bob of mass m = 80 mg, carrying a charge of q=2×10−8C, is at rest in a horizontal, uniform electric field of E = 20,000 V/m. The tension T in the thread of the pendulum and the angle α it makes with vertical, is (take g=9.8m/s2)
View SolutionThe bob of a pendulum of mass 8μg carries an electric charge of 39.2×10−10 coulomb in an electric field of 20×103 volt/meter and it is at rest. The angle made by the pendulum with the vertical will be
View SolutionA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
View SolutionThe bob of a simple pendulum of length 1 m has mass 100 g and a speed of 1.4 m/s at the lowest point in its path. Find the tension in the stirng at this instant.
View SolutionA simple pendulum has a length l & mass of bob m. The bob is given a charge q coulomb. The pendulum is suspended in a uniform horizontal electric field of strength E as shown in figure, then calculate the time period of oscillation when bob is slightly displaced from its mean position.
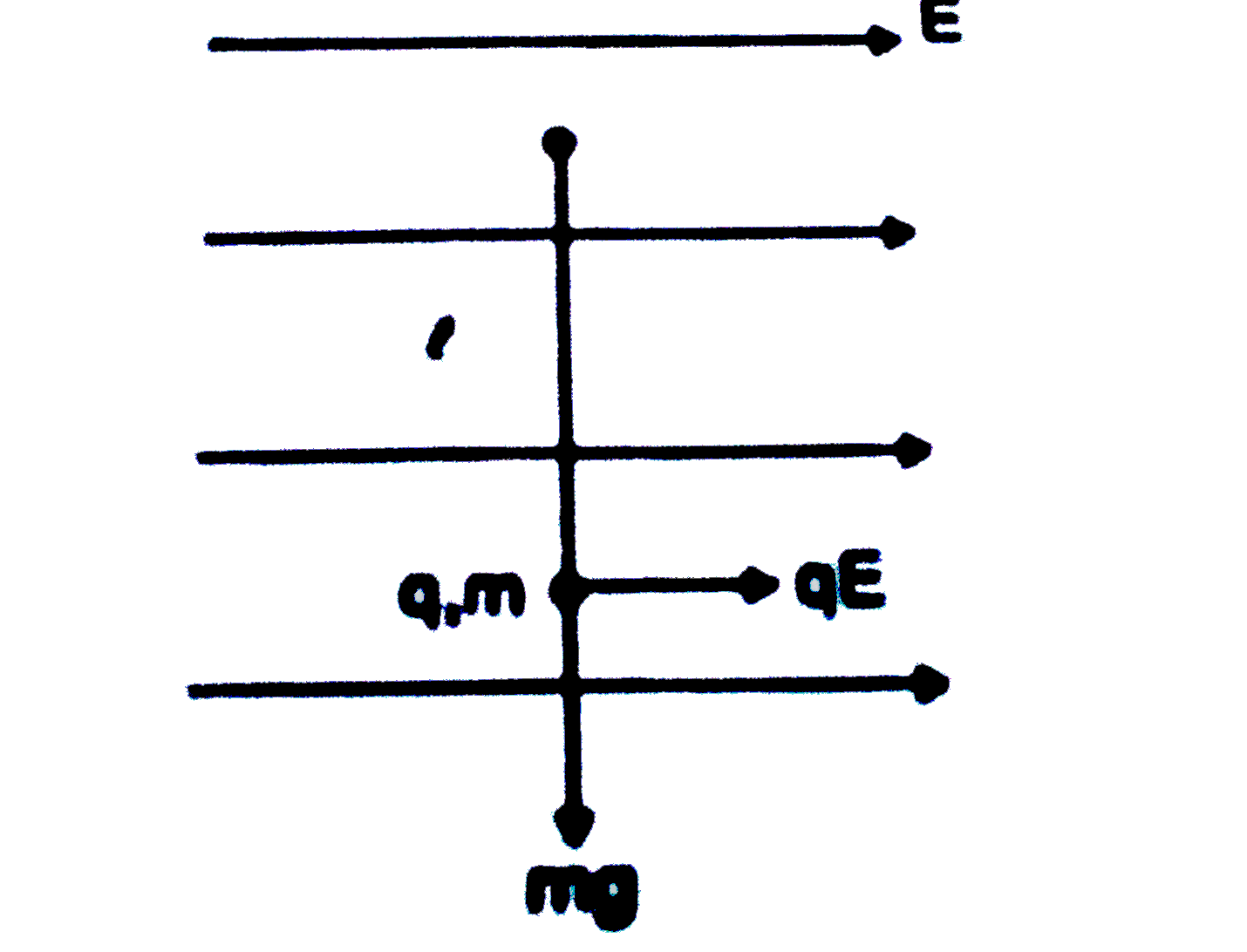
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionA simple pendulum is suspended from the ceiling of a car taking a turn of radius 10 m at a speed of 36 km/h. Find the angle made by the string of the pendulum with the vertical if this angle does not change during the turn. Take g=10ms2.
View SolutionThe bob of a simple pendulum has a mass of 40 g and a positive charge of 4.0×10−5C . It makes 20 oscillations in 45 s. A vertical electric field pointing upward and of magnitude 2.5×104NC−1 is switched on. How much time will it now take to complete 20 oscillations?
View SolutionA bob of a simple pendulum of mass 40 mg with a positive charge 4×10−6C is oscilliating with time period T1. An electric field of intensity 3.6×104 N/c is applied vertically upwards now time period is T2. The value of T2T1 is (g=10ms2)
View Solution
