A circuit connected to ac source of emf e=e0(100t) with t in second gives a phase difference of π4 between , emf e and current i. which of the following circuits will exhibit this?
RL Circuit with R=1Kω and L=10mH
RL circuit With R=1Kω and L=1mH
RC circuit With R=1KΩ and C=1μF
RC circuit with R=1kΩ and C=10μF
The correct Answer is:C
To solve the problem, we need to determine which circuit configuration will exhibit a phase difference of π4 (or 45 degrees) between the EMF e and the current i. The EMF is given by e=e0sin(100t).
1. Understand the Circuit Configuration:
- The phase difference between the voltage (EMF) and the current in an AC circuit can be influenced by the type of components in the circuit (resistor, inductor, capacitor).
- In an RL circuit, the phase difference ϕ is given by tan(ϕ)=XLR, where XL=ωL is the inductive reactance.
- In an RC circuit, the phase difference is given by tan(ϕ)=XCR, where XC=1ωC is the capacitive reactance.
2. Calculate the Phase Difference:
- We need to find the conditions under which the phase difference ϕ=π4.
- For an RL circuit, this means:
tan(π4)=1⟹XLR=1⟹XL=R
- For an RC circuit, this means:
tan(π4)=1⟹XCR=1⟹XC=R
3. Determine Values:
- Given ω=100 rad/s (from 100t), we can express:
- For RL circuit: XL=ωL=100L
- For RC circuit: XC=1ωC=1100C
4. Check Each Option:
- Option A: R=1kΩ, L=10mH
- XL=100×10×10−3=1Ω (not equal to R)
- Option B: R=1kΩ, L=1mH
- XL=100×1×10−3=0.1Ω (not equal to R)
- Option C: R=1kΩ, C=1μF
- XC=1100×1×10−6=10Ω (not equal to R)
- Option D: R=1kΩ, C=10μF
- XC=1100×10×10−6=100Ω (equal to R)
5. Conclusion:
- The only option where the phase difference is π4 is Option D.
Final Answer:
Option D is the correct answer.
- The phase difference between the voltage (EMF) and the current in an AC circuit can be influenced by the type of components in the circuit (resistor, inductor, capacitor).
- In an RL circuit, the phase difference ϕ is given by tan(ϕ)=XLR, where XL=ωL is the inductive reactance.
- In an RC circuit, the phase difference is given by tan(ϕ)=XCR, where XC=1ωC is the capacitive reactance.
- We need to find the conditions under which the phase difference ϕ=π4.
- For an RL circuit, this means:
tan(π4)=1⟹XLR=1⟹XL=R
- For an RC circuit, this means:
tan(π4)=1⟹XCR=1⟹XC=R
- Given ω=100 rad/s (from 100t), we can express:
- For RL circuit: XL=ωL=100L
- For RC circuit: XC=1ωC=1100C
- Option A: R=1kΩ, L=10mH
- XL=100×10×10−3=1Ω (not equal to R)
- Option B: R=1kΩ, L=1mH
- XL=100×1×10−3=0.1Ω (not equal to R)
- Option C: R=1kΩ, C=1μF
- XC=1100×1×10−6=10Ω (not equal to R)
- Option D: R=1kΩ, C=10μF
- XC=1100×10×10−6=100Ω (equal to R)
- The only option where the phase difference is π4 is Option D.
Option D is the correct answer.
Topper's Solved these Questions
Similar Questions
Give the difference between e.m.f. and potential difference.
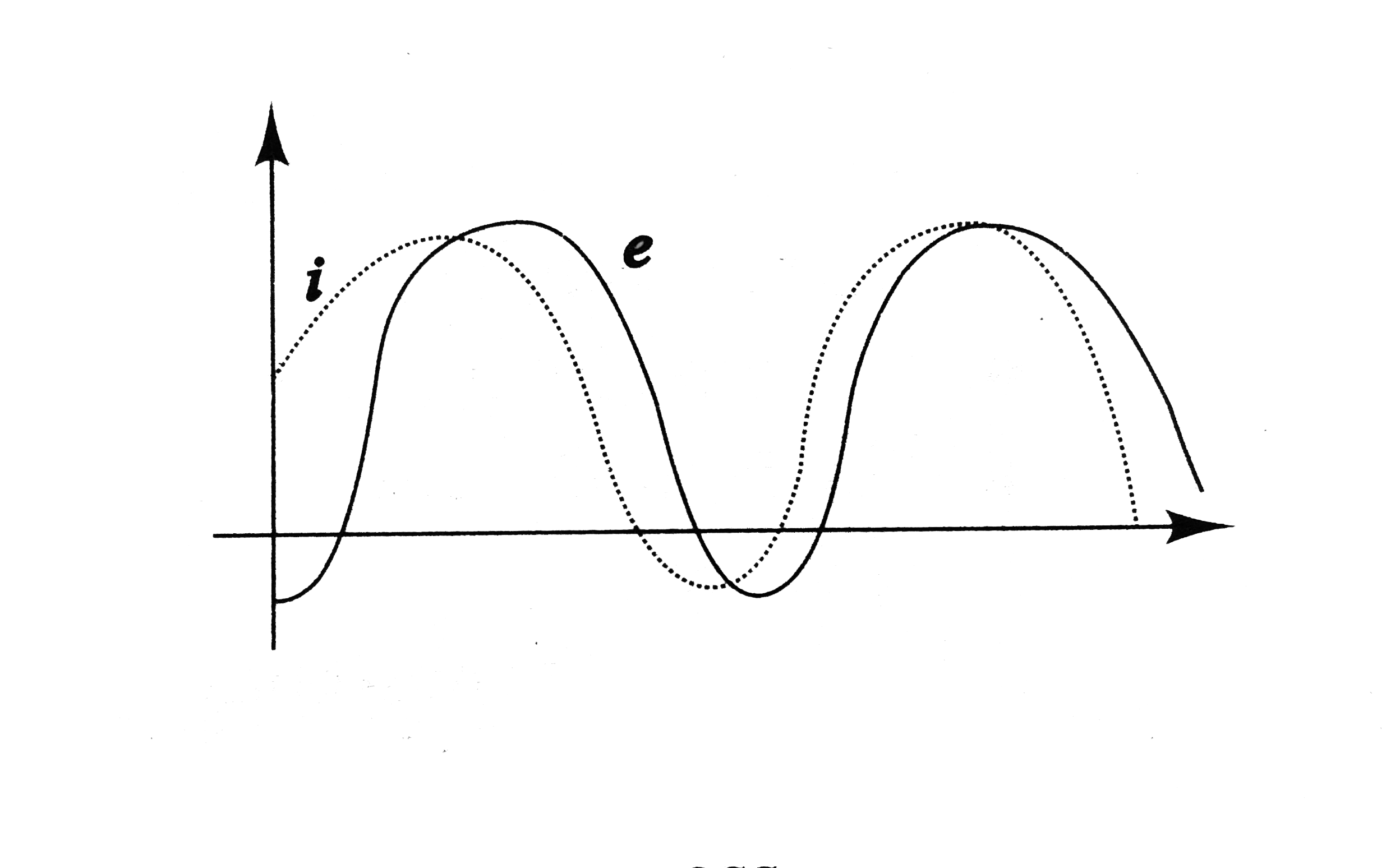
View SolutionWhen an ac source of emfe=E0sin(100t) is connected across a circuit, the phase difference between emf e and currnet I in the circuit is observed to be (π)/(4) as shown in fig. If the circuit consists possibly only of R-C or R-C of L-R series, find the relationship find the relationship between the two elements.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
When an ac source of emfe=E0sin(100t) is connected across a circuit, the phase difference between emf e and currnet I in the circuit is observed to be (π)/(4) as shown in fig. If the circuit consists possibly only of R-C or R-C of L-R series, find the relationship find the relationship between the two elements.
 AR=1kΩ,C=10μFBR=1kΩ,C=1μFCR=1kΩ,L=10mHDR=10kΩ,L=10mH
AR=1kΩ,C=10μFBR=1kΩ,C=1μFCR=1kΩ,L=10mHDR=10kΩ,L=10mH
The phase diffenernce between the alternating current and emf is π2. Which of the following cannot be the constiuent of the circuit?
View SolutionCan you have an AC series circuit in which there is a phase difference of (a) 1800 (b) 1200 between the emf and the current?
View SolutionA 25(μ)F capacitor, a 0.1 H inductor and a 25Ω resistor are connected in series with an ac source of emf E=310sin314t. Find
(a) the frequency of the emf
(b) the impedance of the circuit
(c) the current in the circuit.
(d) the phase angleView SolutionAn alternating emf 200 volts at 50Hz is connected to a circuit resistance 1ohm and inductance 0.01H. What is the phase difference between the current and the emf in the circuit? Also, find the current in the circuit.
View SolutionA seriesLCR circuit is connected to an ac voltage source. When L is removed from the circuit, the phase difference between current and voltage is π3. If instead C is removed from the circuit phase difference is again π3 between current and voltage. Power factor of circuit is:
View SolutionA 200Ω resistor and 1H inductor are joined in series with an ac source of emf 10√2sin(200t)V. Calculate the phases difference between emf and current.
View SolutionIn an L-R circuit connected to a battery of constant emf E, switch is closed at time t=0. If e denotes the induced emf across inductor and I the current in the circuit at any time t. Then which of the following graphs shown the variation of e with i?
View Solution

