A projectile is projected upward with speed 2m/s on an incline plane of inclination 30∘ at an angle of 15∘ from the plane. Then the distance along the plane where projectile will fall is :
20 cm
18 cm
26
14 cm
The correct Answer is:A
To solve the problem of finding the distance along the inclined plane where the projectile will fall, we can use the formula for the range of a projectile on an inclined plane. Here's a step-by-step solution:
Step 1: Identify the parameters
- Initial speed u=2m/s
- Angle of inclination of the plane β=30∘
- Angle of projection from the plane α=15∘
- Acceleration due to gravity g=10m/s2
Step 2: Use the range formula for projectile motion on an inclined plane
The formula for the range R along the inclined plane is given by:
R=2u2sinαcos(α+β)gcos2β
Step 3: Calculate sinα and cos(α+β)
- sin15∘ can be calculated using the sine value:
sin15∘=√6−√24
- α+β=15∘+30∘=45∘
- Thus, cos45∘=1√2
Step 4: Calculate cos2β
- cos30∘=√32
- Therefore, cos230∘=(√32)2=34
Step 5: Substitute the values into the range formula
Now substituting the values into the range formula:
R=2⋅(22)⋅sin15∘⋅cos45∘10⋅cos230∘
=2⋅4⋅sin15∘⋅1√210⋅34
=8⋅sin15∘⋅1√210⋅34=8⋅sin15∘⋅4310
=32⋅sin15∘30
Step 6: Calculate sin15∘ and finalize the calculation
Using sin15∘≈0.2588:
R=32⋅0.258830≈8.281630≈0.276m
Step 7: Convert to centimeters
To convert to centimeters:
R≈0.276×100≈27.6cm
Final Answer
The distance along the plane where the projectile will fall is approximately 27.6 cm.
---
- Initial speed u=2m/s
- Angle of inclination of the plane β=30∘
- Angle of projection from the plane α=15∘
- Acceleration due to gravity g=10m/s2
The formula for the range R along the inclined plane is given by:
R=2u2sinαcos(α+β)gcos2β
- sin15∘ can be calculated using the sine value:
sin15∘=√6−√24
- α+β=15∘+30∘=45∘
- Thus, cos45∘=1√2
- cos30∘=√32
- Therefore, cos230∘=(√32)2=34
Now substituting the values into the range formula:
R=2⋅(22)⋅sin15∘⋅cos45∘10⋅cos230∘
=2⋅4⋅sin15∘⋅1√210⋅34
=8⋅sin15∘⋅1√210⋅34=8⋅sin15∘⋅4310
=32⋅sin15∘30
Using sin15∘≈0.2588:
R=32⋅0.258830≈8.281630≈0.276m
To convert to centimeters:
R≈0.276×100≈27.6cm
The distance along the plane where the projectile will fall is approximately 27.6 cm.
Topper's Solved these Questions
Similar Questions
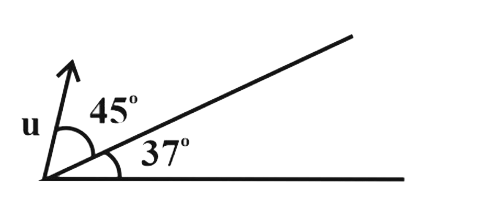
A particle is projected on a frictionless inclined plane of inclination 37∘ at an angle of projection 45∘ from the inclined plane as shown in the figure. If after the first collision from the plane, the particle returns to its point of projection, then what is the value of the reciprocal of the coefficient of restitution between the particle and the plane?
View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘ with velocity of 40m/s at an angle of 60∘ with horizontal. Find the speed of the particle when its velocity vector is parallel to the plane. Take g=10m/s2.
View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘. At what angle α (from the horizontal) should the particle be projected to get the maximum range on the inclined plane.
View SolutionA ball is projected from the bottom of an inclined plane of inclination 30∘, with a velocity of 30ms−1, at an angle of 30∘ with the inclined plane. If g=10ms−2 , then the range of the ball on given inclined plane is
View SolutionThe maximum range of a projectile is 500m. If the particle is thrown up a plane is inclined at an angle of 30∘ with the same speed, the distance covered by it along the inclined plane will be:
View SolutionA projectile si thrown with velocity of 50m/s towards an inclined plane from ground such that is strikes the inclined plane perpendiclularly. The angle of projection of the projectile is 53∘ with the horizontal and the inclined plane is inclined at an angle of 45∘ to the horizonta..

(a) Find the time of flight.
(b) Find the distance between the point of projection and the foot of inclined plane.
View SolutionA ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
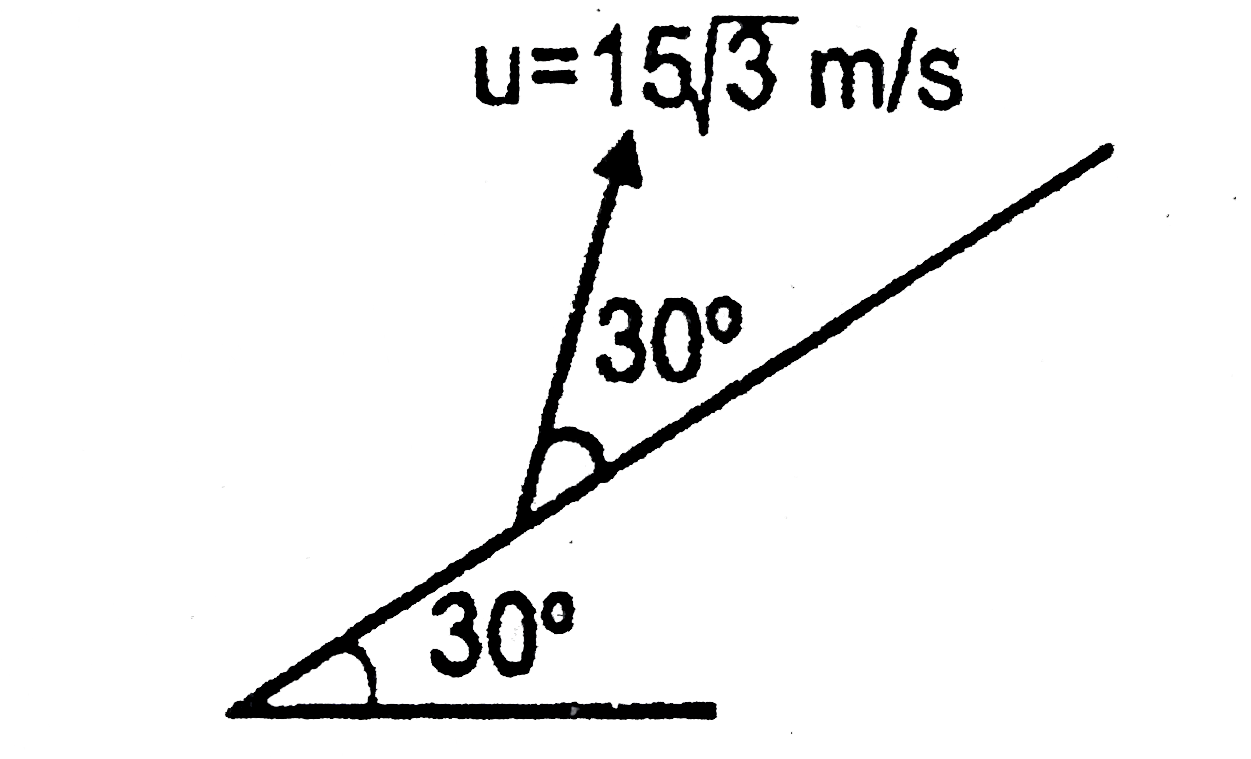
View SolutionA partile is projected up an incline (inclination angle =30∘) with 15√3m/s at an angle of 30∘ with the incline (as shown in figure) (g=10m/s2)
View SolutionA projectile is fired with a velocity v at right angle to the slope inclined at an angle θ with the horizontal. The range of the projectile along the inclined plane is

View SolutionA thin uniform circular ring is rolling down an inclined plane of inclination 30∘ without slipping. Its linear acceleration along the inclined plane will be
View Solution
