A ball is projected from the bottom of an inclined plane of inclination 30∘, with a velocity of 30ms−1, at an angle of 30∘ with the inclined plane. If g=10ms−2 , then the range of the ball on given inclined plane is
12 m
60 m
120 m
600 m
The correct Answer is:B
To solve the problem of finding the range of a ball projected from the bottom of an inclined plane, we can follow these steps:
1. Identify the Given Values:
- Angle of inclination of the plane, θ=30∘
- Initial velocity of the ball, v=30m/s
- Acceleration due to gravity, g=10m/s2
- Angle of projection with respect to the inclined plane, α=30∘
2. Resolve the Initial Velocity:
- The component of the initial velocity along the inclined plane is given by:
valong=vcosα=30cos30∘=30×√32=15√3m/s
3. Determine the Vertical Component of Gravity:
- The effective acceleration due to gravity acting down the incline is:
geffective=gsinθ=10sin30∘=10×12=5m/s2
4. Use the Range Formula for Projectile Motion on an Incline:
- The range R on an inclined plane can be calculated using the formula:
R=v2alonggeffectivesin(2α)
- Here, sin(2α)=sin(60∘)=√32.
5. Substituting the Values:
- Substitute valong and geffective into the range formula:
R=(15√3)25⋅√32
- Calculate (15√3)2=675:
R=6755⋅√32=135⋅√32=67.5√3m
6. Final Calculation:
- Approximating √3≈1.732:
R≈67.5×1.732≈116.5m
Conclusion:
The range of the ball on the inclined plane is approximately 116.5m.
- Angle of inclination of the plane, θ=30∘
- Initial velocity of the ball, v=30m/s
- Acceleration due to gravity, g=10m/s2
- Angle of projection with respect to the inclined plane, α=30∘
- The component of the initial velocity along the inclined plane is given by:
valong=vcosα=30cos30∘=30×√32=15√3m/s
- The effective acceleration due to gravity acting down the incline is:
geffective=gsinθ=10sin30∘=10×12=5m/s2
- The range R on an inclined plane can be calculated using the formula:
R=v2alonggeffectivesin(2α)
- Here, sin(2α)=sin(60∘)=√32.
- Substitute valong and geffective into the range formula:
R=(15√3)25⋅√32
- Calculate (15√3)2=675:
R=6755⋅√32=135⋅√32=67.5√3m
- Approximating √3≈1.732:
R≈67.5×1.732≈116.5m
The range of the ball on the inclined plane is approximately 116.5m.
Similar Questions
A particle is projected from the bottom of an inclined plane of inclination 30∘ with velocity of 40m/s at an angle of 60∘ with horizontal. Find the speed of the particle when its velocity vector is parallel to the plane. Take g=10m/s2.
View SolutionA cylinder rolls down an inclined plane of inclination 30∘, the acceleration of cylinder is
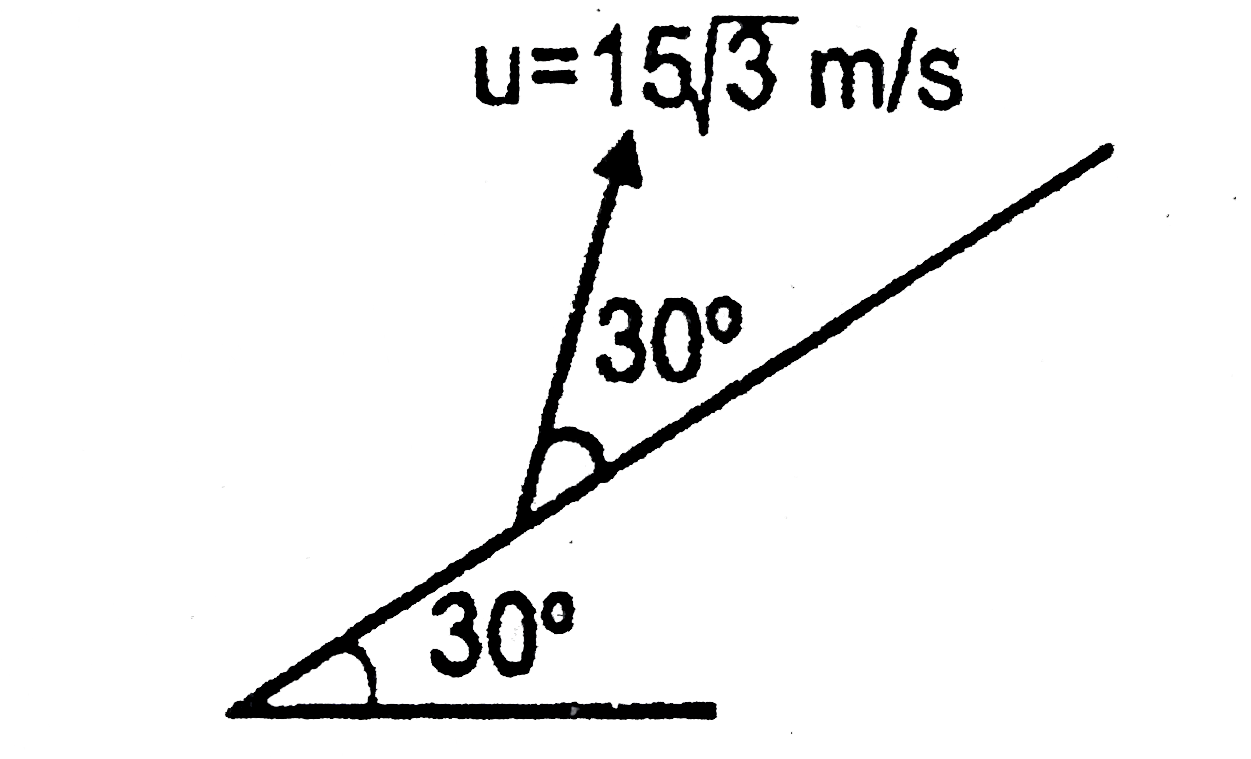
View SolutionA partile is projected up an incline (inclination angle =30∘) with 15√3m/s at an angle of 30∘ with the incline (as shown in figure) (g=10m/s2)
View SolutionA ball is projected from point A with velocity 10ms−1 perpendicular to the inclined plane as shown in figure. Range of the ball on the inclined plane is

View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘. At what angle α (from the horizontal) should the particle be projected to get the maximum range on the inclined plane.
View SolutionA bullet is fired from the bottom of the inclined plane at angle θ=37∘ with the inclined plane. The angle of incline is 30∘ with the horizontal. Find the
(a) position of the maximum height of the bullet from the inclined plane ,
(b) time of light ,
( c) range along the incline ,
(d) the value of θ at which the range will be maximum ,
( e) maximum range.
View Solution
A particle of mass m is projected up from the bottom of an inclined plane with initial velocity v0 at angle 45∘ with an inclined plane of inclination 30∘ as shown in figure. At the same time a small block of same mass m is released from rest at a height h, the particle hits the block at some point on the inclined plane. Neglect friction at inclined.
Q. The value of height h is
View SolutionA body is projected from the bottom of a smooth inclined place with a velocity of 20ms−1, If it is just sufficient to carry it to the top in 4s, find the inclination and height of the plane.
View SolutionA block starts moving up a fixed inclined plane of inclination 60∘C with a velocityof 20ms−1 and stops after 2s. The approximate value of the coefficient of friction is [g=10ms−2]
View SolutionA particle is fired horizontally form an inclined plane of inclination 30∘ with horizontal with speed 50ms−1. If g=10ms−2 , the range measured along the incline is
View Solution
