A mass of 10 kg is suspended vertically by a rope from the roof. When a horizontal force is applied on the rope at some point, the rope deviated at an angle of 45∘ at the roof point. If the suspended mass is at equilibrium, the magnitude of the force applied is (g=10ms−2)
200 N
140 N
70 N
100 N
To solve the problem, we need to analyze the forces acting on the mass that is suspended by a rope when a horizontal force is applied, causing the rope to deviate at an angle of 45 degrees.
1. Identify the Forces Acting on the Mass:
- The weight of the mass (W) acting downward:
W=mg
- The tension (T) in the rope acting along the rope at an angle of 45 degrees from the vertical.
- The horizontal force (F) applied at the point of the rope.
2. Calculate the Weight of the Mass:
- Given:
- Mass (m) = 10 kg
- Acceleration due to gravity (g) = 10 m/s²
- Therefore, the weight (W) is:
W=mg=10kg×10m/s2=100N
3. Resolve the Tension into Components:
- The tension (T) in the rope can be resolved into two components:
- Vertical component (T_y) = Tcos(45∘)
- Horizontal component (T_x) = Tsin(45∘)
4. Set Up the Equilibrium Conditions:
- Since the mass is in equilibrium, the sum of the vertical forces must equal zero:
Tcos(45∘)=W
- The horizontal force applied (F) must balance the horizontal component of the tension:
F=Tsin(45∘)
5. Substituting the Values:
- From the vertical equilibrium condition:
Tcos(45∘)=100N
- Since cos(45∘)=1√2:
T⋅1√2=100⟹T=100√2N
6. Calculate the Horizontal Force (F):
- Substitute T into the horizontal force equation:
F=Tsin(45∘)=100√2⋅1√2=100N
Final Answer:
The magnitude of the horizontal force applied is:
F=100N
- The weight of the mass (W) acting downward:
W=mg
- The tension (T) in the rope acting along the rope at an angle of 45 degrees from the vertical.
- The horizontal force (F) applied at the point of the rope.
- Given:
- Mass (m) = 10 kg
- Acceleration due to gravity (g) = 10 m/s²
- Therefore, the weight (W) is:
W=mg=10kg×10m/s2=100N
- The tension (T) in the rope can be resolved into two components:
- Vertical component (T_y) = Tcos(45∘)
- Horizontal component (T_x) = Tsin(45∘)
- Since the mass is in equilibrium, the sum of the vertical forces must equal zero:
Tcos(45∘)=W
- The horizontal force applied (F) must balance the horizontal component of the tension:
F=Tsin(45∘)
- From the vertical equilibrium condition:
Tcos(45∘)=100N
- Since cos(45∘)=1√2:
T⋅1√2=100⟹T=100√2N
- Substitute T into the horizontal force equation:
F=Tsin(45∘)=100√2⋅1√2=100N
The magnitude of the horizontal force applied is:
F=100N
Similar Questions
A mass of 6 kg is suspended by a rope of length 2m from a celling. A force of 50N is applied in horizontal direction at the mid point of the rope. What is the angle of the rope with the verticle in equilibrium:-
View SolutionA 10 kg body is suspended by a rope is pulled by means of a horizontal force to make 60° by rope to vertical. The horizontal force is
View Solution
Knowledge Check
A block fof mass 5kg is suspended by a massless rope of length 2 m from the ceilling. A force of 50 N is applied in the horizontal direction at the midpoint P of the rope, as shown in the figure. The angle made by the rope with the vertical in equilibrium is (Take g=10ms−2m .
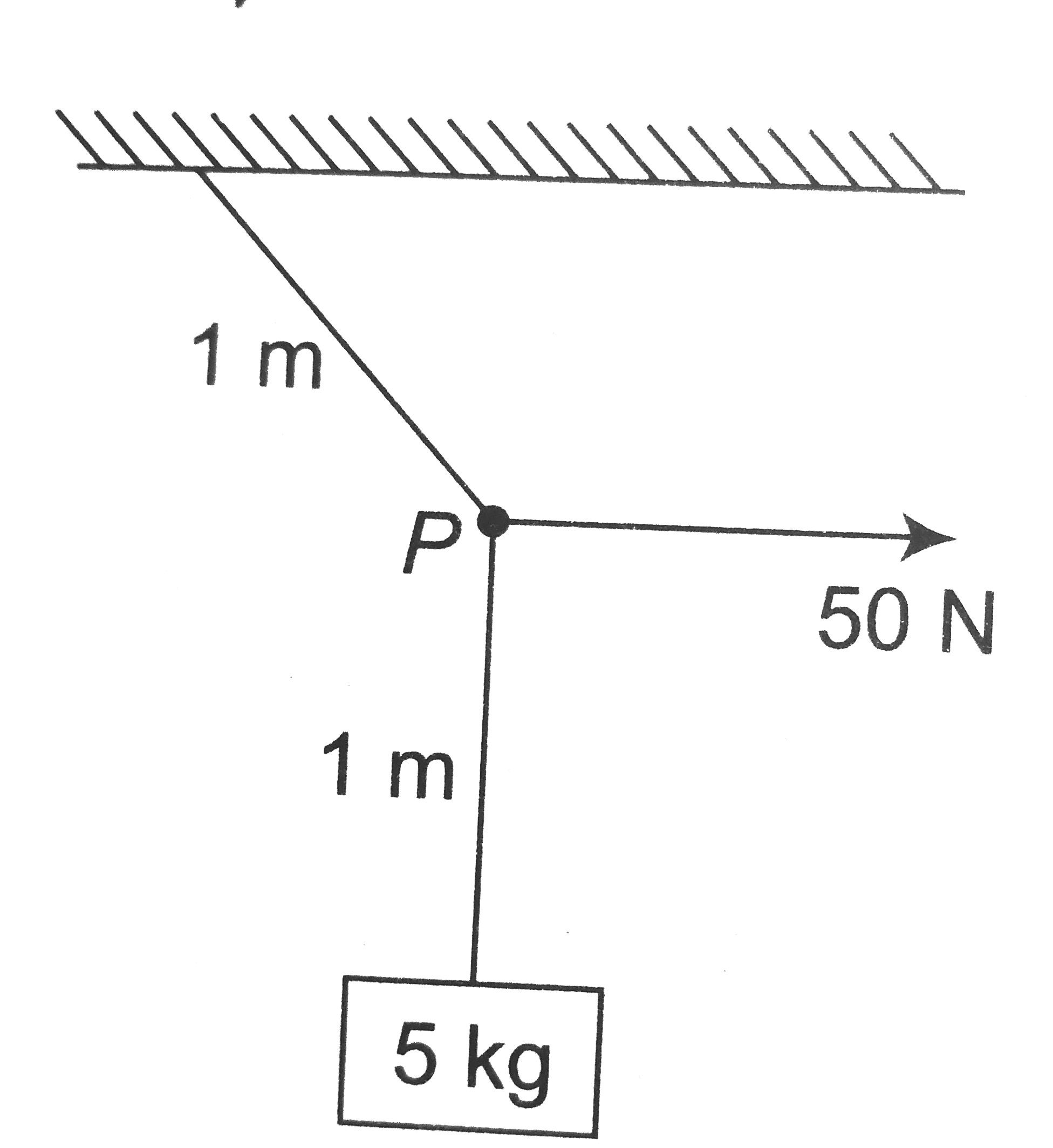 A30∘B40∘C60∘D45∘
A30∘B40∘C60∘D45∘
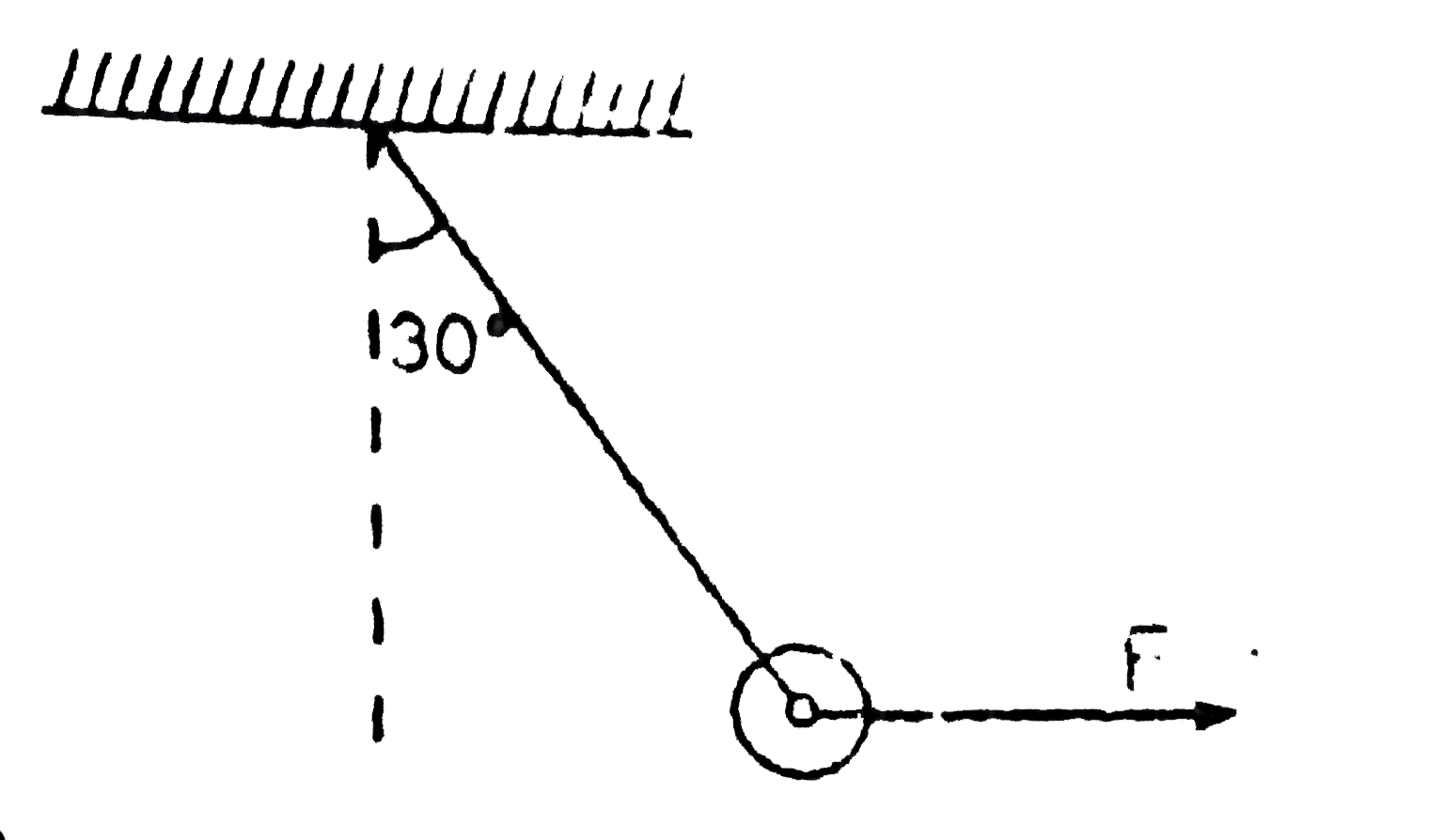
A heavy particle of mass 1kg suspended from a massless string attached to a roof. A horizontal force F is applied to the particle such that in the equilibrium position the string makes and angle 30∘ with the vertical. The magnitude of the force F equals
 .
.
View SolutionA mass of M kg is suspended by a weightless string. The horizontal force required to displace it until string makes an angle of 45∘ with the initial vertical direction is:
View SolutionA mass M kg is suspended by a weightless string. The horizontal force required to hold the mass at 60∘ with the vertical is
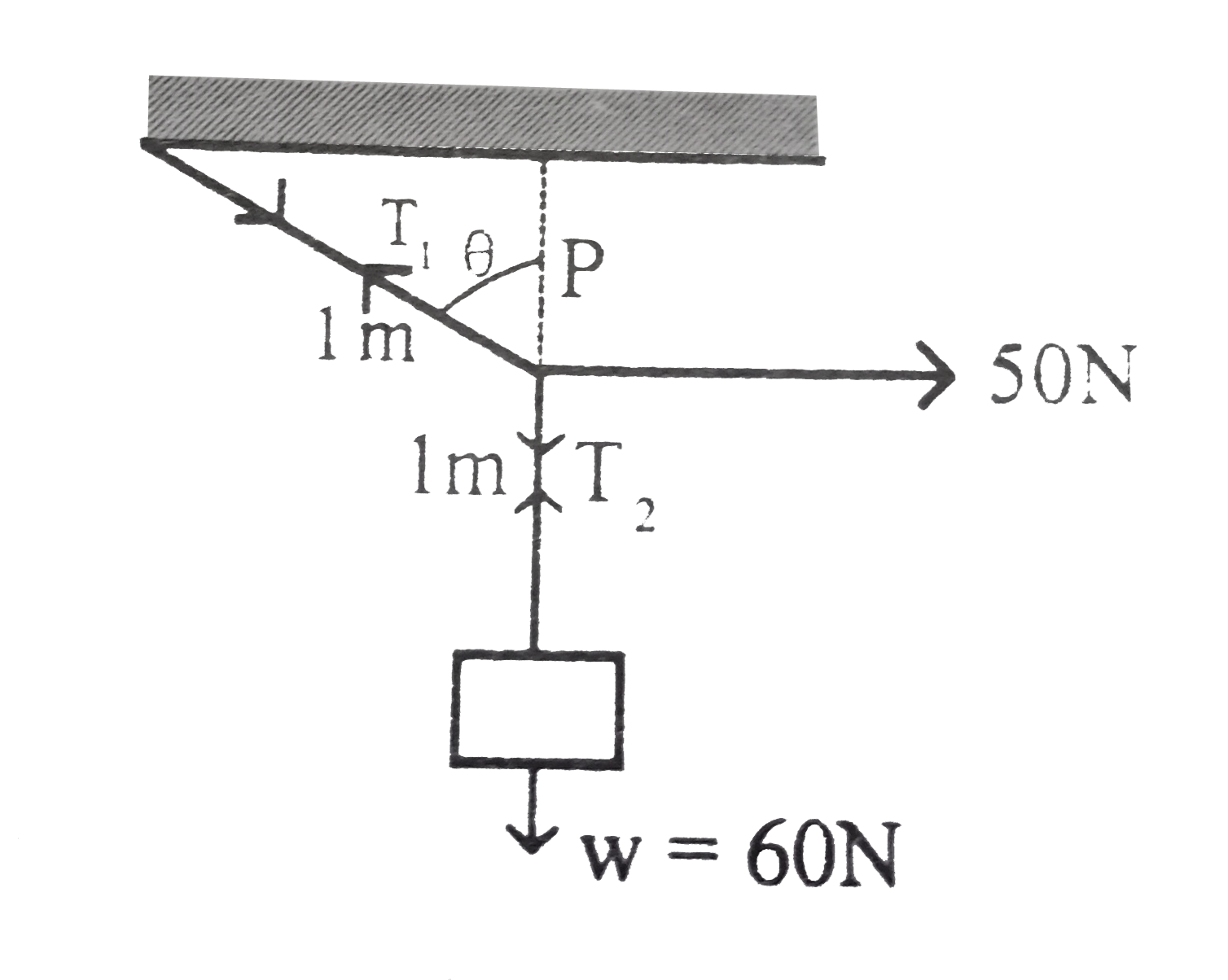
View SolutionSee Fig 5.8 .A mass of 6 kg is suspended by a rope of length 2m from the ceiling . A force of 50 N in the horizontal direction is applied at the mid-point P of the rope, as shown. What is the angle the rope makes with the vertical in equilibrium ? (Take g = 10 m s−2). Neglect the mass of the rope.
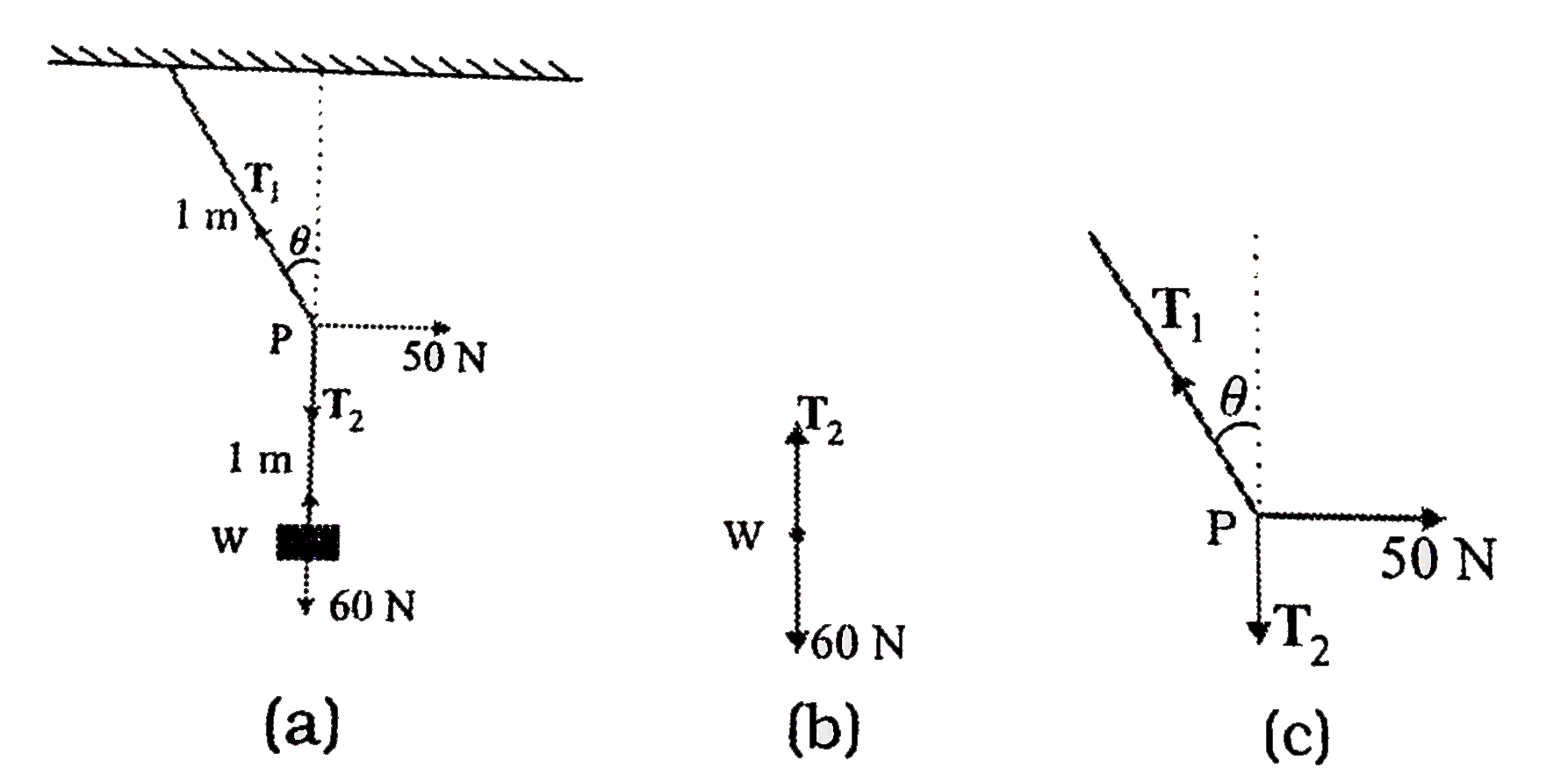
View SolutionA mass of 6 kg is suspended by a rope of length 2 m from the ceilling A force of 50 N in the horizontal direction is applied at the mid - point P of the rope as shown . What is the angle the rope makes with the vertical in equilibrium ? (Take = 10ms−2) . Neglect mass of the rope .
View Solution
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass m3 moving in vertical up wards direction with velocity v0 strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The time taken by the block bullet system to move from y=mgk (initial equilibrium position) to y=0 (natural length of spring) is (A represents the amplitude of motion)
View SolutionA block of mass Mis pulled vertically upward through a rope of mass m by applying force F on one end of the rope. What force does the rope exert on the block?
View Solution
