Let there be a spherically symmetric charge distribution with charge density varying as upto , and for , where r is the distance from the origin. The electric field at a distance r(rltR) from the origin is given by
The correct Answer is:B
To find the electric field at a distance r (where r<R) from the origin for a spherically symmetric charge distribution with the given charge density, we can follow these steps:
Step 1: Define the charge density
The charge density is given as:
ρ(r)=ρ(54−rR)for r<R
and ρ(r)=0 for r≥R.
Step 2: Calculate the volume of a thin spherical shell
Consider a thin spherical shell of radius r and thickness dr. The volume dV of this shell is given by:
dV=4πr2dr
Step 3: Calculate the charge in the thin shell
The charge dq in the thin shell can be expressed as:
dq=ρ(r)⋅dV=ρ(54−rR)⋅4πr2dr
Step 4: Calculate the total charge enclosed within radius r
To find the total charge Qenclosed within a radius r, we integrate dq from 0 to r:
Qenclosed=∫r0dq=∫r0ρ(54−r′R)4π(r′)2dr′
Here, r′ is a dummy variable for integration.
Step 5: Perform the integration
Substituting dq into the integral:
Qenclosed=4πρ∫r0(54(r′)2−r′R(r′)2)dr′
This simplifies to:
Qenclosed=4πρ(54⋅r33−1R⋅r44)
=4πρ12⋅5r3−4πρ4Rr4
=5πρr33−πρr4R
Step 6: Apply Gauss's Law
According to Gauss's Law:
∮E⋅dA=Qenclosedϵ0
The left-hand side becomes:
E⋅4πr2
Thus, we have:
E⋅4πr2=1ϵ0(5πρr33−πρr4R)
Step 7: Solve for the electric field E
Rearranging gives:
E=14πϵ0r2(5πρr33−πρr4R)
=ρ4ϵ0(5r3−r2R)
Final Expression
Thus, the electric field at a distance r from the origin is:
E=ρ4ϵ0(5r3−r2R)
The charge density is given as:
ρ(r)=ρ(54−rR)for r<R
and ρ(r)=0 for r≥R.
Consider a thin spherical shell of radius r and thickness dr. The volume dV of this shell is given by:
dV=4πr2dr
The charge dq in the thin shell can be expressed as:
dq=ρ(r)⋅dV=ρ(54−rR)⋅4πr2dr
To find the total charge Qenclosed within a radius r, we integrate dq from 0 to r:
Qenclosed=∫r0dq=∫r0ρ(54−r′R)4π(r′)2dr′
Here, r′ is a dummy variable for integration.
Substituting dq into the integral:
Qenclosed=4πρ∫r0(54(r′)2−r′R(r′)2)dr′
This simplifies to:
Qenclosed=4πρ(54⋅r33−1R⋅r44)
=4πρ12⋅5r3−4πρ4Rr4
=5πρr33−πρr4R
According to Gauss's Law:
∮E⋅dA=Qenclosedϵ0
The left-hand side becomes:
E⋅4πr2
Thus, we have:
E⋅4πr2=1ϵ0(5πρr33−πρr4R)
Rearranging gives:
E=14πϵ0r2(5πρr33−πρr4R)
=ρ4ϵ0(5r3−r2R)
Thus, the electric field at a distance r from the origin is:
E=ρ4ϵ0(5r3−r2R)
Topper's Solved these Questions
Similar Questions
Let there be a spherical symmetric charge density varying as p(r)=p0rR upto r = R and ρ(r)=0 for r>R, where r is the distance from the origin. The electric field at on a distance r(r<R) from the origin is given by -
View SolutionA point charge q is placed at the origin . How does the electric field due to the charge very with distance r from the origin ?
View SolutionA solid non-conduction cylinder of radius R is charge such that volume charge density is proporation to r where r is distance from axis. The electric field E at a distance r(r<R) well depend on r as.
View SolutionA hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
View SolutionThe mass density of a spherical body is given by ρ(r)=kr for r≤R and ρ(r)=0for r > R , where r is the distance from the centre. The correct graph that describes qualitatively the acceleration, a, of a test particle as a function of r is :
View SolutionIn a spherical distribution , the charge density varies as ρ(r)=A/r for a<r<b (as shown) where A is constant . A point charge Q lies at the centre of the sphere at r = 0 . The electric filed in the region a<r<b has a constant magnitude for
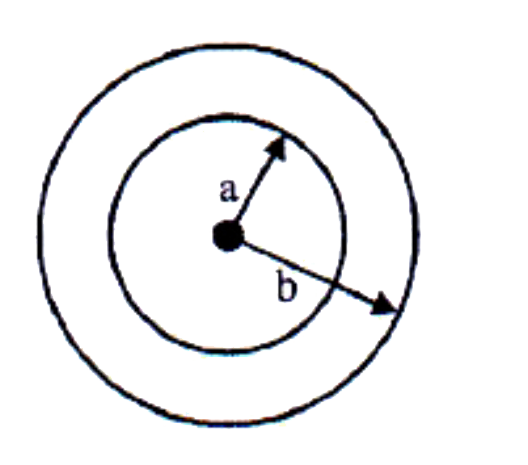
View SolutionFor spherically symmetrical charge distribution, electric field at a distance r from the centre of sphere is →E=kr7→r, where k is a constant. What will be the volume charge density at a distance r from the centre of sphere?
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionA solid sphere of radius R has a charge Q distributed in its volume with a charge density ρ=kra, where k and a are constants and r is the distance from its centre. If the electric field at r=R2 is 18 times that r=R, find the value of a.
View SolutionA non-conducting solid sphere has volume charge density that varies as ρ=ρ0 r, where ρ0 is a constant and r is distance from centre. Find out electric field intensities at following positions.
(i) r<R (ii) r≥R
View Solution
