Consider a spherical shell of radius R at temperature T. The black body radiation inside it can be considered as an ideal gas of photons with internal energy per unit volume u=UV∝T4 and pressure P=13(UV). If the shell now undergoes an adiabatic expansion the relation between T and R is :
T∝e−3R
T∝1R
T∝1R3
T∝e−R
The correct Answer is:B
To solve the problem, we need to find the relationship between the temperature T and the radius R of a spherical shell undergoing adiabatic expansion, given the properties of black body radiation inside it.
1. Understanding the Internal Energy and Pressure:
- The internal energy per unit volume u is given as u=kT4, where k is a proportionality constant.
- The pressure P is given by P=13UV=13u.
2. Expressing Pressure in Terms of Temperature:
- From the internal energy expression, we can write:
P=13kT4
- This is our equation (3).
3. Using the Ideal Gas Law:
- For an ideal gas, the relationship between pressure, volume, and temperature is given by:
PV=nRT
- Assuming we have 1 mole of gas (n = 1), we can rewrite this as:
P=RTV
- This is our equation (4).
4. Equating the Two Expressions for Pressure:
- From equations (3) and (4), we can set them equal to each other:
RTV=13kT4
5. Rearranging the Equation:
- Rearranging gives:
3RT=kT4⋅V
- Thus, we can express V in terms of T:
V=3Rk⋅TT4=3Rk⋅1T3
6. Volume of the Spherical Shell:
- The volume V of a spherical shell of radius R is given by:
V=43πR3
7. Setting the Two Volume Expressions Equal:
- Now we can set the two expressions for volume equal to each other:
43πR3=3Rk⋅1T3
8. Simplifying the Equation:
- Rearranging gives:
T3=3Rk⋅43πR3
- Thus, we can express T3 in terms of R3:
T3∝R3
9. Finding the Relationship Between T and R:
- From the above, we can conclude that:
T∝1R
- Therefore, the relationship between temperature T and radius R during adiabatic expansion is:
T∝1R
Final Result:
The relation between temperature T and radius R is:
T∝1R
- The internal energy per unit volume u is given as u=kT4, where k is a proportionality constant.
- The pressure P is given by P=13UV=13u.
- From the internal energy expression, we can write:
P=13kT4
- This is our equation (3).
- For an ideal gas, the relationship between pressure, volume, and temperature is given by:
PV=nRT
- Assuming we have 1 mole of gas (n = 1), we can rewrite this as:
P=RTV
- This is our equation (4).
- From equations (3) and (4), we can set them equal to each other:
RTV=13kT4
- Rearranging gives:
3RT=kT4⋅V
- Thus, we can express V in terms of T:
V=3Rk⋅TT4=3Rk⋅1T3
- The volume V of a spherical shell of radius R is given by:
V=43πR3
- Now we can set the two expressions for volume equal to each other:
43πR3=3Rk⋅1T3
- Rearranging gives:
T3=3Rk⋅43πR3
- Thus, we can express T3 in terms of R3:
T3∝R3
- From the above, we can conclude that:
T∝1R
- Therefore, the relationship between temperature T and radius R during adiabatic expansion is:
T∝1R
The relation between temperature T and radius R is:
T∝1R
Topper's Solved these Questions
Similar Questions
The internal energy of an ideal gas is sum of total kinetic energy of molecules. Consider an ideal gas in which the relation among U, P and V is U=2+3PVThe gas is
View SolutionAn ideal gas with adiabatic exponent γ=43 undergoes a process in which internal energy is related to volume as U=V2 . Then molar heat capacity of the gas for the process is :
View Solution
Knowledge Check
The relation between internal energy U, pressure P and volume V of a gas in an adiabatic process is
U=a+bPV where a and b are constants. What is the effective value of adiabatic constant γ ?A1. abB2. b+1bC3. a+1aD4. ba
The value of γ=CP/CV is 4/3 for an adaibatic process of an ideal gas for which internal energy U=K+nPV. Find the value of n (K is constant) :
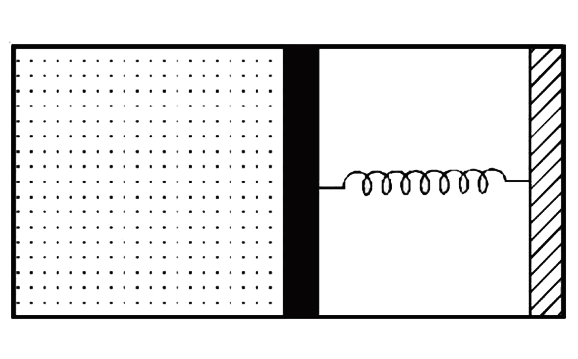
View SolutionAn ideal monoatomic gas is confined in a horizontal cylinder by a spring loaded piston (as shown in the figure). Initially the gas is at temperature T1, pressure P1 and volume V1and the spring is in its relaxed state. The gas is then heated very slowly to temperature T2,pressure P2and volume V2. During this process the piston moves out by a distance x. Ignoring the friction between the piston and the cylinder, the correct statement (s) is (are)
View SolutionIn the given pressure-temperature (P−T) diagram, what is the relation between volumes V1 and V2 for a fixed mass of a gas?
View SolutionAt pressure P and absolute temperature T a mass M of an ideal gas fills a closed container of volume V. An additional mass 2M of the same gas is added into the container and the volume is then reduced to V3 and the temperature to T3. The pressure of the gas will now be:
View SolutionThe temperature of an ideal gas undergoing adiabatic expansion varies with volume as T∝V−34, then the value of CPCV for the gas is
View SolutionThe relation between internal energy U, pressure P and volume V of a gas in an adiabatic processes is : U=a+bPV where a = b = 3 . Calculate the greatest integer of the ratio of specific heats [γ] .
View SolutionWhen a sample of ideal gas is changed from an initial state to a final state, various curves can be plotted for the process like P-V curve, V-T curve,P-T curve etc.
For example, P-V curve for a fixed amount of an ideal gas at constant temperature is a rectangular hyperbola, V-T curve for a fixed amount of an ideal gas at constant volume is again a straight line. However, the shapes may vary if the constant parameters are also changed.
Now, answer the following questions :
From the given plot between Z and P for a real gas the correct is :

View Solution
