A rod PQ of length L revolves in a horizontal plane about the axis YY´. The angular velocity of the rod is w. If A is the area of cross-section of the rod and ρ be its density, its rotational kinetic energy is
134AL3ρω2
12AL3ρω2
124AL3ρω2
18AL3ρω2
The correct Answer is:C
To find the rotational kinetic energy of a rod PQ of length L revolving about an axis YY' with an angular velocity ω, we can follow these steps:
Step 1: Understand the formula for rotational kinetic energy
The rotational kinetic energy (K.E.) of a rotating body is given by the formula:
K.E.=12Iω2
where I is the moment of inertia of the body and ω is the angular velocity.
Step 2: Determine the moment of inertia of the rod
For a uniform rod of length L rotating about an axis through its center, the moment of inertia I is given by:
I=112mL2
where m is the mass of the rod.
Step 3: Calculate the mass of the rod
The mass m of the rod can be expressed in terms of its density ρ and cross-sectional area A:
m=Volume×Density=A⋅L⋅ρ
Thus, substituting this into the moment of inertia equation gives:
I=112(A⋅L⋅ρ)L2=112AρL3
Step 4: Substitute the moment of inertia into the kinetic energy formula
Now we can substitute the expression for I back into the kinetic energy formula:
K.E.=12(112AρL3)ω2
Step 5: Simplify the expression
Simplifying the expression gives:
K.E.=124AρL3ω2
Final Result
Thus, the rotational kinetic energy of the rod is:
K.E.=124AρL3ω2
The rotational kinetic energy (K.E.) of a rotating body is given by the formula:
K.E.=12Iω2
where I is the moment of inertia of the body and ω is the angular velocity.
For a uniform rod of length L rotating about an axis through its center, the moment of inertia I is given by:
I=112mL2
where m is the mass of the rod.
The mass m of the rod can be expressed in terms of its density ρ and cross-sectional area A:
m=Volume×Density=A⋅L⋅ρ
Thus, substituting this into the moment of inertia equation gives:
I=112(A⋅L⋅ρ)L2=112AρL3
Now we can substitute the expression for I back into the kinetic energy formula:
K.E.=12(112AρL3)ω2
Simplifying the expression gives:
K.E.=124AρL3ω2
Thus, the rotational kinetic energy of the rod is:
K.E.=124AρL3ω2
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ENABLE
50 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
A uniform rod AB of length 2l and mass m is rotating in a horizontal plane about a vertical axis through A, with angular velocity ω, when the mid-point of the rod strikes a fixed nail and is brought immediately to rest. Find the impulse exerted by the nail.
View SolutionA uniform rod of length l is from rest such that it rotates about a smooth pivot. The angular speed of the rod when it becomes vertical is.
 .
.
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA rod PQ of length l is rotating about end P, with an angular velocity ω. Due to electrifugal forceed the free electrons in the rod move toward the end Q and an emf is created. Find the induced emf.

View SolutionA uniform rod of length L rests on a frictionless horizontal surface. The rod is pivoted about a fixed frictionless axis at one end. The rod is initially at rest. A bulled travelling parallel to the horizontal surface and perpendicular to the rod with speed v strikes the rod at its centre and becomes embedded in it. the mass of the bullet is one-sixth the mass of the rod.
(a). What is the final angular velocity of the rod?
(b). What is the ratio of the kinetic energy of the system after the collision to the kinetic energy of te bullet the o collision?
View SolutionA rod of length L kept on a smooth horizontal surface is pulled along its length by a force F. The area of cross-section is A and Young's modulus is Y. The extension in the rod is

View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View SolutionA rod is rotating with angular velocity ω about axis AB. Find cosθ.

View SolutionA uniform rod of mass m and length l is pivoted smoothly at O. A horizontal force acts at the bottom of the rod.
a. Find the angular velocity of the rod as the function of angle of rotation θ.
b.What is the maximum angular displacement of the rod?
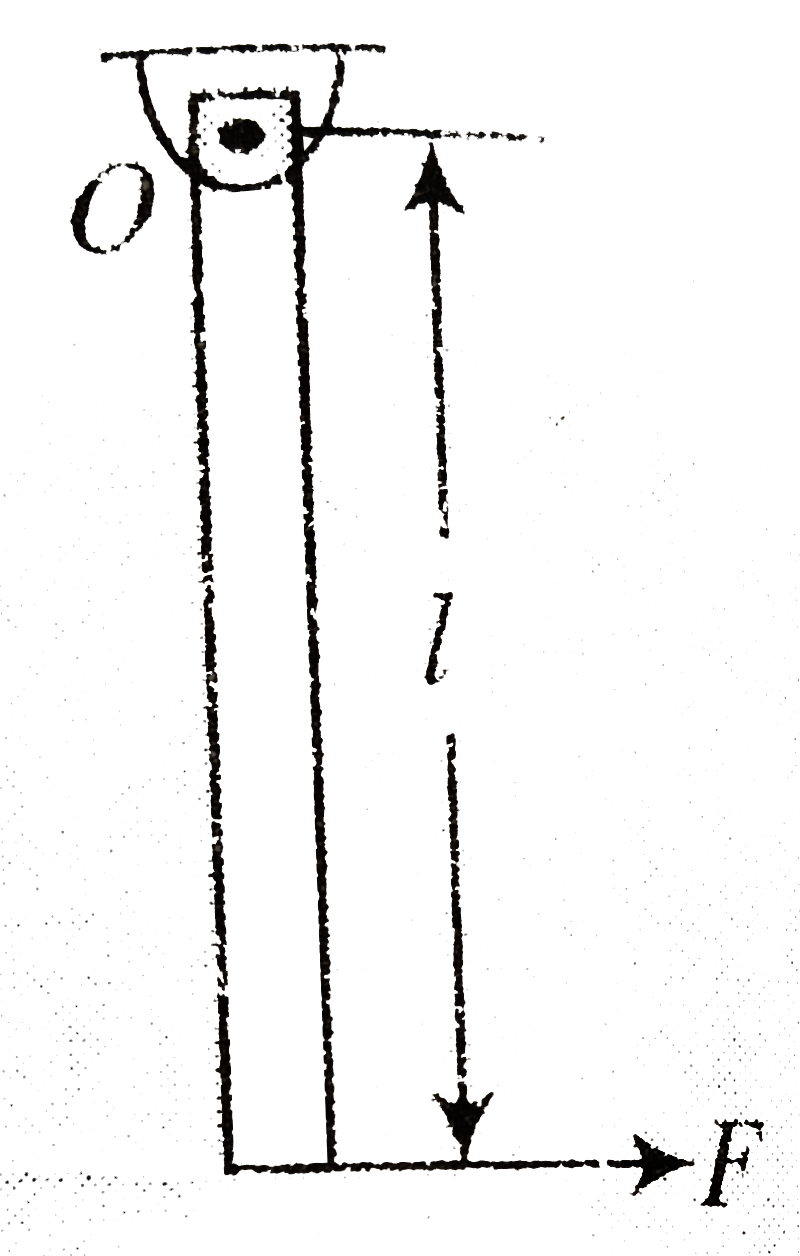
View SolutionA rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View Solution
