An external pressure P is applied on a cube at 0∘C so that is it equally compressed from all sides. K is the bulk modulus o the material of the cube and α is its coefficient of linear expansion. Suppose we want to bring the cube to its original size by heating. the temperature should be raised by:-
The correct Answer is:A
To solve the problem, we need to determine the temperature increase required to restore the original size of a cube that has been compressed under an external pressure P. The bulk modulus K and the coefficient of linear expansion α of the material are given.
1. Understanding Bulk Modulus:
The bulk modulus K is defined as:
K=−PΔVV
where P is the applied pressure, ΔV is the change in volume, and V is the original volume of the cube.
2. Relating Pressure to Volume Change:
Rearranging the formula gives us:
ΔVV=−PK
Since the cube is compressed, ΔV will be negative, hence the negative sign in the equation.
3. Volume Change Due to Temperature Increase:
When the temperature is increased, the volume change ΔV can also be expressed in terms of the coefficient of volume expansion. For a cube, the volume change can be approximated as:
ΔV=V0⋅3αΔθ
where V0 is the original volume, α is the coefficient of linear expansion, and Δθ is the change in temperature.
4. Equating Volume Changes:
Since the volume change due to pressure and temperature must be equal in magnitude (but opposite in sign), we can set the two expressions for ΔV equal to each other:
−PK=3αΔθ
5. Solving for Temperature Change:
Rearranging the equation to solve for Δθ:
Δθ=−P3Kα
Since we are interested in the increase in temperature (which is a positive quantity), we can express it as:
Δθ=P3Kα
Final Answer:
The temperature should be raised by:
Δθ=P3Kα
The bulk modulus K is defined as:
K=−PΔVV
where P is the applied pressure, ΔV is the change in volume, and V is the original volume of the cube.
Rearranging the formula gives us:
ΔVV=−PK
Since the cube is compressed, ΔV will be negative, hence the negative sign in the equation.
When the temperature is increased, the volume change ΔV can also be expressed in terms of the coefficient of volume expansion. For a cube, the volume change can be approximated as:
ΔV=V0⋅3αΔθ
where V0 is the original volume, α is the coefficient of linear expansion, and Δθ is the change in temperature.
Since the volume change due to pressure and temperature must be equal in magnitude (but opposite in sign), we can set the two expressions for ΔV equal to each other:
−PK=3αΔθ
Rearranging the equation to solve for Δθ:
Δθ=−P3Kα
Since we are interested in the increase in temperature (which is a positive quantity), we can express it as:
Δθ=P3Kα
The temperature should be raised by:
Δθ=P3Kα
Topper's Solved these Questions
Similar Questions
An external presseure P is applied on a cube at 273K hence it compresses equally from all sides α is coefficient of linear expansion & K is bulk modulus of material. To bring cube to its original size by heating the temperature rise must be
View SolutionThe pressure applied from all direction on a cube is P. How much its temperature should be raised to maintain the original volume ? The volume elasticity of the cube is β and the coefficient of volume expansion is α
View Solution
Knowledge Check
A cube at temperature 0∘ C is compressed equal from all sides by an external pressure P. BY what amount should its temperature be raised to bring it back to the size it had before the external pressure was applied. The bulk modulus of the material of the cube is K and the coefficient of linear expansion is α.
AP/KαBP/3KαC3πα/KD3K/P
A uniform pressure P is exerted on all sides of a solid cube at temperature t ∘C. By what amount should the temperature of the cube be raised in order to bring its volume back to the value it had before the pressure was applied? The coefficient of volume expansion of cube is α and the bulk modulus is K.
View SolutionA metallic rod l cm long, A square cm in cross-section is heated through t∘C. If Young’s modulus of elasticity of the metal is E and the mean coefficient of linear expansion is α per degree celsius, then the compressional force required to prevent the rod from expanding along its length is
View SolutionThe ends Q and R of two thin wires, PQ and RS, are soldered (joined) together. Initially each of the of wire has a length of 1m at 10∘C. Now the end P is maintained at 10∘C, while the ends S is heated and maintained at 400∘C. The system is thermally insultated from its surroundings. If the thermal conductivity of wire PQ is twice that of the wire RS and the coefficient of linear thermal expansion of PQ is 1.2×10−5K−1, the change in length of the wire PQ is
View SolutionA material has normal density ρ and bulk modulus K. The increase in the density of the material when it is subjected to an external pressure P from all sides is
View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
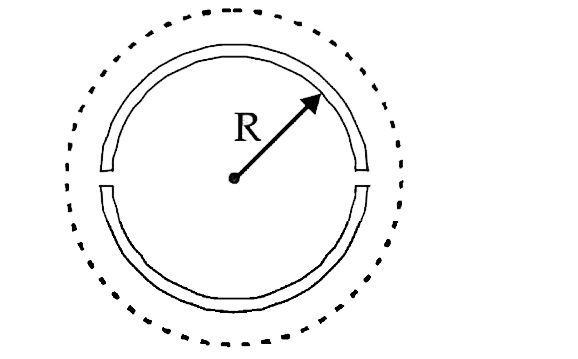
View SolutionA force of 200 N is applied at one end of a wire of length 2m and having area of cross-section 10−2cm2. The other end of the wire is rigidly fixed. If coefficient of linear expansion of the wire α=8×10−6/.∘C and Young's modulus Y=2.2×1011N/m2 and its temperature is increased by 5∘C, then the increase in the tension of the wire will be
View SolutionA uniform pressure p is exerted on all sides of a solid cube of a material at temprature t∘C. By what amount should the temperature of the cube be raised in order to bring its original volume back to the value it had before the pressure was applied ? K is the bulk modulus and α is the coefficient of linear expansion of material of solid cube.
View Solution
