An external presseure P is applied on a cube at 273K hence it compresses equally from all sides α is coefficient of linear expansion & K is bulk modulus of material. To bring cube to its original size by heating the temperature rise must be
P/3αK
P/αK
P/2αK
P/4αK
The correct Answer is:A
To solve the problem, we need to determine the temperature rise required to return a cube to its original size after it has been compressed by an external pressure P. We will use the concepts of bulk modulus and linear expansion.
1. Understanding Bulk Modulus:
The bulk modulus K is defined as:
K=−ΔPΔVV
where ΔP is the change in pressure, ΔV is the change in volume, and V is the original volume.
2. Relating Pressure and Volume Change:
Rearranging the formula gives:
ΔV=−ΔP⋅VK
Here, ΔP=P (the applied pressure).
3. Volume Change in Terms of Linear Expansion:
The change in volume ΔV can also be expressed in terms of the coefficient of linear expansion α and the change in temperature Δθ:
ΔV=γ⋅V⋅Δθ
where γ=3α for a cube (since volume is proportional to the cube of the linear dimensions).
4. Setting the Equations Equal:
From the two expressions for ΔV:
−P⋅VK=3α⋅V⋅Δθ
We can cancel V from both sides (assuming V≠0):
−PK=3αΔθ
5. Solving for Temperature Change:
Rearranging the equation to solve for Δθ:
Δθ=−P3αK
Since we are interested in the magnitude of the temperature rise, we can drop the negative sign:
Δθ=P3αK
Final Answer:
The temperature rise required to bring the cube back to its original size is:
Δθ=P3αK
The bulk modulus K is defined as:
K=−ΔPΔVV
where ΔP is the change in pressure, ΔV is the change in volume, and V is the original volume.
Rearranging the formula gives:
ΔV=−ΔP⋅VK
Here, ΔP=P (the applied pressure).
The change in volume ΔV can also be expressed in terms of the coefficient of linear expansion α and the change in temperature Δθ:
ΔV=γ⋅V⋅Δθ
where γ=3α for a cube (since volume is proportional to the cube of the linear dimensions).
From the two expressions for ΔV:
−P⋅VK=3α⋅V⋅Δθ
We can cancel V from both sides (assuming V≠0):
−PK=3αΔθ
Rearranging the equation to solve for Δθ:
Δθ=−P3αK
Since we are interested in the magnitude of the temperature rise, we can drop the negative sign:
Δθ=P3αK
The temperature rise required to bring the cube back to its original size is:
Δθ=P3αK
Topper's Solved these Questions
Similar Questions
An external pressure P is applied on a cube at 0∘C so that is it equally compressed from all sides. K is the bulk modulus o the material of the cube and α is its coefficient of linear expansion. Suppose we want to bring the cube to its original size by heating. the temperature should be raised by:-
View SolutionA rod, of length L at room tempera ture and uniform area of cross section A, is made of a metal having coefficient of linear expansion α/∘C. It is observed that an external compressive force F, is applied on each its ends, prevents any change in the length of the rod, when its temperature rises by ΔTK. Young's modulus, Y, for this metal is :
View Solution
Knowledge Check
A cube at temperature 0∘ C is compressed equal from all sides by an external pressure P. BY what amount should its temperature be raised to bring it back to the size it had before the external pressure was applied. The bulk modulus of the material of the cube is K and the coefficient of linear expansion is α.
AP/KαBP/3KαC3πα/KD3K/PThe normal density of gold is ρ and its bulk modulus is K. The increase is density of a lump of gold when a pressure P is applied uniformly on all sides is
Aρ P/KBρ K/PCP/ρ KDK/ρP
A rod, of length L at room tempera ture and uniform area of cross section A, is made of a metal having coefficient of linear expansion α/∘C. It is observed that an external compressive force F, is applied on each its ends, prevents any change in the length of the rod, when its temperature rises by ΔTK. Young's modulus, Y, for this metal is :
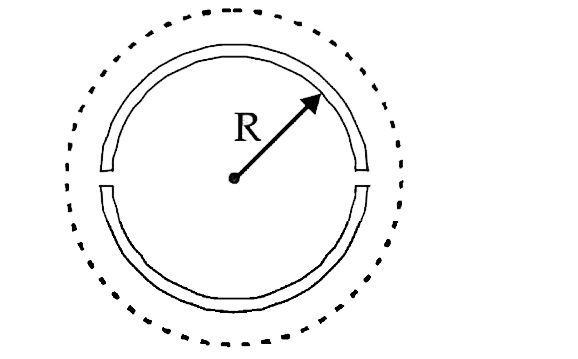
View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
View SolutionA uniform pressure p is exerted on all sides of a solid cube of a material at temprature t∘C. By what amount should the temperature of the cube be raised in order to bring its original volume back to the value it had before the pressure was applied ? K is the bulk modulus and α is the coefficient of linear expansion of material of solid cube.
View SolutionA material has normal density ρ and bulk modulus K. The increase in the density of the material when it is subjected to an external pressure P from all sides is
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View Solution
