If relative permittivity and relative permeability of a medium are 3 and respectively. the critical angle for this medium is.
The correct Answer is:C
To find the critical angle for a medium with given relative permittivity and relative permeability, we can follow these steps:
Step 1: Understand the concept of critical angle
The critical angle is the angle of incidence above which total internal reflection occurs. It can be calculated using Snell's law, where the angle of refraction is 90 degrees.
Step 2: Write down Snell's law for critical angle
According to Snell's law:
n1sin(θc)=n2sin(90∘)
Here, n1 is the refractive index of the medium and n2 is the refractive index of the second medium (usually air, with n2=1).
Step 3: Rearrange the equation for critical angle
Since sin(90∘)=1, we can simplify the equation to:
sin(θc)=n2n1=1n1
Step 4: Calculate the refractive index of the medium
The refractive index n of a medium can be calculated using the formula:
n=√μrϵr
where μr is the relative permeability and ϵr is the relative permittivity.
Given:
- ϵr=3
- μr=43
Substituting these values:
n=√3×43=√4=2
Step 5: Substitute the refractive index into the critical angle equation
Now, substituting n1=2 into the equation for critical angle:
sin(θc)=12
Step 6: Calculate the critical angle
To find θc:
θc=arcsin(12)=30∘
Final Answer
The critical angle for the medium is 30∘.
---
The critical angle is the angle of incidence above which total internal reflection occurs. It can be calculated using Snell's law, where the angle of refraction is 90 degrees.
According to Snell's law:
n1sin(θc)=n2sin(90∘)
Here, n1 is the refractive index of the medium and n2 is the refractive index of the second medium (usually air, with n2=1).
Since sin(90∘)=1, we can simplify the equation to:
sin(θc)=n2n1=1n1
The refractive index n of a medium can be calculated using the formula:
n=√μrϵr
where μr is the relative permeability and ϵr is the relative permittivity.
- ϵr=3
- μr=43
n=√3×43=√4=2
Now, substituting n1=2 into the equation for critical angle:
sin(θc)=12
To find θc:
θc=arcsin(12)=30∘
The critical angle for the medium is 30∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The refractive index and the permeability of a medium are respectively 1.5 and 5×10−7Hm−1. The relative permitivity of the medium is nearly
A25B15C10D6
What is the relative permeability of air ?
View SolutionDo permeability and relative permeability have the same dimensions?
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 4000 Å respectively . The critical angle for the medium is given by
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 3000 Å, respectively. The critical angle is
View SolutionRelative permitivity and permeability of a material εrandμr, respectively . Which of the following values of these quantities are allowed for a diamagnetic material?
View SolutionIf ε0 and μ0 are respectively the electric permittivity and the magnetic permeability of free space and ε and μ the corresponding quantities in a medium, the refractive index of the medium is
View SolutionIn figure, the reflected ray B makes an angle 90∘ with the ray C. If I,r1andr2 are the angles of incidence, reflection and refraction, respectively. Then, the critical angle of the medium is
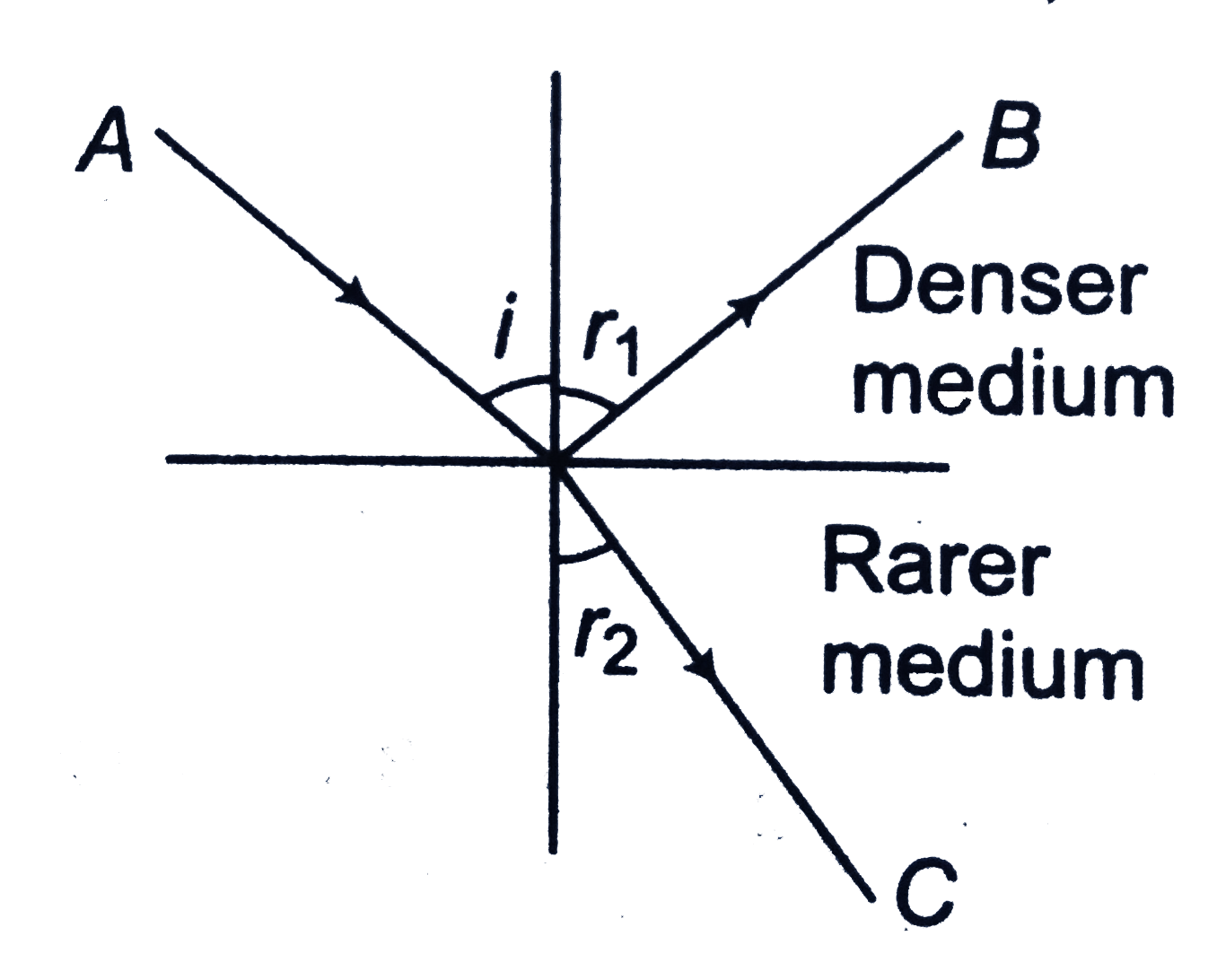
View Solution
