The mass of gas adsorbed , x , per unit mass of adsorbate , m , was measured at various pressures , p . A graph between log xm and log p gives a straight line with slope equal to 2 and the intercept equal to 0.4771 .The value of xm at a pressure of 4 atm is : (Given log 3 = 0.4771)
To solve the problem, we will follow these steps:
Step 1: Understand the relationship given by the graph.
The problem states that a graph between log(xm) and logp gives a straight line with a slope of 2 and an intercept of 0.4771. This can be expressed in the form of the linear equation:
log(xm)=2logp+0.4771
Step 2: Rewrite the equation using properties of logarithms.
Using the property of logarithms that states loga+logb=log(ab), we can rewrite the equation as:
log(xm)=log(p2)+log(3)
This simplifies to:
log(xm)=log(3p2)
Step 3: Remove the logarithm by exponentiating both sides.
To eliminate the logarithm, we exponentiate both sides:
xm=3p2
Step 4: Substitute the given pressure into the equation.
We are asked to find xm at a pressure p=4atm:
xm=3×(4)2
Step 5: Calculate the value.
Calculating 42:
42=16
Now substituting back:
xm=3×16=48
Final Answer:
The value of xm at a pressure of 4 atm is 48.
---
The problem states that a graph between log(xm) and logp gives a straight line with a slope of 2 and an intercept of 0.4771. This can be expressed in the form of the linear equation:
log(xm)=2logp+0.4771
Using the property of logarithms that states loga+logb=log(ab), we can rewrite the equation as:
log(xm)=log(p2)+log(3)
This simplifies to:
log(xm)=log(3p2)
To eliminate the logarithm, we exponentiate both sides:
xm=3p2
We are asked to find xm at a pressure p=4atm:
xm=3×(4)2
Calculating 42:
42=16
Now substituting back:
xm=3×16=48
The value of xm at a pressure of 4 atm is 48.
Topper's Solved these Questions
Similar Questions
Graph between log(xm) and logP is straight line at angle of 45∘ with the intercept of 0.6020.
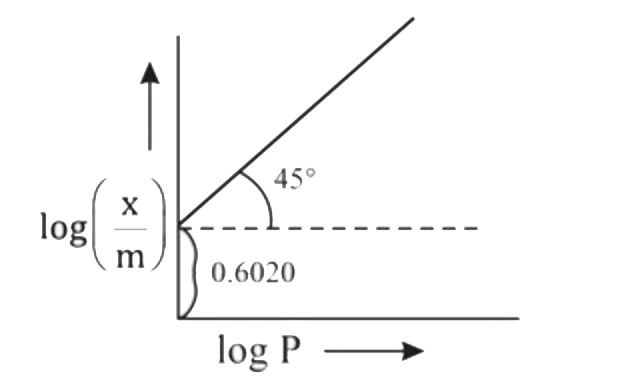
The extent of adsorption (xm) at a pressure of 1 atm is
View SolutionGraph between log(x/m) vs logP has a slope=2 and intercept = 0.477. Find (x/m) at pressure 4 atm.
View SolutionIf a graph is plotted between log(a−x) and t, the slope of the straight line is equal to −0.03. The specific reaction rate will be
View SolutionGraph between log x/m and log P is a straight line at angle of 45∘ with intercept 0.4771 on y-axis. Calculate the amount of gas adsorbed in gram per gram of adsorbent when pressure is 3 atm.
View SolutionFor the adsorption of a gas on a solid, the plot of log(x/m) versus log p is linear with slope equal to
View SolutionGraph between logx/m and logp is a straight line inclined at an angle of 45∘ . When pressure is 0.5atm and 1nk=0.693, the amount of solute adsorbed per gram of adsorbent will be:
View SolutionIn an adsorption experiment, a graph of log(x/m) versus log P was found to be linear with a slope of 45°, and the the intercept is found to be 0.3010. The amount of gas adsorbed per gram charcoal under a pressure of 0.8 atm is
View SolutionWhen a graph is plotted between log x/m and log p, it is straight line with an angle 45∘ and intercept 0.3010 on y− axis . If initial pressure is 0.3 atm, what wil be the amount of gas adsorbed per gm of adsorbent :
View SolutionWhen a graph is plotted between log x/m and log p, it is straight line with an angle 45∘ and intercept 0.6020 on y-axis.if initial pressure is 0.3 atm, what will be the amount of gas adsorbed per gram of adsorbent :
View SolutionA plot of log (xm) against log P for the adsorption of a gas on a sloid gives a straight a straight line with slope equal to :
View Solution
