The force acting on a body of mass 10 kg is (2ˆi+j−ˆk) N. If the body is initially at rest, then velocity at the end of 20 sec will be
2√6
3√2
6√2
2√3
To solve the problem step by step, we will follow these steps:
Step 1: Identify the Given Information
We are given:
- Mass of the body, m=10kg
- Force acting on the body, →F=(2^i+^j−^k)N
- Initial velocity, u=0m/s (since the body is initially at rest)
- Time, t=20s
Step 2: Calculate the Resultant Force
The force vector is given as →F=2^i+1^j−1^k. The magnitude of the force can be calculated using the formula:
|→F|=√(Fx)2+(Fy)2+(Fz)2
where Fx=2, Fy=1, and Fz=−1.
Calculating:
|→F|=√(2)2+(1)2+(−1)2=√4+1+1=√6N
Step 3: Calculate the Acceleration
Using Newton's second law, the acceleration →a can be calculated as:
→a=→Fm
Substituting the values:
→a=√610m/s2
Step 4: Use the First Equation of Motion
The first equation of motion states:
v=u+at
Substituting the known values:
- Initial velocity u=0
- Acceleration a=√610
- Time t=20
Calculating:
v=0+(√610)×20
v=20√610=2√6m/s
Step 5: Final Answer
Thus, the velocity of the body at the end of 20 seconds is:
2√6m/s
---
We are given:
- Mass of the body, m=10kg
- Force acting on the body, →F=(2^i+^j−^k)N
- Initial velocity, u=0m/s (since the body is initially at rest)
- Time, t=20s
The force vector is given as →F=2^i+1^j−1^k. The magnitude of the force can be calculated using the formula:
|→F|=√(Fx)2+(Fy)2+(Fz)2
where Fx=2, Fy=1, and Fz=−1.
|→F|=√(2)2+(1)2+(−1)2=√4+1+1=√6N
Using Newton's second law, the acceleration →a can be calculated as:
→a=→Fm
Substituting the values:
→a=√610m/s2
The first equation of motion states:
v=u+at
Substituting the known values:
- Initial velocity u=0
- Acceleration a=√610
- Time t=20
v=0+(√610)×20
v=20√610=2√6m/s
Thus, the velocity of the body at the end of 20 seconds is:
2√6m/s
Topper's Solved these Questions
Similar Questions
A force of 4N acts on a body of mass 2kg for 4s. Assuming the body to be initially at rest, find (a) its velocity when the force stops acting (b) the distance covered in 10s after the force starts acting.
View SolutionA force acting on a body of mass 2 kg varies with time as shown in fig . 20 find impulse of the force and final velocity of the body.
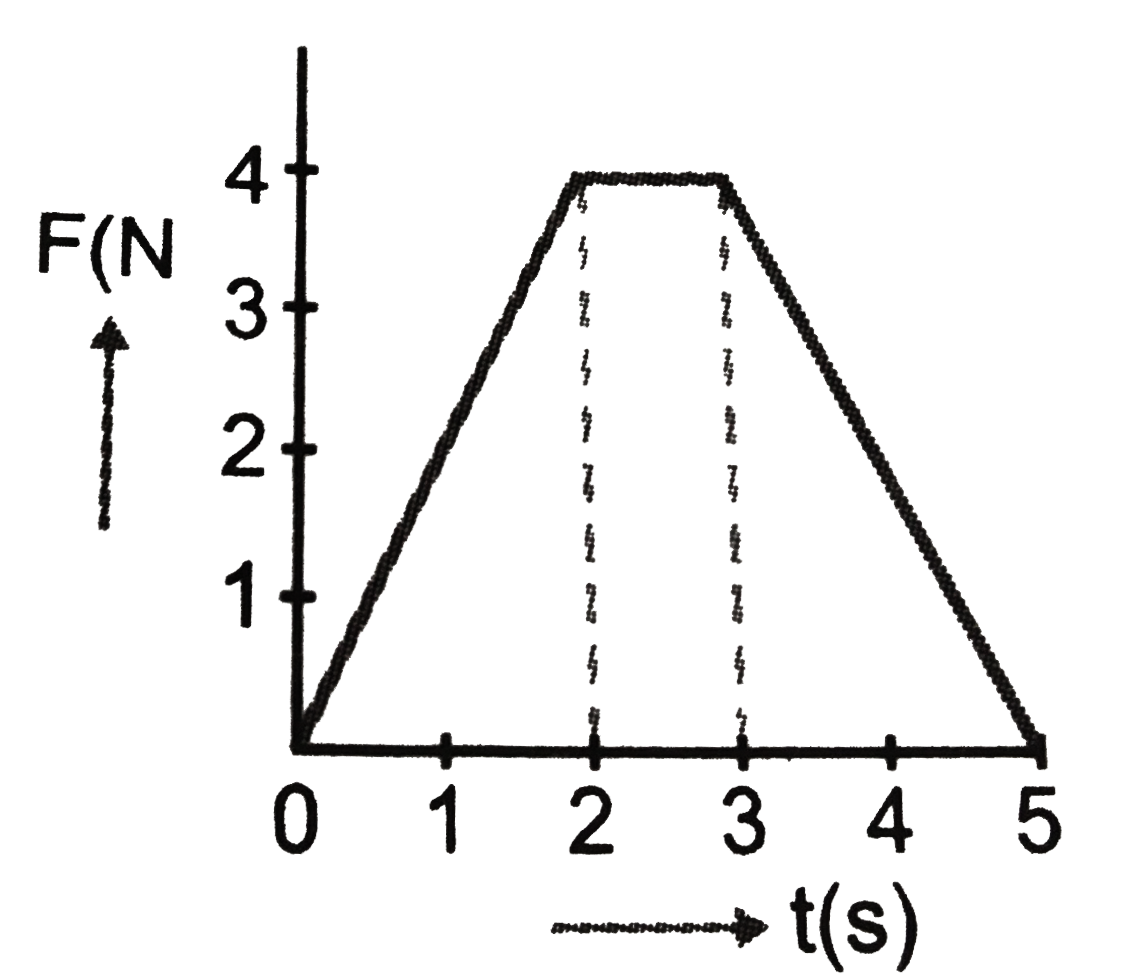
View Solution
Knowledge Check
A force 100 N acts on a body of mass 2 kg for 10 s. The change in velocity of the body is
A100ms−1B250ms−1C500ms−1D1000ms−1The force on a body of mass 1 kg is (20ˆi+10ˆj) N. If is starts from rest, then the position of the body at time t = 2 s, is
A−20ˆi−40ˆjB20ˆi−40ˆjC40ˆi−20ˆjD40ˆi+20ˆjA constant force acts on a body of mass 0.9 kg at rest for 10 s . If the body moves a distance of 250 m, the magnitude of the force is
A3NB3.5NC4.0ND4.5N
Forces of √2N and 6√2N are acting on a body of mass 10 kg at an angle of 60∘ to each other . Find the acceleration , distance covered and velocity of the body after 10 second , if the body is initially at rest .
View SolutionA body of mass 5 kg initially at rest is subject to a force of 20 N. What is the kinetic energy acquired by the body at the end of 10 s ?
View SolutionA body of mass 2 kg is at rest. Two forces of 6 N and 8 N act on the body at right angles to each other. What is the velocity of the body after 3 second?
View SolutionA constant force acts on a body of mass 5 kg at rest for 10s. If the body moves through a distance of 250 m, what is the magnitude of the force?
View SolutionAt a place where the acceleration due to gravity is 10m sec−2 a force of 5 kg - wt acts on a body of mass 10 kg initially at rest. The velocity of the body after 4 second is
View Solution
