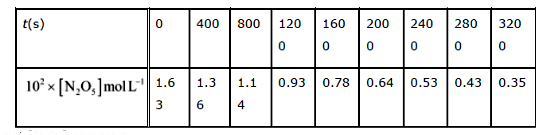
The experimental data for decomposition of N2O5[2N2O5→4NO2+O2] in gas phase at 318 K are given below:
The experimental data for decomposition of N(2)O(5)[2N(2)O(5)to4NO(2)+O(2)] in gas phase at 318 K are given below: (i)Plot [N(2)O(5)]againt t.(ii)Find the half-life period for the reaction (iii)Draw a graph between log[N(2)O(5)] and t. (iv)What is the rate law? (v) Calculate the rate constant. (vi)Calculate the half-life periof from k and compare it with (ii)
(i)Plot [N2O5]againt t.(ii)Find the half-life period for the reaction
(iii)Draw a graph between log[N2O5] and t. (iv)What is the rate law?
(v) Calculate the rate constant.
(vi)Calculate the half-life periof from k and compare it with (ii)

Text Solution
Verified by Experts

ocr_image
ocr_image
(ii)Calculation of half life of reaction (t12):
Time=0 to 3200 second
∴t12=1600s
t=0 time [N2O5]
=1.63×10−2molL−1
So, Half concentration 1.632=0.815×10−2molL−1 ∴t12=1402s
(iii) The graph of log [N2O5] and time t:
ocr_image
(Note:The graph is linear and negative slope=−k2.303)
ocr_image
(iv)Derive rate law:The graph of log [N2O5] and t is linear means 1st order reaction.
Reaction :2N2O5→4NO2+O2
(v)Calculation of rate constant (K):
Graph method:From log [N2O5]→t graph
The slope of line =−k2.303
∴k=−slope×2.303
=−2.46−(−1.79)(3200−0)s×2.303
=−−0.673200×2.303
=4.8219×10−4s−1=4.82×10−4s−1
OR
Calculation of k on the base of equation :
k=2.303Δt log [R]0[R]t
Where ,Δt=3200-0=3200s
[R]0=Concentration at zero time 1.63×10−2molL−1
[R]t=Concnentration at 3200 second 0.35×10−2molL−1
∴[R]0[R]t=1.63×10−20.35×10−2 ∴k=2.3033200×0.6681 4.8083×10−4=4.81×10−4s−1
(vi)Calculation of t12:
t12=0.693k=0.6934.8219×10−4s−1 0.1437×104s=1.437×103S=1437s
Half life time :1437 s < 1402 s

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12CHEMISTRYCHEMICAL KINETICS

Topper's Solved these Questions

Similar Questions