Two unequal masses are connected by a very light string over a clamped light smooth pulley. Find the acceleration of the system and the tension in the string. Solution in Telugu
Step by step video & image solution for Two unequal masses are connected by a very light string over a clamped light smooth pulley. Find the acceleration of the system and the tension in the string. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - SHORT ANSWER TYPE QUESTION2videos
LAWS OF MOTION
- BOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS14videos
LAWS OF MOTION
- BOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE108videos
LAWS OF MOTION
- BOOK - AAKASH SERIESCHAPTER - KINETIC THEORY OF GASESEXERCISE - Practice Exercise15videos
KINETIC THEORY OF GASES
- BOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-343videos
MECHANICAL PROPERTIES OF FLUIDS
Similar Questions
Two blocks of masses M1andM2 are placed in contact with each other on a frictionless horizontal surface. If a force F is applied on the block of mass M, what is the contact force between the two blocks?
04:31View SolutionWhen a horse pulls a cart, which force helps the horse to move forward ?
04:08View SolutionA force of 50 N acts on a body of mass 10 kg at rest. Its velocity after 5 seconds if the same force acts, is
05:05View SolutionThe kinetic energy of translation of 20gm of oxygen at 47∘C is (molecular wt. of oxygen is 32gm/mol and R=8.3 J/mol/K)
05:30View SolutionA U-tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are in level with 10.0cm of water in one arm and 12.5cm of spirit in the other. What is the specific gravity of spirit.
08:29View SolutionA light string passing over a smooth light pulley connects two blocks of masses m_1 and m_2 (vertically). If the acceleration of the system is g//8 , then the ratio of the masses is
View SolutionTwo bodies of masses 4kg and 3kg respectively are connected by a light string passing over a smooth frictionless pulley Calculate the acceleration of the masses and tension in the string .
02:48View SolutionThe pulley is light and smooth : the strings are inextensible and light. The system is released from rest, find the acceleration of each block, tensions in the strings and reaction in pulley.
04:56View SolutionTwo blocks are connected by an inextensible light string, the string is passing over smooth, light pulley as shown. Find the acceleration of blocks and tension in the string, reaction in pulley.
03:07View SolutionThe strings are inextensible and light: the pulleys are smooth and light. Find the acceleration of each block and tensions in the strings.
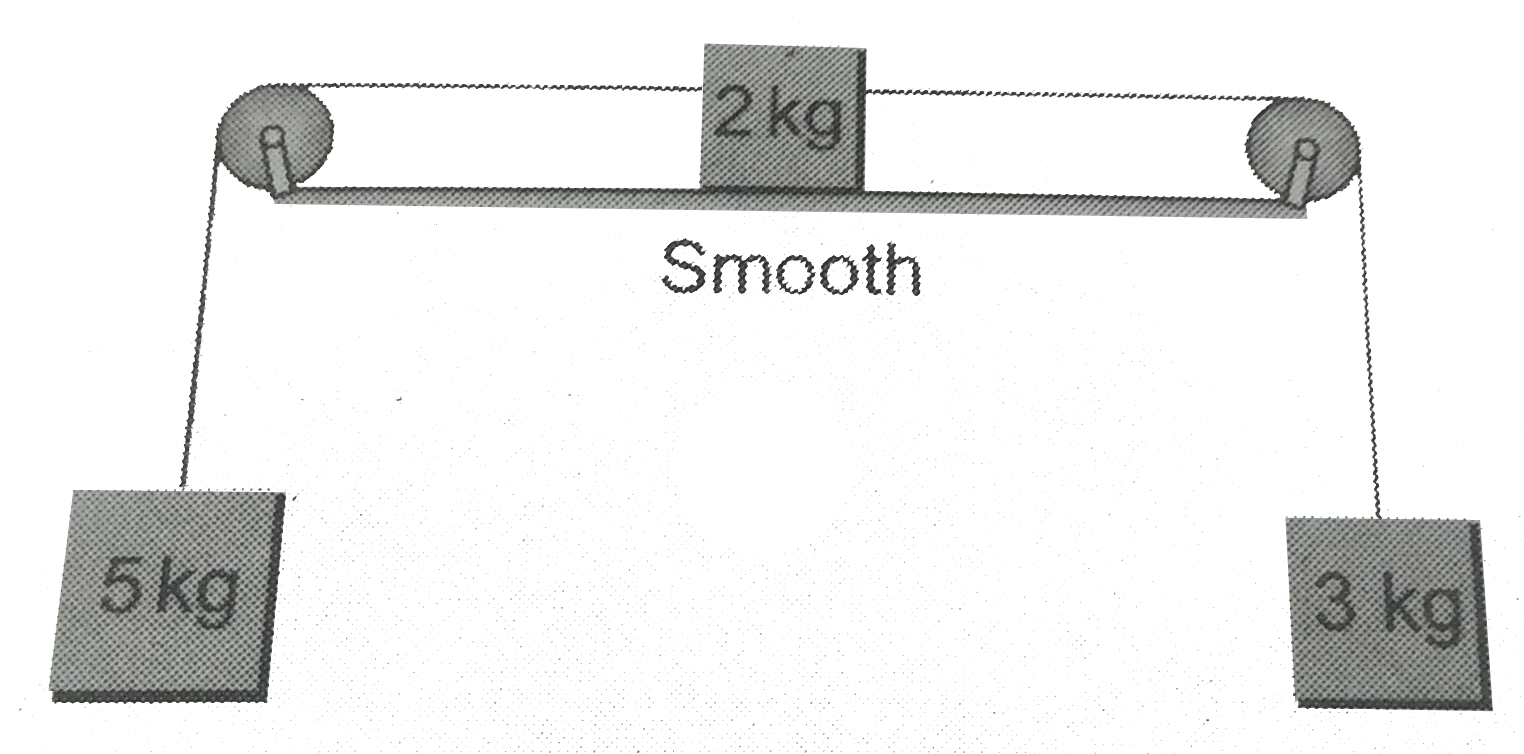 03:57View Solution
03:57View SolutionString going over a pulley connects two blocks of masses m, and my. String and pulley are light. Calculate acceleration of centre of mass of blocks system.
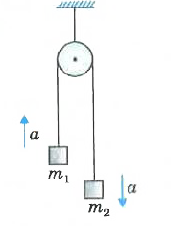 View Solution
View SolutionA light string passing over a smooth light pulley connects two blocks of masses m_1 and m_2 (vertically). If the acceleration of the system is g/8 then the ratio of the masses is
03:57View SolutionTwo bodies of masses 2kg and 3kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string (g=10m s^(-2) )
View SolutionTwo unequal masses are connected by a very light string over a clamped light smooth pulley. Find the acceleration of the system and the tension in the string.
04:23View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
07:50View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
03:33View Solution

