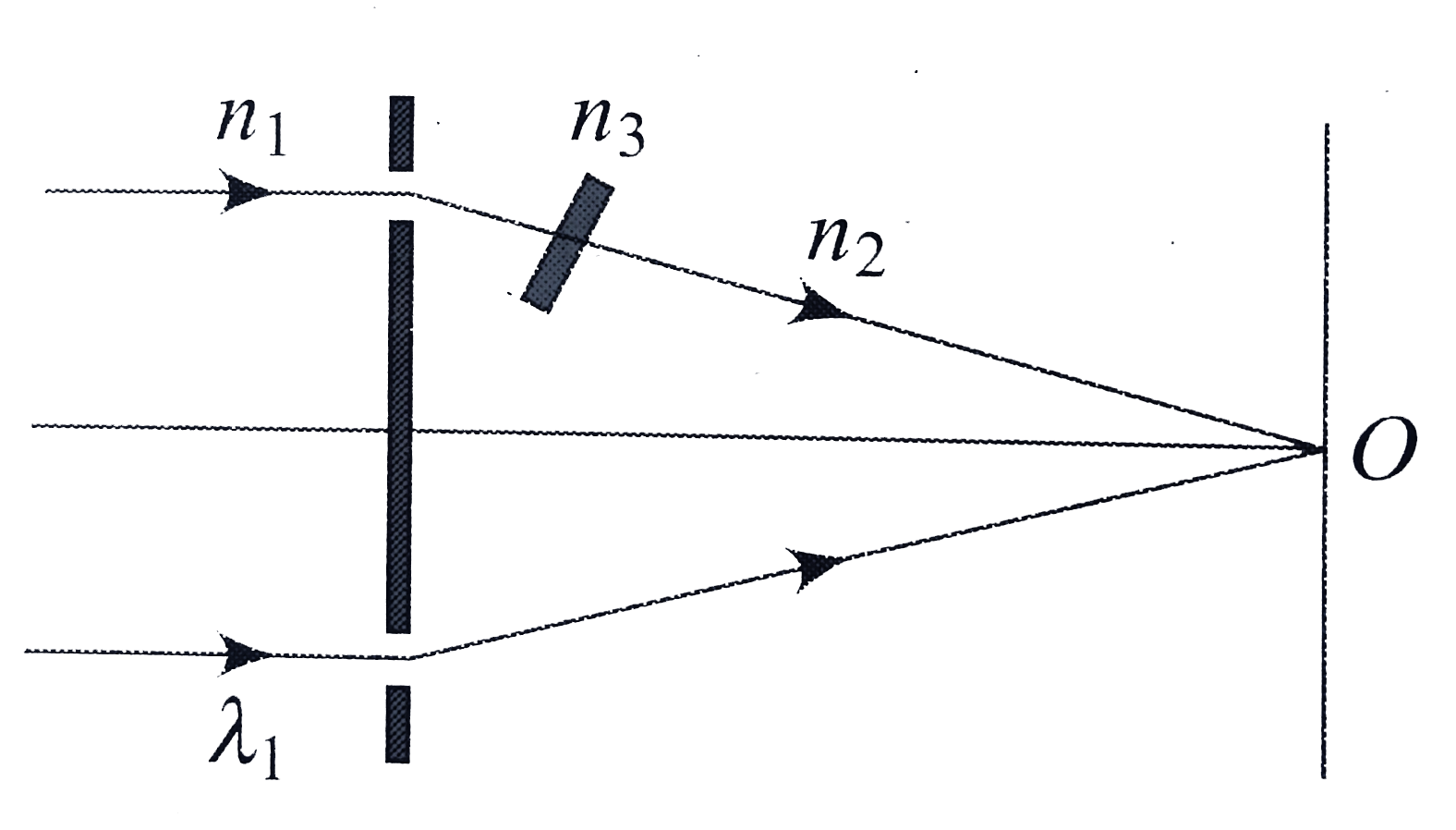
In the figure shown in YDSE, a parallel beam of light is incident on the slit from a medium of refractive index $n_{1}$ . The wavelength of light in this medium is $λ_{1}$ . A transparent glass of thickness t and of refractive index $n_{3}$ is put in front of one of the slits. The medium between the screen and the plane of the slits is $n_{2}$ . The phase difference between the light waves reaching point .O. (symmetrically relative to the slit is

Text Solution

Verified by Experts

$ϕ = \frac{2 π}{λ_{2}} (\frac{n_{3}}{n_{2}} - 1) t = \frac{2 π}{n_{1} λ_{1}} n_{2} (\frac{n_{3} - n_{2}}{n_{2}}) t$
$(∴ n_{1} λ_{1} = n_{2} λ_{2}) ϕ = \frac{2 π}{n_{1} λ_{1}} (n_{3} - n_{2}) t$ .

|

Updated on:21/07/2023

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In YDSE shown in figure a parallel beam of light is incident on the slits from a medium of refractive index $n_{1}$ . The wavelength of light in this medium is $λ_{1}$ . A transparent of thickness t and refractive index $n_{3}$ is put in front of one slit. The medium between the screen and the plane of the slits is $n_{2}$ . The phase difference between the light waves reaching point O (symmetrical, relative to the slits) is

A $\frac{2 π}{n_{1} λ_{1}} (n_{3} - n_{2}) t$
B $\frac{2 π}{λ_{1}} (n_{3} - n_{2}) t$
C $\frac{2 π n_{1}}{n_{2} λ_{1}} (\frac{n_{3}}{n_{2}} - 1) t$
D $\frac{2 π n_{1}}{λ_{1}} (n_{3} - n_{2}) t$
Question 2 - Select One
AN unpolarised beam of light is incident at the polarising angle on the surface of a transparent medium of refractive index 1.6. The angle of refraction in the medium is
A $58^{\circ}$
B $29^{\circ}$
C $32^{\circ}$
D $42^{\circ}$
Question 3 - Select One
In a medium of refractive index $n_{1}$ , a monochromatic light of wavelength $λ_{1}$ is travelling. When it enters in a denser medium of refractive index $n_{2}$ , the wavelength of the light in the second medium is
A $λ_{1} (\frac{n_{1}}{n_{2}})$
B $λ_{1} (\frac{n_{2}}{n_{1}})$
C $\frac{λ_{1} (n_{2} - n_{1})}{n_{2}}$
D $\frac{λ_{1} (n_{2} - n_{1})}{n_{1}}$

AAKASH SERIES-WAVES OPTICS-EXERCISE -III (POLARISITION)

Home
Ask Doubt
Profile