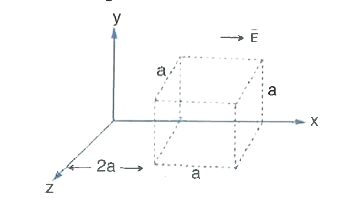
In a region of space, the electric field is the x direction and is given as →E=E0xˆi. Consider an imaginary cubical volume of edge a, with its edges parallel to axes of coordinates. The charge inside this volume is : Solution in Telugu
zero
∈0E0a3
1∈0E0a3
16∈0E0a2
The correct Answer is:B
Step by step video, text & image solution for In a region of space, the electric field is the x direction and is given as vec(E ) = E_(0)x hat(i). Consider an imaginary cubical volume of edge a, with its edges parallel to axes of coordinates. The charge inside this volume is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -III ELECTRO FLUX & GAUSS.S LAW) (LEVEL - I (ADVANCED) MORE THAN ONE CORRECT ANWER TYPE QUESTIONS5videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -III ELECTRO FLUX & GAUSS.S LAW) (LEVEL - I (ADVANCED) LINKED COMPREHENSION TYPE QUESTIONS)3videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE SHEET (EXERCISE -III ELECTRO FLUX & GAUSS.S LAW) (LEVEL - I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)9videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - ADDITIONAL EXERCISE6videos
ELECTROMAGNETICS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS9videos
ELEMENTS OF VECTORS
Similar Questions
The electric field in a region of space varies as E=(3v/m)xˆi+(4v/m)yˆj+(5v/m)zˆk Consider a differential cube whose one vertex is (x, y, z) and the three sides are dx, dy, dz, sides being parallel to the three coordinate axes.
03:46View SolutionFour initially uncharaged thin, large, plane idential metallic plates A,B,C and D are arranged parallel to each other as shown. Now plates A,B,C and D are given charges Q,2Q,3Q and 4Q respectively. Plates A and D are connected by a mtallic wire while plates B and C connected by other metallic wire then after Electrostatic equilibrium is reached.

Charge on left surface of plate B wll be04:59View SolutionThe electric field in a region of space is given by E=5i+2jNC. The electric flux due to this field through an area 2m2 lying in the YZ plane. In S.I. units is
05:57View SolutionWhat is the radius of the path of an electron (mass 9 × 10-31 kg and charge 1.6×x10–19 C) moving at a speed of 3×x107 m/s in a magnetic field of 6x×10−4 T perpendicular to it? What is its frequency? Calculate its energy in keV. (1eV=1.6×10−19J)
02:28View SolutionFind the components of vector ¯¯¯A=2ˆi+3ˆj along the directions of ˆi+ˆjandˆi−ˆj
05:57View SolutionIn a region of space the electric field in the x-direction and proportional to xi.e., →E=E0xˆi. Consider an imaginary cubical volume of edge a with its sides parallel to the axes of coordinates. The charge inside this volume will be
09:36View SolutionThe electric field in a region is given by →E=E0axˆi. Find the electric flux passing through a cubical volume bounded by the surfaces x=0,x=a,y=0,y=a,z=0 and z=a.
View SolutionThe electric fields in a region is given by →E=E0xLˆi ,Find the charge contained inside a cubical volume bounded by the surface x=0,x=L,y=0,y=L,z=0,z=L.
View SolutionThe magnetic field in a region is given by vec(B)=B_(0)(1+(x)/(a))hat(k) . A square loop of edge length 'd' is placed with its edge along X-axis and Y-axis. The loop is moved with a constant velocity vec(V)=V_(0)hat(i) . The emf induced in the loop is
View SolutionThe electric field in a region is given by →E=E0xˆi. The charge contained inside a cubical volume bounded by the surface x=0, x=2m, y=0, y=2m, z=0 and z=2m is nε0E0. Find the va,ue of n.
View Solutionकिसी स्थान पर विधुत क्षेत्र X -अक्ष की दिशा में है एव x के समानुपाती है, अर्थात →E=E0xˆi। मान लीजिए a भुजा वाला एक काल्पनिक घन इस प्रकार है की इसकी कोरे निर्देशाक्षो के समान्तर है । इस घन के अंदर आवेश ज्ञात कीजिए।
02:23View SolutionThe electric field in a region is given by vec(E )= E_(0) (x)/(L) hat(i) . Find the charge contained inside a cubical volume bounded by the surface x=0, x= L, y= 0, y= L, z= 0 and z=L.
05:49View SolutionThe electric field in a region of space is given by, →E=E0ˆi+2E0ˆj where E0=100N/C. The flux of this field through a circular surface of radius 0.02m parallel to the Y-Z plane is nearly.
04:37View SolutionThe electric field in a regions is given by vec(E) = E_(0) (x)/(L) hat(i) . Find the charge contained inside a cubical volume bounded by the surface x =0,x=L,y = 0 ,z = 0 and z=L.
02:58View SolutionIn a region, electric field depends on X-axis as E≈E0x2. There is a club of edge a as shown. Then find the charge enclosed in the cube.
 02:39View Solution
02:39View Solution

