Obtain an expression for the position vector of centre of mass of a system n particles in two dimension. Solution in Gujrati
Suppose that there are three particles not lying in a straight line so they are taken in a plane.
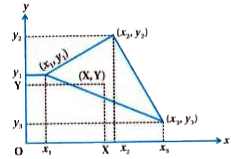
Suppose the coordinates of three particles of masses m1,m2andm3 are (x1,y1),(x2,y2)and(x3,y3) in two dimension respectively.
If the centre of mass of this system is (x,y) then X-coordinate of the centre of mass,
X-coordinate = m1x1+m2x2+m3x3m1+m2+m3 and
Y-coordinate = m1y1+m2y2+m3y3m1+m2+m3
∴ Centre of mass,
(X,Y)=(m1x1+m2x2+m3x3m1+m2+m3,m1y1+m2y2+m3y3m1+m2+m3)
If all three particles having same mass,
m1=m2=m3=m assume,
∴x-coordinate of centre of mass,
X=mx1+mx2+mx3m+m+m
=x1+x2+x33 and
y-coordinate Y=my1+my2+my3m+m+m
=y1+y2+y33
Hence centre of mass of system form by three particles of same mass will be at middle of triangle form by these three particles.
The centre of mass of three particles which are not lying on a line is written as,
Coordinate of C.M.
(X,Y)=m1(x1,y1)+m2(x2,y2)+m3(x3,y3)m1+m2+m3
Coordinate of centre of mass of n particle which are not in line.
(X,Y)=m1(x1,y1)+m2(x2,y2)+...mn(xn,yn)m1+m2+...mn
OR
(X,Y)=Σmi(xi.yi)M
where M=Σmi total mass of system and x-coordinate and y-coordinate of centre of mass of system,
X=m1x1+m2x2+...mnxnm1+m2...mn=ΣmixiM
and Y=m1y1+m2y2+...mnxnm1+m2...mn=ΣmiyiM
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-A HOTS3videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-A (TRY YOURSELF (VSQs))94videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTIONS ASKED IN JEE - 2020 EXERCISE - Question16videos
QUESTIONS ASKED IN JEE - 2020
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Paper (Section - D) (Answer following in brief :) Each carry 4 marks1videos
THERMAL PROPERTIES OF MATTER
Similar Questions
What is torque? Explain the torque acting on a particle.
01:54View SolutionWhat is a rigid body ? Explain the differences between rigid body and solid body.
08:28View SolutionA non-isotropic solid metal cube has coefficient of linear expansion as 5×10−5/∘C along the x-axis and 5×10−6/∘C along y-axis and z-axis. If the coefficient of volumetric expansion of the solid is n×10−6/∘C then the value of n is....
04:19View SolutionIn an experiment on the specific heat of a metal, a 0.20 kg block of the metal at 150∘C is dropped in a copper calorimeter (of water equivalent 0.025 kg) containing 150 cm3 of water at 27∘C. The final temperature is 40∘C. Compute the specific heat of the metal. If heat losses to the surroundings are not negligible, is your answer greater or smaller than the actual value for specific heat of the metal ?
02:02View SolutionWhat is the position vector of centre of mass of two particles of equal masses ?
04:45View SolutionObtain an expression for the position vector of centre of mass of a two particle system.
11:39View SolutionObtain an expression for the position vector of centre of mass of a system of n particles.
View SolutionStatement-1 : The centre of mass of a system of n particles is the weighted average of the position vector of the n particles making up the system . Statement-2 : The position of the centre of mass of a system is independent of coordinate system
06:16View SolutionDerive an expression for the position vector of the center of mass of particle system.
01:26View SolutionWhat is centre of mass of a body ? Obtain an expression for the positive vector of a centre of mass of two particle system.
View SolutionPosition vector of the centre of mass of a system of N particles of total mass M is
01:50View SolutionWrite an expression for the position vector of the centre of mass of n-particle system. Also write the equations of motion which govern the motion of the centre of mass
02:37View SolutionThe position vector of the centre of mass of a system of particles is the _________ of the position vectors of the particles, each particle making a contribution proportional to its _________
04:07View Solution(A) : The centre of mass of system of n particles is the weight average of the position vector of the n particles making up the system. (R ) : The position of the centre of mass of a system is independent of co-ordinate system.
01:54View SolutionObtain an expression for the position vector of centre of mass of a system of n particles in one dimension.
02:02View Solution

