Some of notable matters in derivation of M→A=→Fext. Solution in Gujrati
(1) To determine the motion of the centre of mass no knowledge of internal forces of the system of particles is required for this purpose to know only the external forces.
(2) To obtain this equation we did not need to specify the nature of the system of particles. The system may be a collection of particles in which there may be all kinds of internal motion, or it may be a rigid body which has either pure translational motion or a combination of translational and rotational motion.
(3) Whatever is the system and the motion of its individual particles, the centre of mass moves according to M→A=→Fext.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-A HOTS3videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-A (TRY YOURSELF (VSQs))94videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTIONS ASKED IN JEE - 2020 EXERCISE - Question16videos
QUESTIONS ASKED IN JEE - 2020
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Paper (Section - D) (Answer following in brief :) Each carry 4 marks1videos
THERMAL PROPERTIES OF MATTER
Similar Questions
What is torque? Explain the torque acting on a particle.
06:27View SolutionWhat is a rigid body ? Explain the differences between rigid body and solid body.
06:32View SolutionA non-isotropic solid metal cube has coefficient of linear expansion as 5×10−5/∘C along the x-axis and 5×10−6/∘C along y-axis and z-axis. If the coefficient of volumetric expansion of the solid is n×10−6/∘C then the value of n is....
02:50View SolutionIn an experiment on the specific heat of a metal, a 0.20 kg block of the metal at 150∘C is dropped in a copper calorimeter (of water equivalent 0.025 kg) containing 150 cm3 of water at 27∘C. The final temperature is 40∘C. Compute the specific heat of the metal. If heat losses to the surroundings are not negligible, is your answer greater or smaller than the actual value for specific heat of the metal ?
03:51View SolutionThe gravitational force vec(F) upon an object of mass m placed in external gravitational field vec(E )_("ext") is given by vec(F)=m vec(E )_("ext") . Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction. The intensity of gravitational field has the maximum value at x equal to
02:51View SolutionThe gravitational force →F upon an object of mass m placed in external gravitational field →Eext is given by →F=m→Eext. Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction.
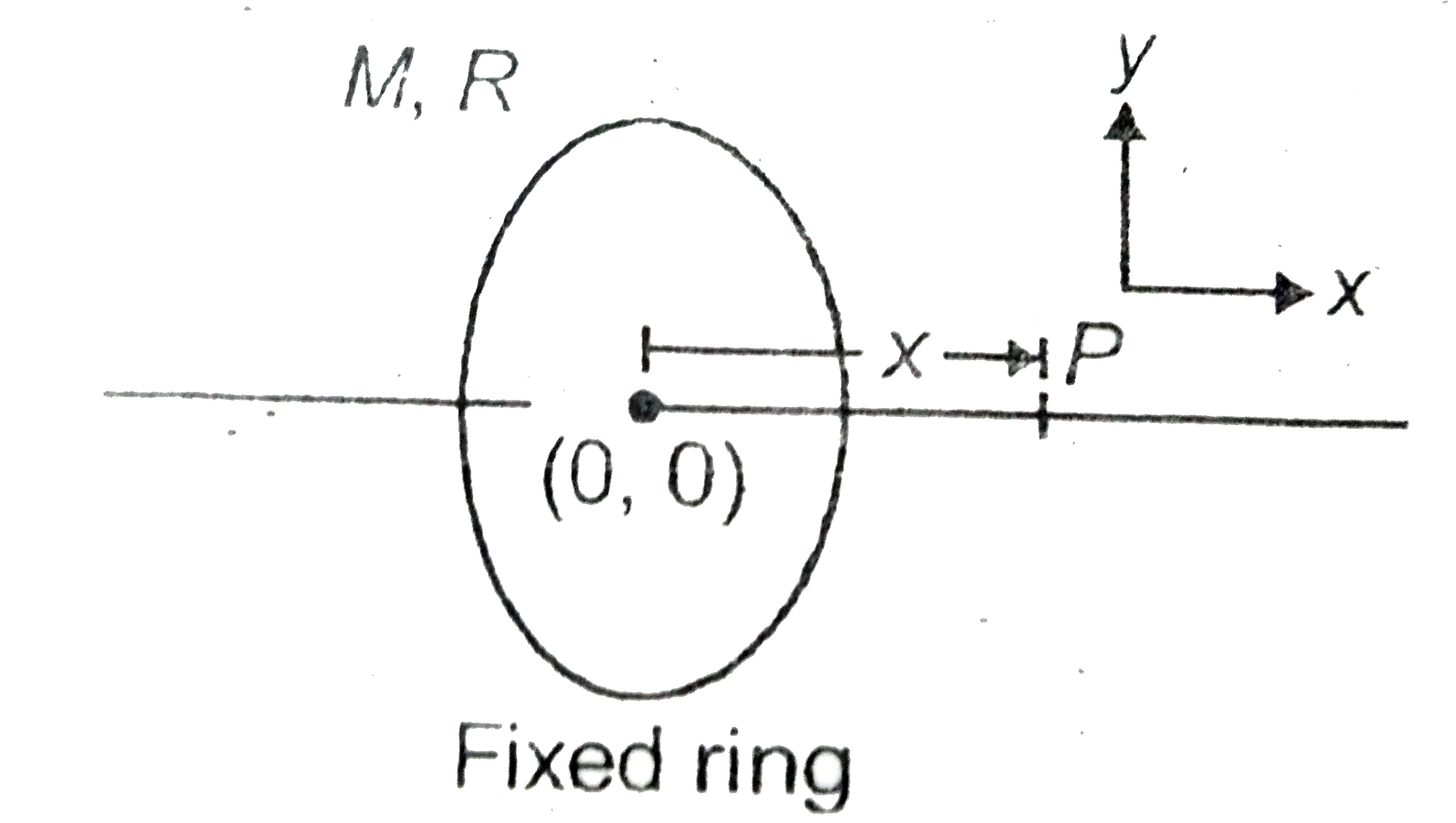
The value of maximum gravitational field intensity is02:12View SolutionThe gravitational force →F upon an object of mass m placed in external gravitational field →Eext is given by →F=m→Eext. Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction.

The gravitational field is zero at x equal to06:14View SolutionThe gravitational force →F upon an object of mass m placed in external gravitational field →Eext is given by →F=m→Eext. Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction.
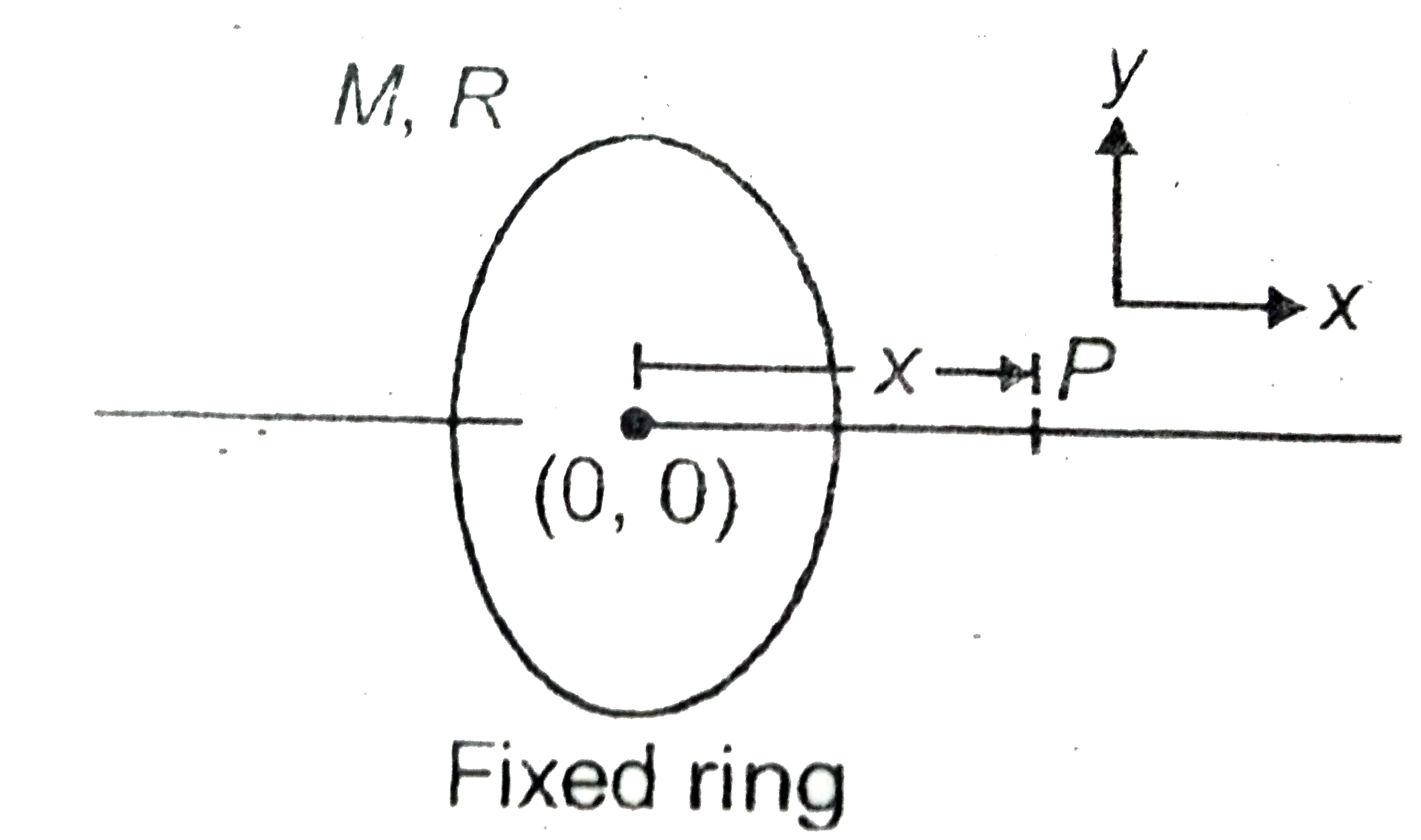
For a point near to the centre i.e., x lt lt R, the intensity of gravitational field E03:04View SolutionThe gravitational force vec(F) upon an object of mass m placed in external gravitational field vec(E )_("ext") is given by vec(F)=m vec(E )_("ext") . Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction. If the particle (mass m) is released from rest from a point distant x = R sqrt(3) , then it will pass through the centre of ring with speed
05:13View SolutionThe gravitational force vec(F) upon an object of mass m placed in external gravitational field vec(E )_("ext") is given by vec(F)=m vec(E )_("ext") . Consider a fixed mass ring (of mass M, radius R) in y-z plane, with centre at (0,0) and axis of ring along x direction. If the point mass m placed at the centre of ring and given a velocity v_(0) such that point mass escapes from the gravitational field of ring. Then v_(0) is
01:31View SolutionIf the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Work done on the gas in single stage compression is :06:44View Solutionसूत्र →F=m→a का निगमन कीजिए।
05:45View SolutionExplain the physical significance of the equation M→A=→Fext, where 'M' is the mass of the whole rotating body and →A is the acceleration of the centre of mass of the system of particles.
03:10View SolutionEquation MvecA=vecF_(ext) denote what?
02:17View SolutionDerive the equation W=−PextΔV
02:43View Solution

