Question

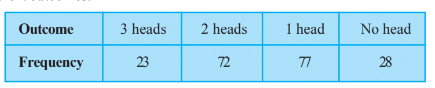
Three coins are tossed simultaneously 200 times with the following frequencies of different outcomes :
$\begin{array}{ccccc}\text{Outcome}& 3& 2& 1& No\\ & \text{heads}& \text{heads}& \text{heads}& \text{heads}\\ \text{Frequency}& 23& 72& 77& 28\end{array}$
If the three coins are simultaneously tossed again , compute the probability of 2 heads coming up.

Explanation

To solve the problem of finding the probability of getting 2 heads when three coins are tossed, we can follow these steps:

Step 1: Understand the problem
We are given the frequencies of different outcomes when three coins are tossed 200 times. The outcomes and their frequencies are:
- 3 heads: 23 times
- 2 heads: 72 times
- 1 head: 77 times
- No heads: 28 times

Step 2: Identify the total number of trials
The total number of times the coins were tossed is 200.

Step 3: Identify the favorable outcomes
We need to find the probability of getting exactly 2 heads. From the given data, the frequency of getting 2 heads is 72.

Step 4: Calculate the probability
The probability (P) of an event is given by the formula:
\( P(\text{event}) = \frac{\text{Number of favorable outcomes}}{\text{Total number of outcomes}} \)
In this case, the event is getting 2 heads. Thus, we can substitute the values:
\( P(\text{2 heads}) = \frac{72}{200} \)

Step 5: Simplify the fraction
Now we simplify the fraction:
\( P(\text{2 heads}) = \frac{72 \div 8}{200 \div 8} = \frac{9}{25} \)

Step 6: Final answer
The probability of getting exactly 2 heads when three coins are tossed is:
\( \frac{9}{25} \)

---