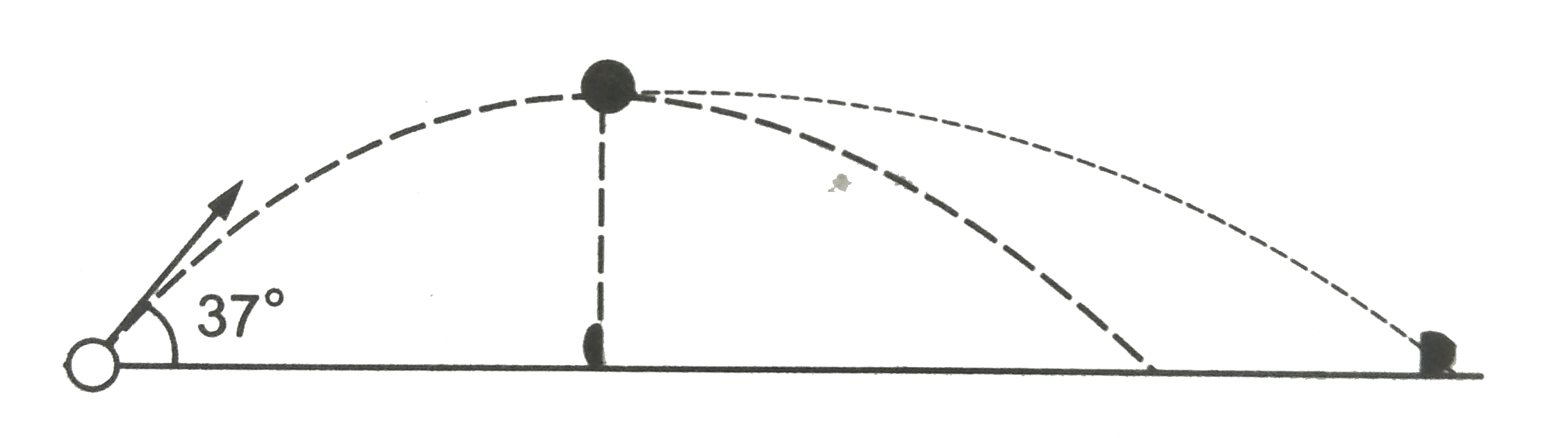
A projectile is fired at a speed of 100 m/s at an angle of 37° above the horizontal. At the highest point, the projectile breaks into two parts of mass ratio 1 :3, the smaller coming to rest. Find the distance from the launching point to the point where the heavier piece lands.

Text Solution

Verified by Experts

At the highest point, the projectile has horizontal velocity. The lighter part comes to rest. Hence, the heavier part will move with increased horizontal velocity. In vertical direction, both parts have zero velocity and undergo same acceleration. Thus, both will hit the ground together. As internal forces do not affect the motion of the centre of mass, the centre of mass hits the ground at the position where the original projectile would have landed. The range of the original projectile is,
$x_{C M} = \frac{2 u^{2} sin θ cos θ}{g} = \frac{2 \times 10^{4} \times \frac{3}{5} \times \frac{4}{5}}{10} m = 960 m$
The centre of mass will hit the ground at this position. As the smaller block comes to rest after breaking, it falls down vertically and hits the ground at half of the range, i.e., at x = 480m. If the heavier block hits the ground at $x_{2}$ , then
$x_{C M} = \frac{m_{1} x_{1} + m_{2} x_{2}}{m_{1} + m_{2}}$ (or) $960 = \frac{(m) (480) + (3 m) (x_{2})}{m + 3 m}$ or $x_{2} = 1120$ m.

Updated on:21/07/2023

A bullet fired from gun with a velocity 30 m/s at an angle of $60^{\circ}$ with horizontal direction. At the highest point of its path, the bullet explodes into two parts with masses in the ratio 1 : 3. The lighter mass comes to rest immediately. Then the speed of the heavier mass is
A30 m/s
B20 m/s
C10 m/s
D5 m/s
A projectile of mass 20 kg is fired with a velocity of $400 m / s$ at an angle of $45^{\circ}$ with the horizontal .At the highest point of the trajectocy the projectile explodes into two fragments of equal mass,one of which falls vertically downward with zero initial speed, The distance of the point where the other fragment falls from the point offiring is:
A24000 m
B16000 m
C32000 m
D8000 m
A projectile is fired with a speed u at an angle $θ$ above the horizontal field. The coefficient of restitution between the projectile and field is e. Find the position from the starting point when the projectile will land at its second collision
A $\frac{e^{2} u^{2} sin 2 θ}{g}$
B $\frac{(1 - e^{2}) u^{2} sin 2 θ}{g}$
C $\frac{(1 - e) u^{2} sin θ cos θ}{g}$
D $\frac{(1 + e) u^{2} sin 2 θ}{g}$