If a particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P (AP < PB) at successive intervals of 0.5 sec and 1.5 sec with a speed of 3 m/s. The maximum speed of the particle is
3 m/s
3√2
3√3 m/s
6 m/s
The correct Answer is:B
Topper's Solved these Questions
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - OSCILLATIONS (SIMPLE PENDULUM)
16 Videos
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - OSCILLATIONS (SPRINGS)
18 Videos
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - SYSTEM OF PARTICLES AND ROTATIONAL MOTION (EQUILIBRIUM OF BODY & PARALLEL FORCES)
6 Videos
- CALORIMETRY BOOK - AAKASH SERIESCHAPTER - CALORIMETRY EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) (LECTURE SHEET (ADVANCED)(Matrix Type Questions)
1 Video
Similar Questions
(i) The acceleration (a) of a particle moving along a straight line is related to time (t) as per the differential equation d2adt2=−ba. b is a positive constant. Is the particle performing SHM? If yes, what is the time period?
(ii) A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P (AP>PB) with a speed of 3m/s at times recorded as t = 0, 0.5 s, 2.0 s, 2.5 s, 4.0 s, 4.5 s......Determine the maximum speed of the particle and also the ratio AP/PB.
View SolutionA particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.
View Solution
Knowledge Check
A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(AP<BP) at successive intervals of 0.5 sec and 1.5 sec with a speed of 3 m/s. Then
AThe maximum speed of particle is 3√2 m/sBthe maximum speed of particle is √2 m/sCthe ratio APBP is sqr2−1√2+1Dthe ratio APBP is 1√2A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.
Athe maximum speed of particle is 3√2m/sBthe maximum speed of particle is √2ms.Cthe ratio (AP/BP) is √2−1√2+1Dthe ratio AP/BP is 1√2A and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. The ratio AXBX isA2√2−12√2+1B√3−1√3+1C3√2−23√2+2D13
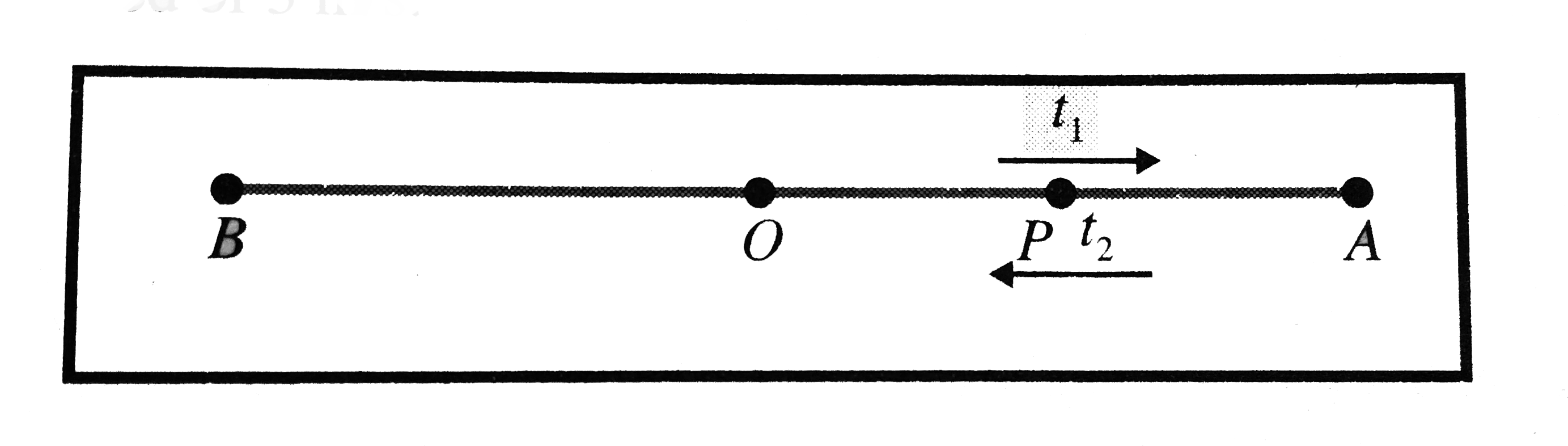
A particle starts SHM from mean position O executing SHM A and B are the two point at which its velocity is zero . It passes through a certain point P at time t1=0.5andt2=1.5s with a speed of 3m/s.
i. The maximum speed .......
ii. ratio AP/PB ....
 .
.
View SolutionA and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. The ratio AXBX is
View SolutionA and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. Amplitude of oscillation is
View SolutionA particle is executing SHM along a straight line. Then choose the correct statement
View SolutionA and B are two points on the path of a particle executing SHM in a straight line, at which its velocity is zero. Starting from a certain point X (AX<BX) the particle crosses this pint again at successive intervals of 2 seconds and 4 seconds with a speed of 5m/s
Q. Amplitude of oscillation is
View Solution
