An ideal monoatomic gas undergoes a process where its pressure is inversely proportional to its temperature. a) Calculate the molar specific heat for the process b) Find the work done by two moles of gas if the temperature changes from $T_{1}$ to $T_{2}$

Text Solution

Verified by Experts

The correct Answer is: $\frac{7 R}{2}, 4 R (T_{2} - T_{1})$

Updated on:21/07/2023

In a process the pressure of a gas is inversely proportional to the square of the volume. If temperature of the gas increases, then work done by the gas:
Ais positive
Bis negative
Cis zero
Dmay be positive
In a process, the pressure of an ideal gas is proportional to square of the volume of the gas. If the temperature of the gas increases in this process, then work done by this gas
A(a) is positive
B(b) is negative
C(c) is zero
D(d) may be positive or negative
One mole of an ideal monoatomic gas undergoes a process as shown in the figure. Find the molar specific heat of the gas in the process.

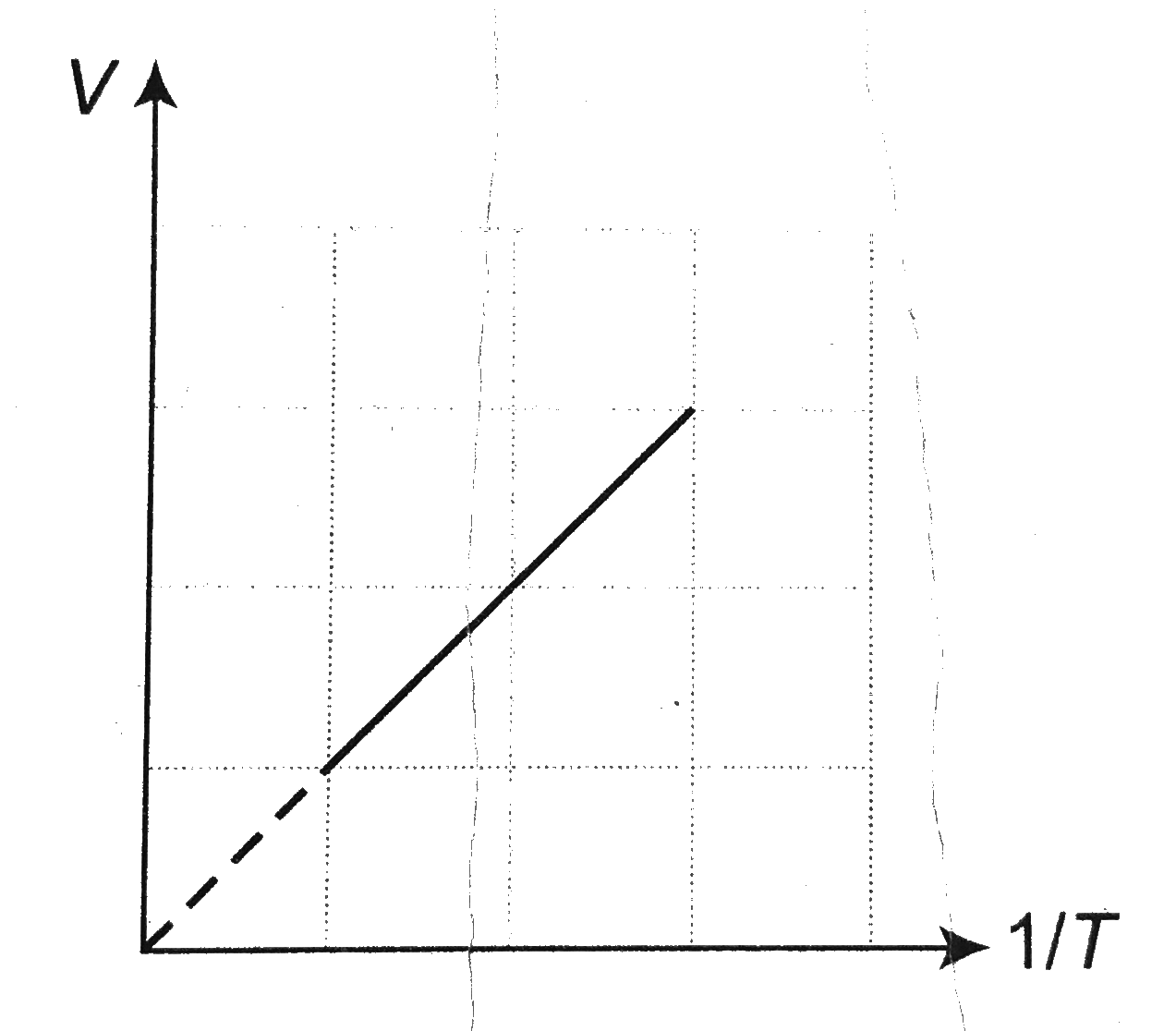
R is a gas constant.
A $\frac{3 R}{4}$
B $\frac{R}{2}$
C $\frac{R}{3}$
D $\frac{2 R}{3}$