A heated object (at time t = 0 and temperature T=T0) is taken out of the oven to cool and placed on a table near an open window. Write an depression for its temperature as a function of time T, where TS is the surrounding temperature
T=TS−(T0−TS)e−kt
T=TS+(T0+TS)e−kt
T=TS+(T0−TS)e−kt
T−TS−(T0−TS)e−kt
The correct Answer is:C
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEATEXERCISE - LECTURE SHEET (EXERCISE-I) Thermal Conduction (More than One correct answer Type Questions)4videos
TRANSMISSION OF HEAT
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEATEXERCISE - LECTURE SHEET (EXERCISE-I) Thermal Conduction (Linked Comprehension Type Questions) Passage - II3videos
TRANSMISSION OF HEAT
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEAT EXERCISE - QUESTION FOR DESCRIPTIVE ANSWER4videos
TRANSMISSION OF HEAT
- BOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - Practice Exercise45videos
UNITS AND MEASUREMENT
Similar Questions
A monatomic ideal gas is contained in a rigid container of volume V with walls of total inner surface area A, thickness x and thermal conductivity K. The gas is at an initial temperature t0 and pressure P0 . Find the pressure of the gas as a function of time if the temperature of the surrounding air is Ts . All temperature are in absolute scale.
View SolutionA rod of length l with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as k=a/T, where a is a constant. The ends of the rod are kept at temperatures T1andT2. Find the function T(x), where x is the distance from the end whose temperature is T1.
View Solution
Knowledge Check
- Question 1 - Select One
A heated object (at time t=0 and temperature T=T0) is taken out of the oven to cool and placed on a table near an open window Write an expression for its temperature as function of time T where Ts is the surrounding temperature .
AT=Ts−(T0+Ts)e−ktBT=Ts+(T0+Ts)e−ktCT=Ts+(T0−Ts)e−ktDT=Ts−(T0−Ts)e−kt - Question 2 - Select One
If a piece of metal is heated to temperature θ and the allowed to cool in a room which is at temperature θ0, the graph between the temperature T of the metal and time t will be closet to
A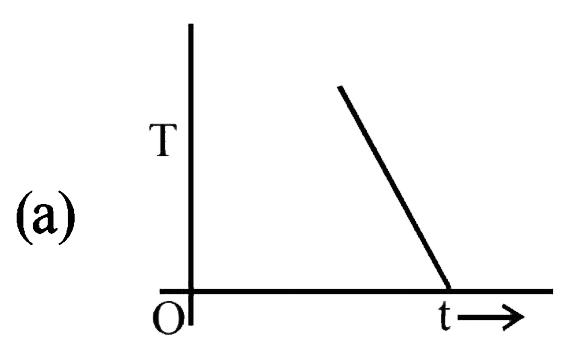 B
B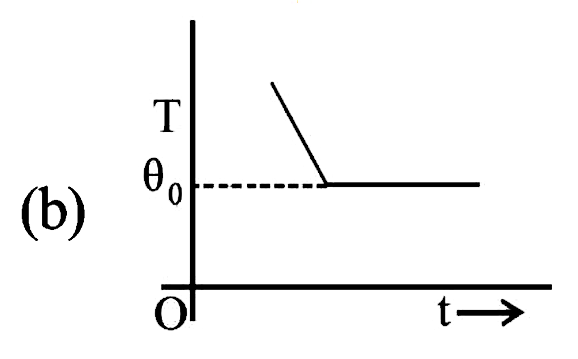 C
C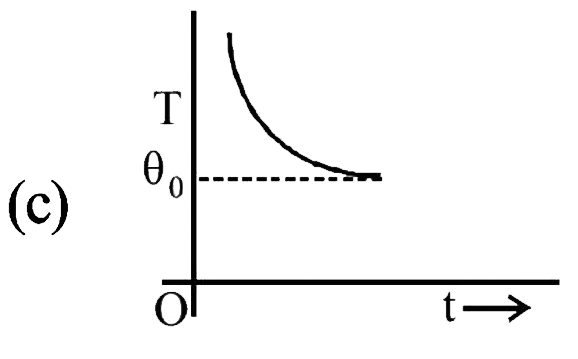 D
D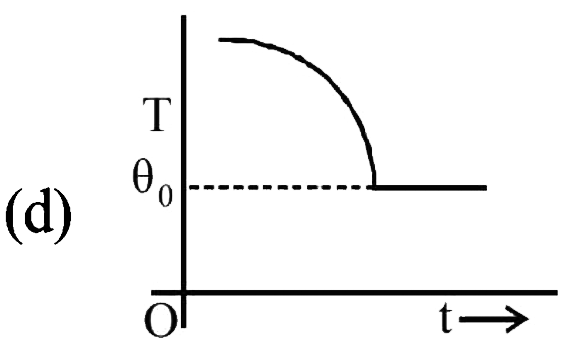
- Question 3 - Select One
A body at temperature θ0 having Newton's cooling constant K is placed in a surrounding having temperature T0 at time t = 0. The graph of temperature of the body as a function of time t is shown in the adjacent figure. A tangent on the curve is drawn at t = 0 as shown in the figure. Find the value of τ.
 AKB1KCK(Q0−T0)DData insufficient
AKB1KCK(Q0−T0)DData insufficient
A rod of length l with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as k=a/T, where a is a constant. The ends of the rod are kept at temperatures T1andT2. Find the function T(x), where x is the distance from the end whose temperature is T1.
View SolutionIf a piece of metal is heated to temperature θ and the allowed to cool in a room which is at temperature θ0, the graph between the temperature T of the metal and time t will be closet to
View SolutionA solid body A of mass m and specific heat capacity ‘s’ has temperature T1=400K. It is placed, at time t=0, in atmosphere having temperature T0=300K. It cools, following Newton’s law of cooling and its temperature was found to be T2=350Kat time t0. At time t0, the body A is connected to a large water bath maintained at atmospheric temperature T0, using a conducting rod of length L, cross section A and thermal conductivity k. The cross sectional area A of the connecting rod is small compared to the overall surface area of body A. Find the temperature of A at time t=2t0.
View SolutionA cylindrical conductor has a resistance R. When the conductor is at a temperature (T) above its surrounding temperature (T0), the ratio of thermal power dissipated by the conductor to its excess temperature (ΔT=T–T0) above surrounding is a known constant k. The conductor is connected to a cell of emf V. Initially, the conductor was at room temperature T0. Mass and specific heat capacity of the conductor are m and s respectively.
(i) find the time (t) dependence of the temperature (T) of the conductor after it is connected to the cell. Assume no change in resistance due to temperature.
(ii) find the temperature of the conductor after a long time.View SolutionOne end of a rod of length L and crosssectional area A is kept in a furnace at temperature T1. The other end of the rod is kept at a temperature T2. The thermal conductivity of the matrieal of the rod is K and emissivity of the rod is e. It is gives that T!=Ts+ΔT, where ΔT<<Ts,Ts is the temperature of the surroundings. If ΔT∝(T1−T2) find the proportional constant, consider that heat is lost only by rediation at the end where the temperature of the rod is T1.
 View Solution
View Solution

