Light of wavelength λ=500nm falls on two narrow slits placed a distance d=50×10−4cm apart, at an angle ϕ=30∘ relative to the slits as shown in figure. On the lower slit, a transparent slab of thickness 0.1 mm and refractive index 3/2 is placed. The interference pattern is observed on a screen at a distance D = 2m from the slits. Then calculate
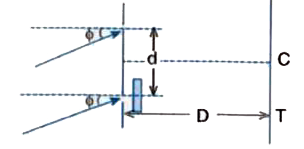
(a) position of the central maxima? (b) the order of minima closest to centre C of screen ? (c) How many fringes will pass over C, if we remove the transparent slab from the lower slit?
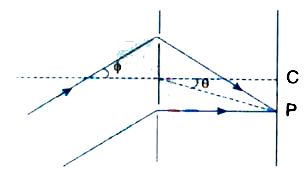
(a) Path difference,
Δx=dsinϕ+dsinθ−(μ−1)t
For central maxima,
Δx=0∴sinθ=(μ−1)td−sinϕ
sinθ=(3/2−1)(0.1)50×10−3−sin30∘,sinθ=12
∴θ=30∘
(b) At C,θ=0∘ , therefore
Δx=dsinθ−(μ−1)t=(50×10−3)(12)−(3/2−1)(0.1)
=0.025−0.05=−0.025mm
Substituting Δx=nλ, we get
n=Δxλ=−0.025500×10−6=−50
Hence at C, there will be 50th maxima. Therefore, closest to C, order of minima is -49 (c) Number of fringes shifted upwards on removing the slab
=(μ−1)tλ=(32−1)0.1500×10−6=100
Topper's Solved these Questions
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - I)
18 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)
20 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED ) Straight Objective Type Questions)
12 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
81 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)
15 Videos
Similar Questions
Light of wavelengthλ=500nm falls on two narrow slits placed a distance d = 50×10−4 cm
apart, at an angle ϕ=30∘ relative to the slits as shown in figure. On the lower slit a transparent slab of thickness 0.1 mm and refractive index 32 is placed. The interference pattern is observed at a distance D=2m from the slits. Then, calculate
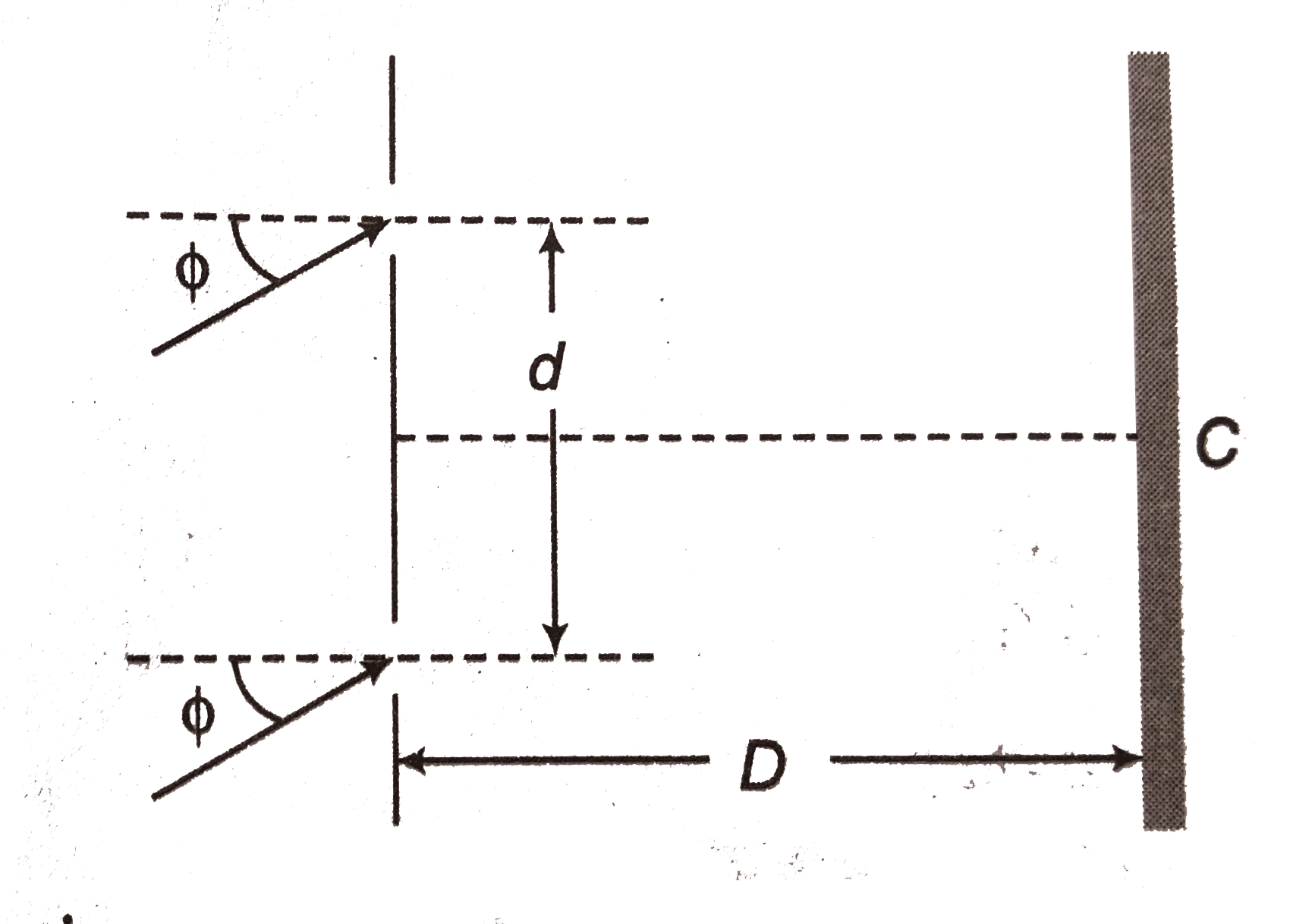
(a) position of the central maxima.
(b) the order of maxima at point C of screen .
(c)how many fringes will pass C, if we remove the transparent slab from the lower slit?
View SolutionLight of wavelengthλ=500nm falls on two narrow slits placed a distance d = 50×10−4 cm
apart, at an angle ϕ=30∘ relative to the slits as shown in figure. On the lower slit a transparent slab of thickness 0.1 mm and refractive index 32 is placed. The interference pattern is observed at a distance D=2m from the slits. Then, calculate
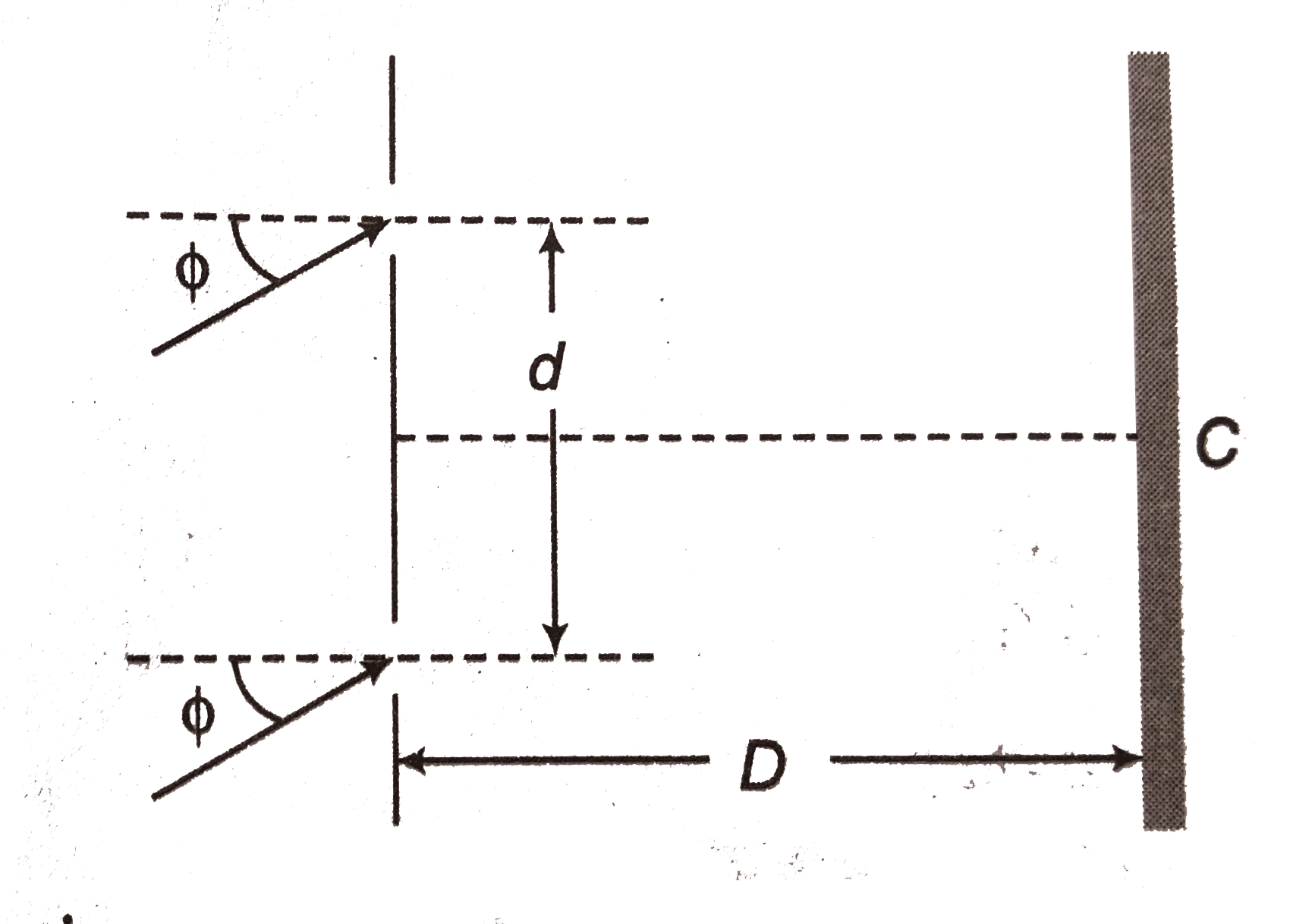
(a) position of the central maxima.
(b) the order of maxima at point C of screen .
(c)how many fringes will pass C, if we remove the transparent slab from the lower slit?
View Solution
Knowledge Check
In Young's double slit experiment the two slits are d distance apart. Interference pattern is observed on a screen at a distance D from the slits. A dark fringe is observed on the screen directly opposite to one of the slits. The wavelength of light is
AD22dBd22DCD2dDd2DA long narrow horizontal slit lies 1mm above a plane mirror. The interference pattern produced by the slit and its and its image is viewed on a screen ∑ distance 1m from the slit. The wavelength of light is 600nm. Then the distance of the first maxima above the mirror is equal to (d<<D) :
 A0.30mmB0.15mmC60mmD7.5mm
A0.30mmB0.15mmC60mmD7.5mm
Two slits in YDSE are placed 2 millimeter from each other. Interference pattern is observed on a screen placed 2m from the plane of slits. What is the fringe width for a light of wavelength 400 nm ?
View SolutionTwo slits in YDSE are placed 2 millimeter from each other. Interference pattern is observed on a screen placed 2 m from the plane of slits. What is the fringe width for a light of wavelength 400 nm?
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionA long narrow horizontal slit lies 1mm above a plane mirror. The interference pattern produced by the slit and its and its image is viewed on a screen ∑ distance 1m from the slit. The wavelength of light is 600nm. Then the distance of the first maxima above the mirror is equal to (d<<D) :

View SolutionIn Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
View Solution
